As the speed of a particle increases, its rest mass
increases
decreases
remains the same
changes
The correct Answer is:C
Topper's Solved these Questions
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMACHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Objective 2
6 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMACHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Exercises
27 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMACHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - worked out example
8 Videos
- THE NUCLEOUSBOOK - HC VERMACHAPTER - THE NUCLEOUSEXERCISE - Exercises
53 Videos
- THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTBOOK - HC VERMACHAPTER - THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTEXERCISE - Exercises
24 Videos
Similar Questions
Knowledge Check
The velocity at which the mass of a particle becomes twice its rest mass, will be -
A2c3Bc2Cc√32D3c4The velocity at which the mass of a particle becomes twice of its rest mass, will be -
A2c3Bc2Cc√32D3c4The speed of a particle moving in a circle is increasing. The dot product of its acceleration and velocity is
AnegativeBzeroCpositiveDmay be positive or negative
A particle of mass m has half the kinetic energy of another particle of mass m2. If the speed of the heavier particle is increased by 2ms−1 its new kinetic energy becomes equal to t he original kinetic energy of the lighter particle. The ratio of the orighinal speeds of the lighter and heavier particle is
View SolutionA particle starts moving from rest on a straight line. Its acceleration a verses time t is shown in the figure. The speed of the particle is maximum at the instant
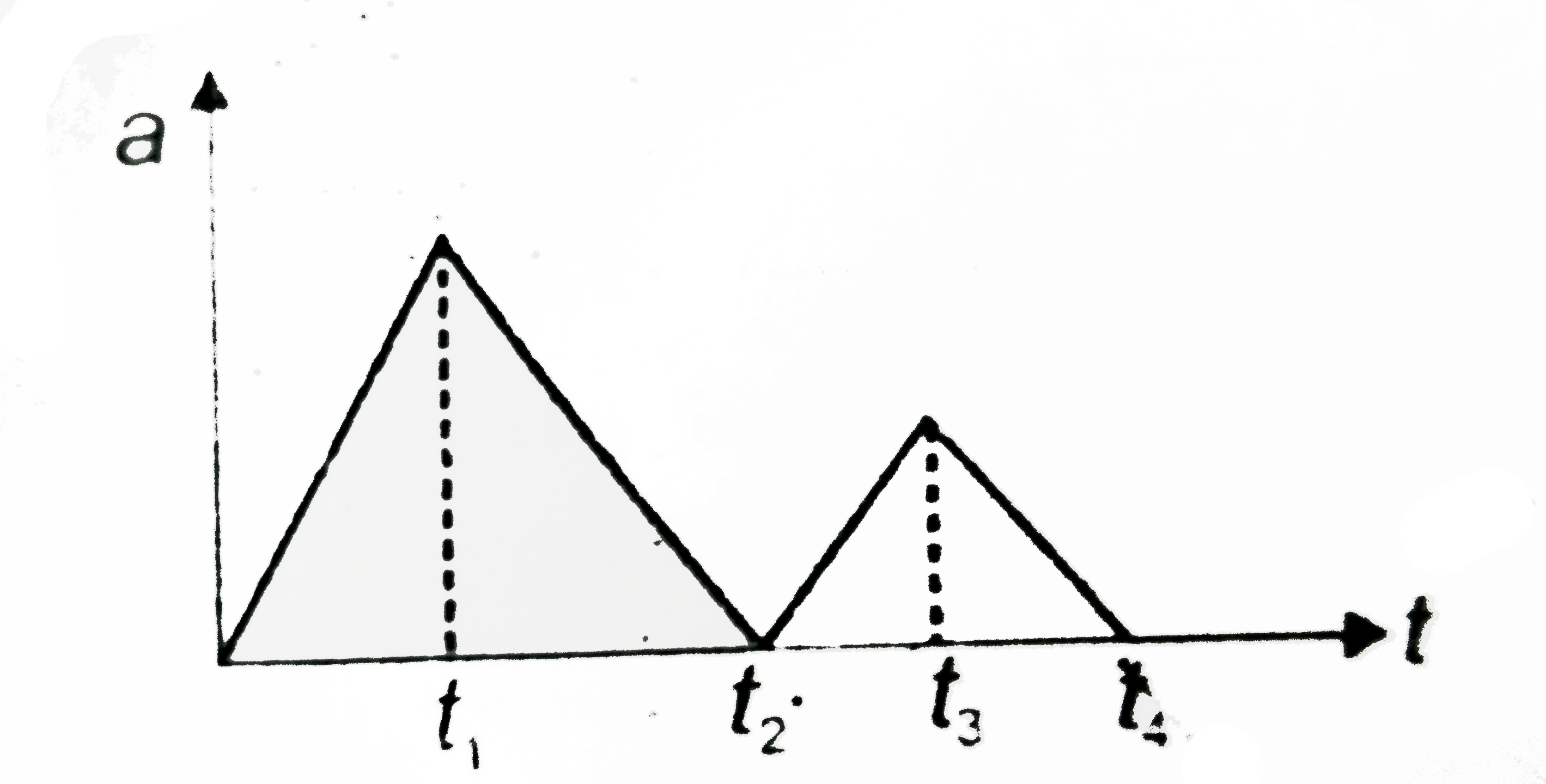
View SolutionA particle starts moving from rest on a straight line. Its acceleration a verses time t is shown in the figure. The speed of the particle is maximum at the instant

View SolutionFind the speed at which the mass of an electron is double of its rest mass.
View SolutionA particle of mass m has half the kinetic energy of another particle of mass m2. If the speed of the heavier particle is increased by 2ms−1 its new kinetic energy becomes equal to t he original kinetic energy of the lighter particle. The ratio of the orighinal speeds of the lighter and heavier particle is
View Solution
