Assertion: Vector addition is commutative.
Reason: (→A+→B)≠(→B+→A)
If both assetion and reason are true and the reason is the correct explanation of the assertion.
If both assertion and reason are true but reason is not the correct explanation of the assertion
If assertion is true but reason is false
If the assertion and reason both are false.
The correct Answer is:C
Step by step video, text & image solution for Assertion: Vector addition is commutative. Reason: (vecA+vecB)!=(vecB+vecA) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Consider three vectors →A,→Band→C as shown in fig. perform graphically the following vector additions and subtractions (a) →A+→B
(b). →A+→B+→C
(c). →A−→B
(d). →A+→B−→C
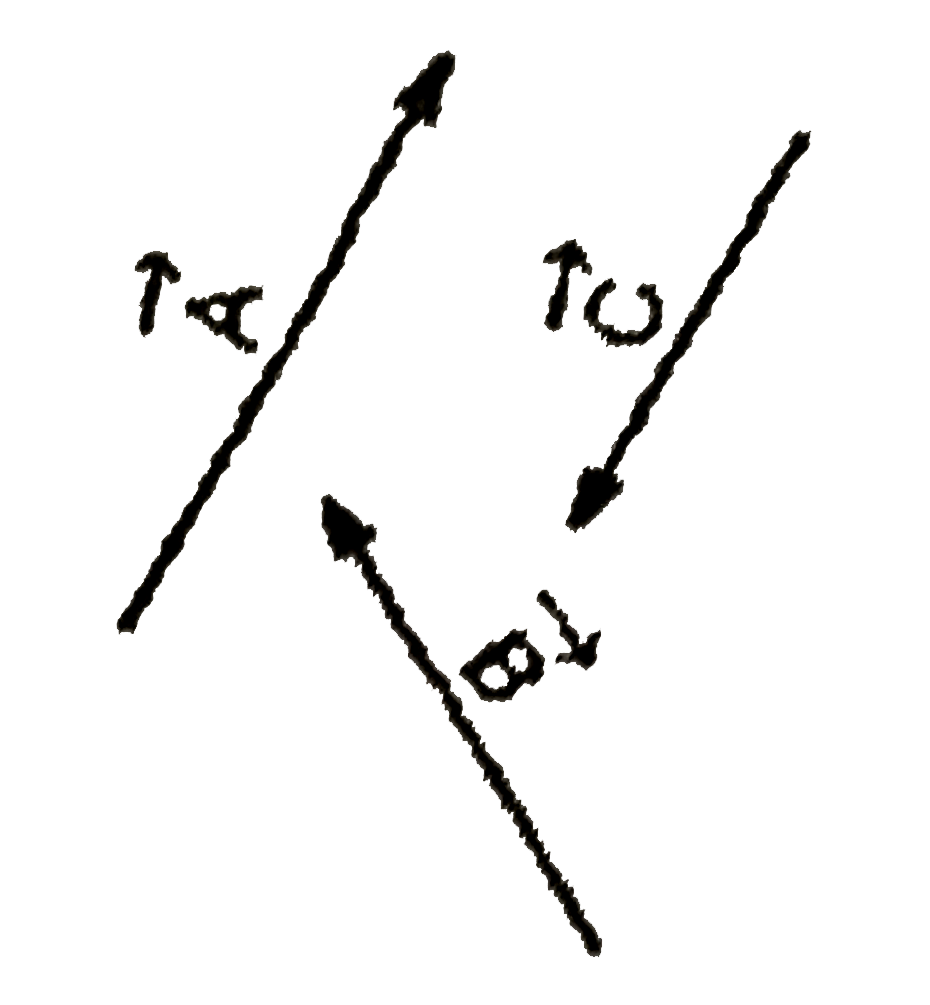
View Solution(a) Show that vector adition is commutative but vector subtraction is non-commutative .
(b) For vectors →A=3ˆi−4ˆj+5ˆkand→B=ˆi−ˆj−ˆk, calculate the value of (→A+→B)×(→A−→B)
View Solution
Knowledge Check
Assertion: Vector addition of two vectors vedA and →B is commutative.
Reason: →A+→B=→B+→AAIf both assetion and reason are true and the reason is the correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of the assertionCIf assertion is true but reason is falseDIf the assertion and reason both are false.Vectors →Aand→B satisfying the vector equation →A+→B=→a,→A×→B=→band→A.→a=1. Vectors and →b are given vectosrs, are
A→A=(→a×→b)−→aa2B→B=(→b×→a)+→a(a2−1)a2C→A=(→a×→b)+→aa2D→B=(→b×→a)−→a(a2−1)a2Vectors →Aand→B satisfying the vector equation →A+→B=→a,→A×→B=→band→A.→a=1. Vectors and →b are given vectosrs, are
A→A=(→a×→b)−→aa2B→B=(→b×→a)+→a(a2−1)a2C→A=(→a×→b)+→aa2D→B=(→b×→a)−→a(a2−1)a2
Assertion : A vector →A can be resolved into component along with given vectors →aand→b lying in the same plane.
Reason : →A=λ→a+μ→b where λ and μ are real numbers.
View SolutionIf →b and →c are any two orthogonal unit vectors and →a is any vector, then (→a.→b)→b+(→a.→c)→c+→a.(→b×→c)∣∣→b×→c∣∣2(→b×→c)=
View SolutionFor vectors →A and →B, (→A+→B).(→A×→B) will be :
View SolutionAssertion: If three vectors →A,→B and →C satisfy the relation →A.→B=0&→A.veC=0 then the vector →A may be parallel to →B×→C.
View SolutionIf two non-parallel vectors →A and →B are equal in magntiude, them vectors (→A−→B) and (→A+→B) will be :
View Solution
