Assuming that earth and mars move in circular orbits around the sun, with the martian orbit being 1.52 times the orbital radius of the earth. The length of the martian year is days is
(1.52)2/3×365
(1.52)3/2×365
(1.52)2×365
(1.52)3×365
The correct Answer is:B
To find the length of the Martian year, we can use Kepler's Third Law of planetary motion, which states that the square of the orbital period (T) of a planet is directly proportional to the cube of the semi-major axis (R) of its orbit. The law can be expressed mathematically as:
T2∝R3
1. Identify the relationship between the orbital radii:
Given that the orbital radius of Mars (Rm) is 1.52 times the orbital radius of Earth (Re), we can write:
Rm=1.52×Re
2. Apply Kepler's Third Law:
According to Kepler's Third Law, we can write the equations for the time periods of Mars (Tm) and Earth (Te):
Tm2∝Rm3(1)
Te2∝Re3(2)
3. Set up the ratio of the time periods:
Dividing equation (1) by equation (2):
Tm2Te2=Rm3Re3
4. Substitute the relationship between Rm and Re:
Substitute Rm=1.52×Re into the equation:
Tm2Te2=(1.52×Re)3Re3
5. Simplify the equation:
This simplifies to:
Tm2Te2=1.523
6. Calculate 1.523:
Calculate 1.523:
1.523≈3.51
7. Relate the time periods:
Now we can express the relationship between the time periods:
Tm2=3.51×Te2
8. Substitute Te:
Since the time period of Earth (Te) is 1 year, which is approximately 365 days:
Tm2=3.51×(365)2
9. Calculate Tm:
Now, calculate Tm:
Tm=√3.51×(365)2
Tm≈√3.51×365≈1.87×365≈683.55 days
10. Final Answer:
Therefore, the length of the Martian year is approximately:
Tm≈687 days
Given that the orbital radius of Mars (Rm) is 1.52 times the orbital radius of Earth (Re), we can write:
Rm=1.52×Re
According to Kepler's Third Law, we can write the equations for the time periods of Mars (Tm) and Earth (Te):
Tm2∝Rm3(1)
Te2∝Re3(2)
Dividing equation (1) by equation (2):
Tm2Te2=Rm3Re3
Substitute Rm=1.52×Re into the equation:
Tm2Te2=(1.52×Re)3Re3
This simplifies to:
Tm2Te2=1.523
Calculate 1.523:
1.523≈3.51
Now we can express the relationship between the time periods:
Tm2=3.51×Te2
Since the time period of Earth (Te) is 1 year, which is approximately 365 days:
Tm2=3.51×(365)2
Now, calculate Tm:
Tm=√3.51×(365)2
Tm≈√3.51×365≈1.87×365≈683.55 days
Therefore, the length of the Martian year is approximately:
Tm≈687 days
Topper's Solved these Questions
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Universal Law Of Gravitations
14 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - The Gravitational Constant
2 Videos
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
Similar Questions
The planet Mars has two moons. Phobos and Delmos (i) phobos has period 7 hours, 39 minutes and an orbital radius of 9.4×103km. Calculate the mass of Mars. (ii) Assume that Earth and mars move in a circular orbit around the sun, with the martian orbit being 1.52 times the orbital radius of the Earth. What is the length of the martian year in days? (G=6.67×10−11Nm2kg−2)
View SolutionIn equilibrium, Mars emits as much radiation as it absorbs. If mars orbits the sun with an orbital radius that is 1.5 times the orbital radius of the earth about the sun, what is the approximate atmospheric temperature of mars? Assume the atmospheric temperature of earth to be 253 K -
View Solution
Knowledge Check
A planet moving in a circular orbit around the sun at a distance, 4times theaverage distance of the earth from the sun will complete one revolution in
A8 yearsB4 yearsC16 yearsD1/4 yearsA satellite is moving in an orbit around the earth due to
ABurning of fuelBgravitational attraction between sun and earthCejection of gases from the exhaust of the satelliteDgravitational attraction between earth and the satelliteEarth completes one orbit around the sun is
A365 days 5 hrs 48 min and 46 secB365 days 1 hrs 22 min and 3 secC364 days 22 hrs 58 min and 39 secD364 days 23 hrs 48 min and 51 sec
Assuming that the moon completes one revolution in a circular orbit around the earth in 27.3 days, calculate the acceleration of the moon towards the earth. The radius of the circular orbit can be taken as 3.85×105km.
View SolutionA satellite of Sun is in a circular orbit around the Sun, midway between the Suna and earth. Find the period of this satellite.
View SolutionIn adjoining figure earth goes around the sun in eliptical orbit on which point the orbital speed is maximum :
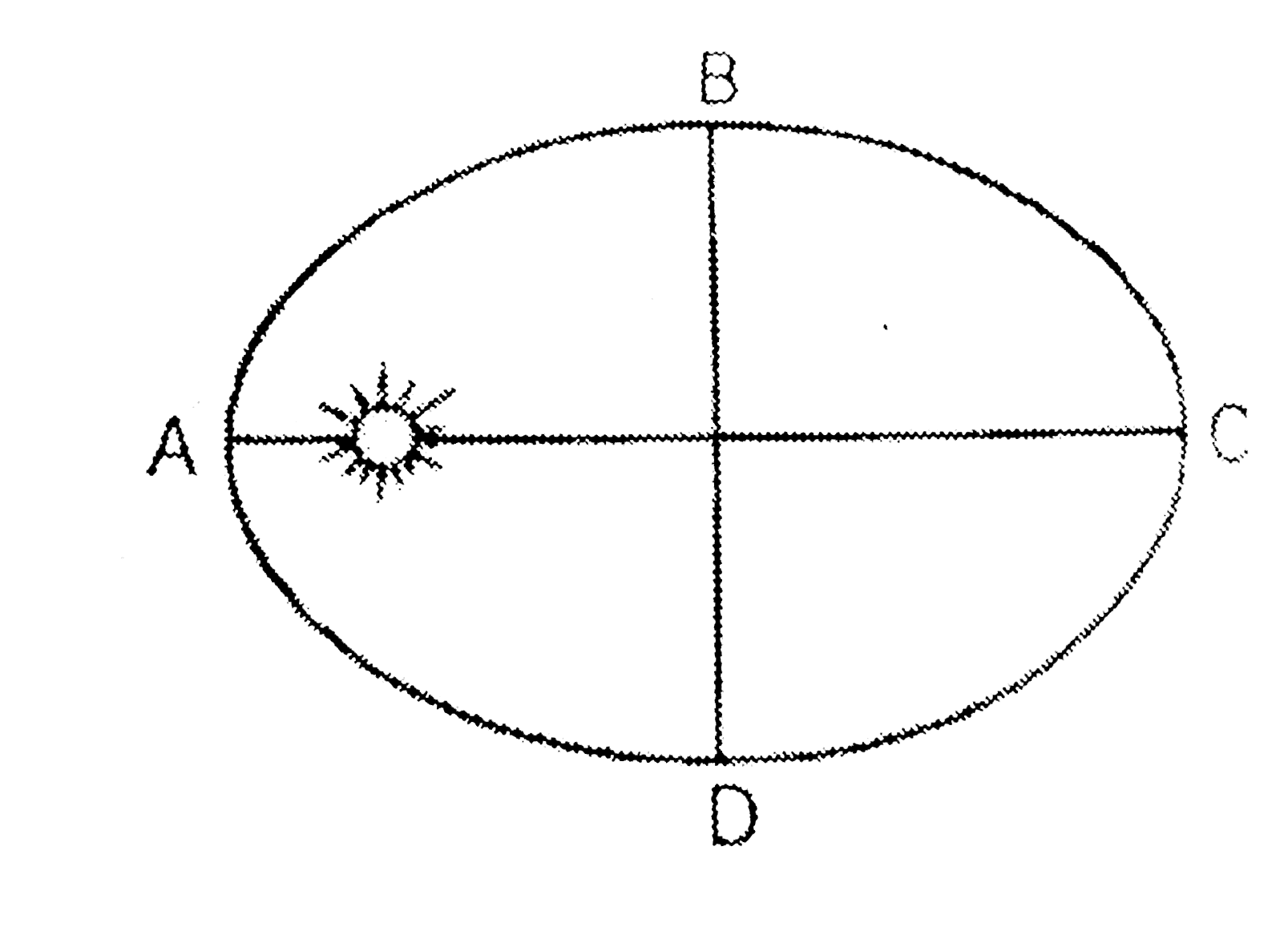
View SolutionAssume that Earth is in circular orbit around the Sun with kinetic energy K and potential energy U, taken to be zero for infinite separation. Then, the relationship between K and U:
View SolutionA planet is rotating around the sun with the orbital radius three times than that of earth. The time period of this planet will be (For earth, time period is 365 days )
View Solution
