At t=0 aparticle of mass m start moving from rest due to a force →F=F0sin(ωt)ˆi :
Particle perform SHM about its initial position of rest
Particle perform SHM with initial position as extreme position with angular frequency ω
At any instant, distance moved by the particle equals its displacement, from the initial position
initial velocity of particle increases with time but after time t=2π/ω it becomes constant
The correct Answer is:C
If a particle S.H.M. If comes to rest at exterm position but at external position , acceleration of the particle is maximum position .Therefore resultantforce on the particle will also the maximum pasition But force on the given by F=Fs on as and at t=0 the particle was at when it is at rest Therefore this particle cannot S.H.M. Therefore (a) and (b) are wrong
In the particle case the force is zero at the initial moment and always act along x−aξs velocity the force start position x− dirfectionThereforepartic≤s⋆t→ae≤rationalongx-dirfectionandcont∈utespositionup→the∈stantgivenbyomega t = piafterpartic≤momentform∵¬ativehenceposition⋆t→rηrdbuttheimpe–oftheforceom t = 0→t = pi//omega tisνmerically→itsimpluseomt = (pi)/(omega)→t = (2pi)/(omega)hencethepartic≤willcomes→restpartic≤contr∈uiously∈creaseandandthenitdecreasepartic≤move∵¬ativehencethepartic≤contitues→movealongpositivedirectionofax-aξshebnceitsdisplacementatany∈stantwillbeequal→thedistancemovedbythepartic≤omthe∈itialposition<br>Henceciscorrect<br>Aftertimet = (2pi)/(omega)force∵positionaga∈itmeanthepartic≤⋆t→ae≤rationaga∈alongpositiondirectionofax- axis .Therefore velocity never becomes correct
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Velocity , Acceleration And Energy Of Simple Harmonic Motion40videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Spring Particle System24videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test30videos
NEWTONS LAWS OF MOTION
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
Similar Questions
At the moment t=0 a particle of mass m starts moving due to a force F=F0sinωt, where F0 and ω are constants. Find the distance covered by the particle as a function of t. Draw the approximate plot of this function.
View SolutionAt the moment t=0 a particle of mass m starts moving due to a force F=F0cosωt, where F0 and ω are constants. How long will it be moving until it stops for the first time? What distance will it traverse during that time? What is the maximum velocity of the particle over this distance?
View Solution
Knowledge Check
- Question 1 - Select One or More
A particle of mass m starts moving at t=0 due to force F=F0sinωt where F0 and ω are constant Then correct statement is//are .
Ait will stop first time at πωBIt will travel distance S=F0mω2 during this time .CDuring this distance maximum velocity of particle is vmax=F0mω .Dit will stop for first time at 2π/ω - Question 2 - Select One
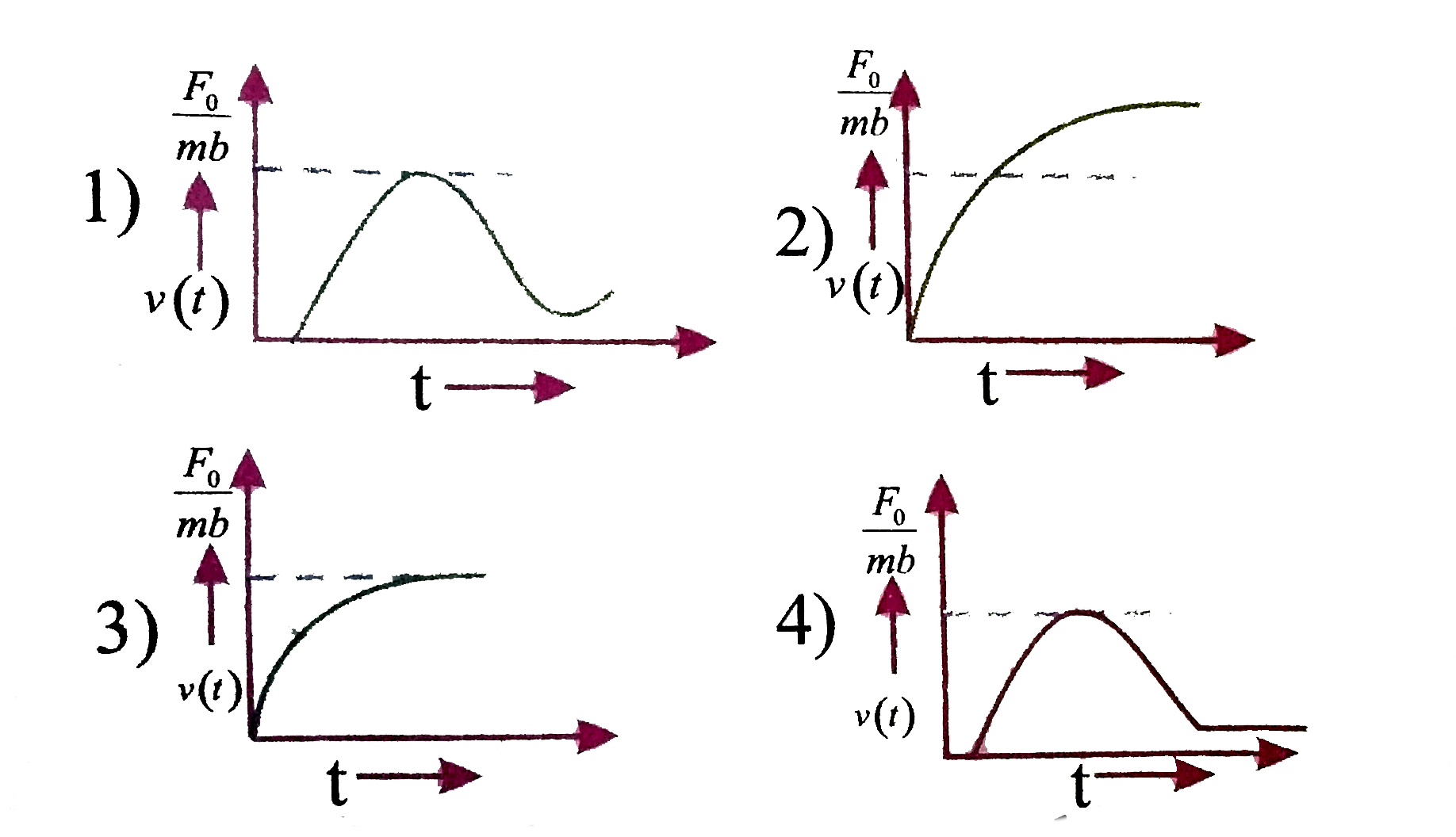
A particle of mass m is at rest the origin at time t=0 . It is subjected to a force F(t)=F0e−bt in the x - direction. Its speed v(t) is depicted by which of the following curves ?
A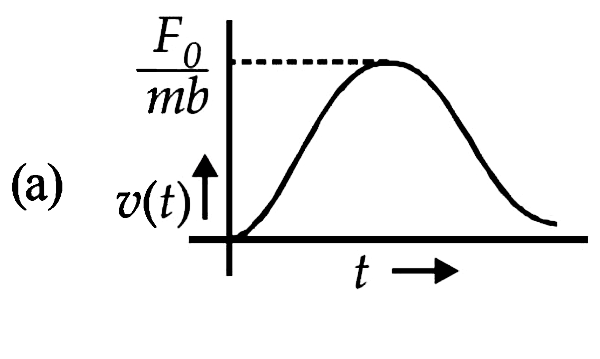 B
B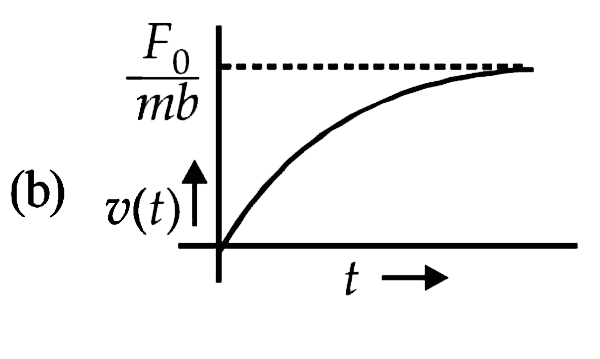 C
C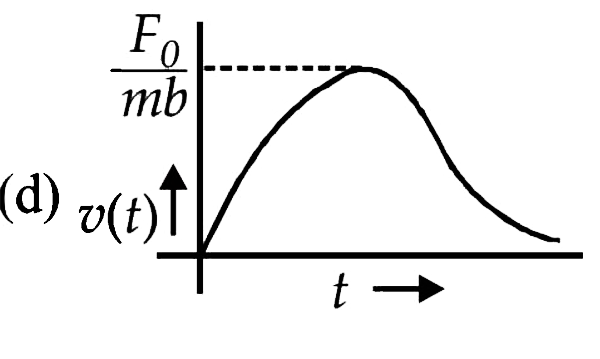 D
D
- Question 3 - Select One
A particle of mass m is subjected to a force →F=F0[cos(t)ˆi+sin(1)ˆj]. If initially (t=0) the particle was at rest, the kinetic energy of the particle as a function of time is given by:
AF20m(1−cos(2t))BF20m(1−cost)CF20msin(t)DF20mt
A particle of rest mass m0 starts moving at a moment t=0 due to a constant force F. Find the time dependence of the particle's velocity and of the distance covered.
View SolutionA particle of mass m is at rest at the origin at time t=0 It is subjected to a force F(t)=F0e−bt in the X-direction. Its speed V(t) is depicted by which of the following curves
 View Solution
View SolutionA particle of mass 10−3 kg and charge 1.0 c is initially at rest At time t=0 the particle comes under the influence of an electric field ˆE(t)=E0sinωtˆi where E0=1.0NC−1 and ω=103rads−1 consider the effect of only the electrical force on the particle .Then the maximum speed in m s−1 attained by the particle at subsequent times is ______________
View SolutionThe displacement vector of a particle of mass m is given by r (t) = ˆiAcosωt+ˆjBsinωt.
(a) Show that the trajectory is an ellipse.
(b) Show that F = −mω2r.View SolutionA particle of mass m is at rest at the origin at time t=0. It is subjected to a force F(t)=F0e−bt in the X - direction. Its speed V(t) is depicted by which of the following curves.
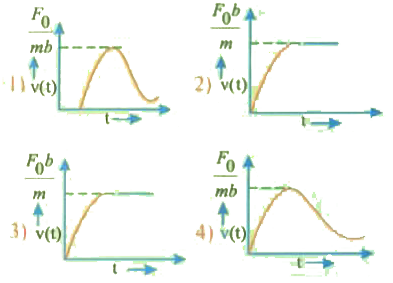 View Solution
View Solution

