Brake or turn? Figure depicts an overhead view of a car's path as the car travels toward a wall. Assume that the driver begins to brake the car when the distance to the wall is d=107 m, and take the car's mass a m=1400 kg, its initial speed as v0=35 m/s and the coefficient of static friction as μs=0.50. Assume that car's weight is distributed evenly on the four wheels, even during braking (a) What magnitude of static friction is needed (between tires and road) to stop the car just as it reaches the wall? (b) What is the maximum possible static friction fs,max/ (c) If the coefficient of kinetic frictic between the (sliding) tires and the road is μk=0.40, at what speed will the car hit the wall? To avoid the crash, a driver could elect to turn the car so that it just barely misses the wall, as shown in the figure. (d) What magnitude of frictional force would be required to keep the car in a circular path of radius d and at the given speed v0, so that the car moves is a quarter circle and then parallel to the wall? (e) Is the required force less than fs.max so that a circular path is possible?
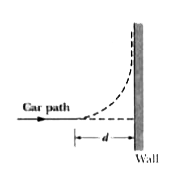
The correct Answer is:(a) 8.0×103N; (b) 6.9×10N; (c) 20m/s (d) 1.6×10N (e) NO circular path is possible
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(SINGLE CORRECT CHOICE TYPE)40videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(MORE THAN ONE CORRECT CHOICE TYPE)6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
Similar Questions
A car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tyre and the road is μs=2.0, find the maximum speed the car can take without skidding.
View SolutionA car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tire and the road is μs=2.0, find the maximum speed the car can take without skidding.
View Solution
Knowledge Check
- Question 1 - Select One
The brakes of a car moving at 20 m/s along a horizontal road are suddenly applied and it comes to rest after travelling some distance if the coefficient of friction between the tyres and the road is 0.90 and it is assumed that all four tyres behave identically, the shortest distance the car would travel before coming to a stop is
A2.27 mB11.35 mC22.7 mD4.54 m - Question 2 - Select One
A 1580 kg car is traveling with a speed of 15.0 m/s. What is the magnitude of the horizontal net force that is required to bring the car to a halt in a distance of 50.0 m ?
A1030 NB2490 NC3560 ND4010 N - Question 3 - Select One
When the driver of a fast moving car suddenly applies brakes, the passengers in the car
Afall backwardBfall forwardCare not affectedDnone of these
A car is travelling at 20 m/s along a road. A child runs out into the road 50 m ahead and the car driver steps on the brake pedal. What must the car's deceleration be if the car is to stop just before it reaches the child ?
View SolutionA car of mass m is moving on a level circular track of radius R if μs represents the static friction between the road and tyres of the car, the maximum speed of the car in circular motion is given by.
View SolutionA car of mass m is moving on a level circular track of radius R. If μs represents the static friction between the road and tyres of the car, the maximum speed of the car in circular motion is given by
View SolutionA car of mass m is moving on a level circular track of radius R, if μs represents the static friction between the road and tyres of the car, the maximum speed of the car in circular motion is given by.
View SolutionA car is moving on a horizontal circular tracck of radius 0⋅2 km with a constant speed. If coefficient of friction between tyres of car and road is 0⋅45, then speed of a car may be [ Take g = 10ms2]
View Solution

