Calculate the increase in mass when a body of rest mass 1 kg is lifted up through 1 m near the earth's surface.
To calculate the increase in mass when a body of rest mass 1 kg is lifted up through 1 meter near the Earth's surface, we can follow these steps:
Step 1: Understand the Concept of Mass-Energy Equivalence
According to the theory of relativity, mass can be converted into energy and vice versa. The relationship is given by the equation:
E=mc2
where E is energy, m is mass, and c is the speed of light in vacuum (c≈3.0×108m/s).
Step 2: Calculate the Increase in Potential Energy
When the body is lifted, its potential energy increases. The formula for gravitational potential energy is:
PE=mgh
where:
- m=1kg (rest mass of the body),
- g=9.8m/s2 (acceleration due to gravity),
- h=1m (height lifted).
Substituting the values:
PE=1kg×9.8m/s2×1m=9.8J
Step 3: Relate the Increase in Energy to the Increase in Mass
Using the mass-energy equivalence principle, the increase in mass (Δm) can be calculated from the increase in energy (ΔE):
ΔE=Δm⋅c2
Rearranging this gives:
Δm=ΔEc2
Step 4: Substitute the Values
We already calculated the increase in energy (ΔE=9.8J). Now, substituting this and the value of c:
Δm=9.8J(3.0×108m/s)2
Calculating c2:
c2=(3.0×108)2=9.0×1016m2/s2
Now substituting this back:
Δm=9.89.0×1016
Step 5: Perform the Calculation
Calculating the above expression:
Δm≈1.11×10−18kg
Final Answer
The increase in mass when a body of rest mass 1 kg is lifted up through 1 m near the Earth's surface is approximately:
Δm≈1.11×10−18kg
---
According to the theory of relativity, mass can be converted into energy and vice versa. The relationship is given by the equation:
E=mc2
where E is energy, m is mass, and c is the speed of light in vacuum (c≈3.0×108m/s).
When the body is lifted, its potential energy increases. The formula for gravitational potential energy is:
PE=mgh
where:
- m=1kg (rest mass of the body),
- g=9.8m/s2 (acceleration due to gravity),
- h=1m (height lifted).
PE=1kg×9.8m/s2×1m=9.8J
Using the mass-energy equivalence principle, the increase in mass (Δm) can be calculated from the increase in energy (ΔE):
ΔE=Δm⋅c2
Rearranging this gives:
Δm=ΔEc2
We already calculated the increase in energy (ΔE=9.8J). Now, substituting this and the value of c:
Δm=9.8J(3.0×108m/s)2
c2=(3.0×108)2=9.0×1016m2/s2
Δm=9.89.0×1016
Calculating the above expression:
Δm≈1.11×10−18kg
The increase in mass when a body of rest mass 1 kg is lifted up through 1 m near the Earth's surface is approximately:
Δm≈1.11×10−18kg
Topper's Solved these Questions
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Objective
2 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Objective 2
1 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Short answer
2 Videos
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Short answer
12 Videos
- THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTBOOK - HC VERMA ENGLISHCHAPTER - THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTEXERCISE - Short Question
9 Videos
Similar Questions
Knowledge Check
The change in the gravitational potential energy when a body of a mass m is raised to a height nR above the surface of the earth is (here R is the radius of the earth)
AmgREn(n−1)BmgRECmgREn(n+1)DmgREn
Calculate the kinetic energy of a body of mass 0.1 kg and momentum 20 kg ms−1
View SolutionCalculate the height through which a body of mass 0.5 kg is lifted if the energy spent in doing so is 10j . Take g = 10 ms−2
View SolutionWhat is the work done in shifting a body of mass m=5okg slowly from the earth's surface to infinity?
View SolutionFind the change in the gravitational potential energy when a body of mass m is raised to a height nR above the surface of the earth. (Here, R is the radius of the earth)
View SolutionCalculate the Force (F) required to cause the block of mass m1=20kg just to slide under the block of mass m2=10kg [ coefficient of friction μ=0⋅25 for all surfaces ]
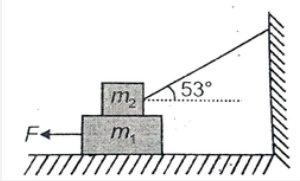
View SolutionA boy is of mass 40 kg. If the pull of gravity on 1 kg on the surface of earth is 9.8 N and at the surface of moon is 1.6 N, find the weight of boy at the earth's surface
View SolutionWhat is the gravitational potential energy of the earth and a satellite of mass m=100kg orbiting close to the earth's surface ?
View Solution
