Can you see how the two wires having different conductivities connected in a circuit will have different values of the electric field inside them? What will be the sign of excess charge built up on the interface between two cylindrical conductors as shown in the figure? 
The correct Answer is:ECu<ENi+ positive
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Problems36videos
CURRENT AND RESISTANCE
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Single Corret Choice Type)39videos
CURRENT AND RESISTANCE
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
ELASTICITY
Similar Questions
Two spheres of same metal have radii a and b . They have been connected to a conducting wire. Find the ratio of the electric field intensity upon them.
View SolutionA power circuit uses a cable having three different wires.
Between which of the two wires should the heating element of an electric geyser be connected ?View Solution
Knowledge Check
- Question 1 - Select One
Two spheres of same metal have radii a and b . They have been connected to a conducting wire. Find the ratio of the electric field intensity upon them.
Aa/bBb/aCb2/aDb2/a2 - Question 2 - Select One
How many different capacitances can you produce using three capacitors if you have all three connected in the circuit each time?
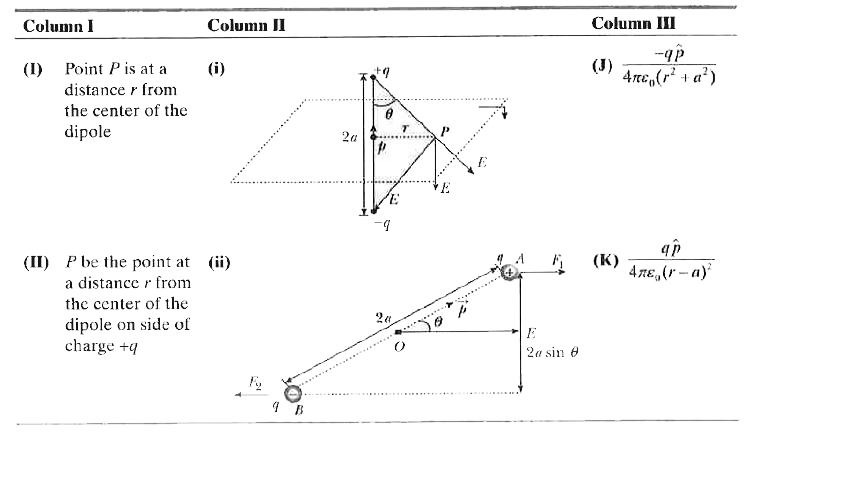
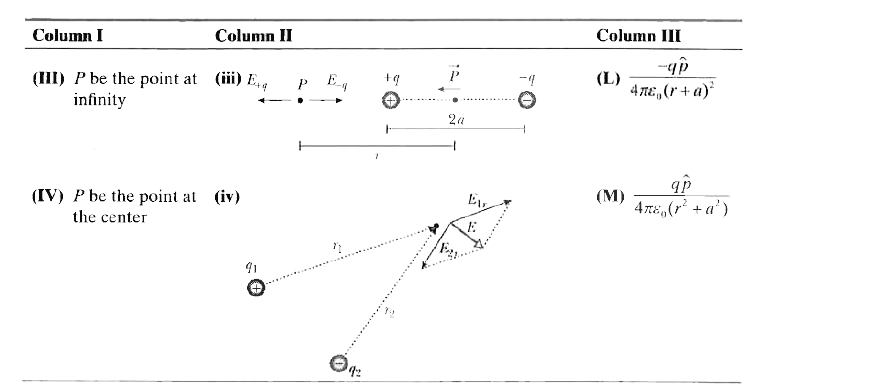
A5B6C7D8 - Question 3 - Select One
An electric dipole is the combination of two equal and opposite charges q and -q, respectively, separated by distance 2a. There are different points where we can find electric field due to the electric dipole. In the given table, column 1 shows different positions of point where we have to find electric field due to dipole, column 2 shows the figure of dipole with different positions of point where we have to find the electric field and column 3 shows the value or final formula of the electric field of different positions of the point.
When point P lies on the equatorial plan of the electric dipole, electric field due to -q is ?
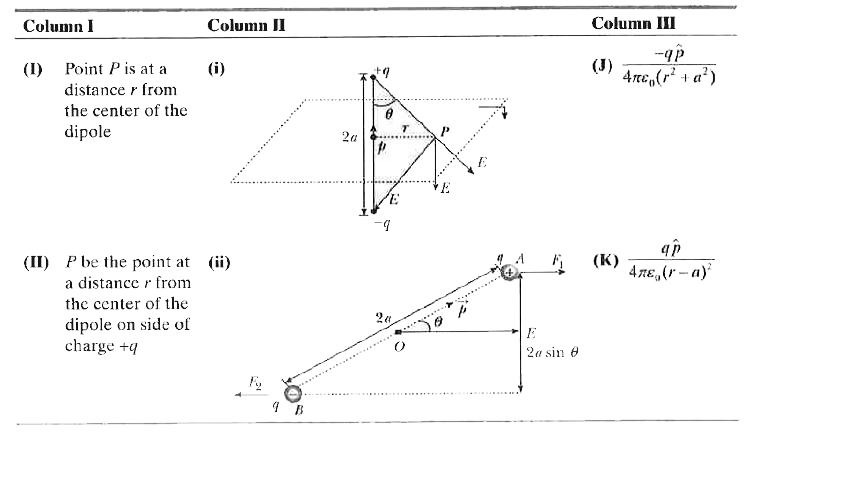
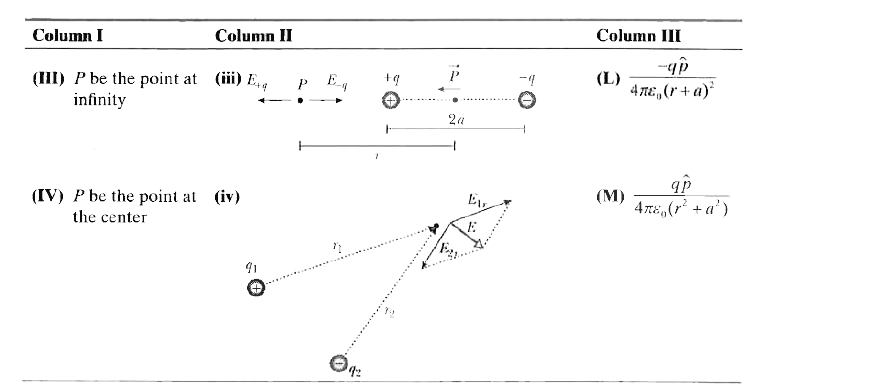A(I) (i) (J)B(I) (i) (M)C(III)(iii) (J)D(I) (i) (K)
Two thin infinite parallel plates have uniform charge densities +σ and −σ. The electric field in the space between them is
View SolutionAn electric dipole is the combination of two equal and opposite charges q and -q, respectively, separated by distance 2a. There are different points where we can find electric field due to the electric dipole. In the given table, column 1 shows different positions of point where we have to find electric field due to dipole, column 2 shows the figure of dipole with different positions of point where we have to find the electric field and column 3 shows the value or final formula of the electric field of different positions of the point.
when point P lies on the equatorial plane of the electric dipole, electric field due to +q is ?
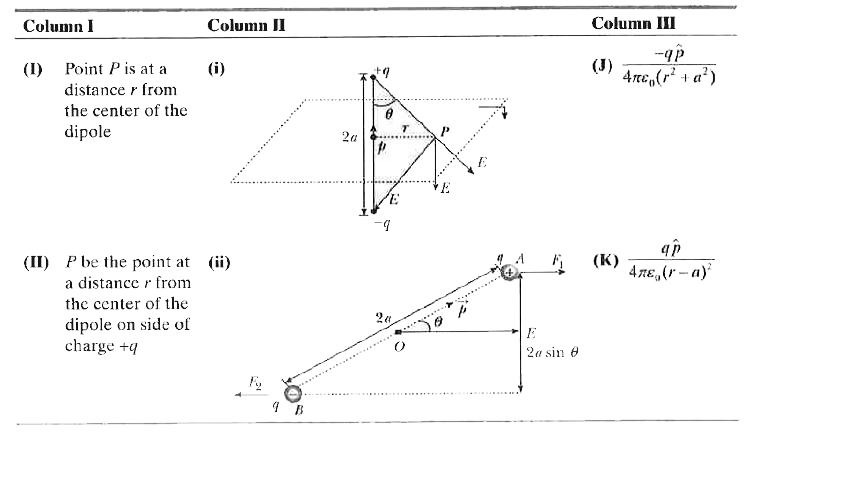
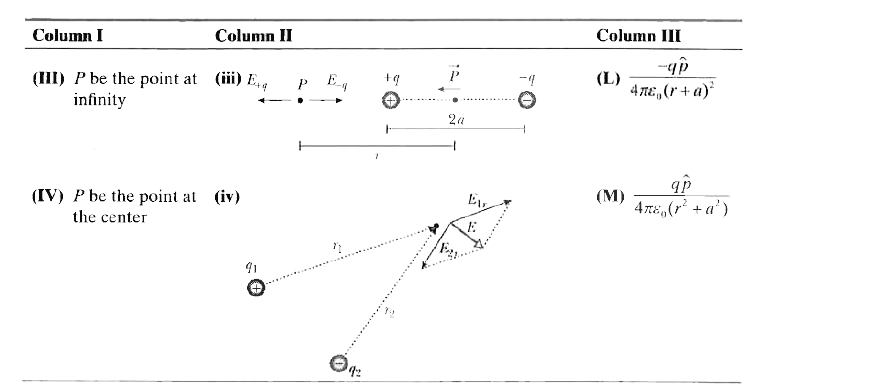
View SolutionAn electric dipole is the combination of two equal and opposite charges q and -q, respectively, separated by distance 2a. There are different points where we can find electric field due to the electric dipole. In the given table, column 1 shows different positions of point where we have to find electric field due to dipole, column 2 shows the figure of dipole with different positions of point where we have to find the electric field and column 3 shows the value or final formula of the electric field of different positions of the point.
When point P lies on the axis of the electric dipole, electric field due to -q is ?
View SolutionTwo large parallel plane sheets have uniform charge densities + σand−σ. Determine the electric field (i) between the sheets, and (ii) outside the sheets.
View SolutionAssertion (A): If two spherical conductors of different radii have the same surface charge densities, then their electric field intensities will be equal.
Reason (R ): Surface charge density =Total chargeareaView Solution