Conisder an electric field $\to E = E_{0} ˆ x$ where $E_{0}$ is a constant .
The flux through the shaded area (as shown in the figure) due to this field is

A

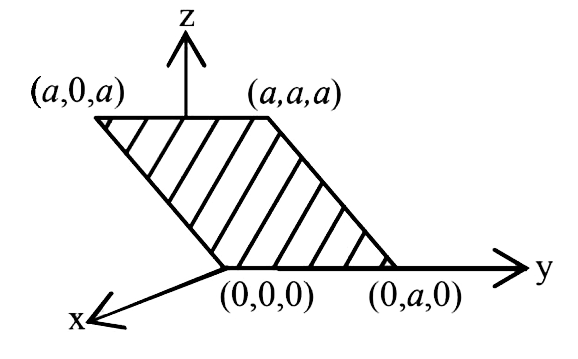
(a) $2 E_{0} a_{2}$

B

(b) $\sqrt{2} E_{0} a^{2}$

C

(c) $E_{0} a^{2}$

D

(d) $\frac{E_{0} a^{2}}{\sqrt{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Given $\to E = E_{0} ˆ x$
This shows that the electric field acts along +x direction and is a constant. The area vector makes an angle of $45^{\circ}$ with the electric field. Therefore the electric flux through the shaded portion whose area is
$a \times \sqrt{2} a = \sqrt{2} a^{2}$ is $ϕ = \to E . \to A = E A cos θ = E_{0} (\sqrt{2} a^{2}) {cos 45}^{\circ} = E_{0} (\sqrt{2} a^{2}) \times \frac{1}{\sqrt{2}} = E_{0} a^{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $\to E = \frac{E_{0} X}{a} ˆ i (x - m t)$ then flux through the shaded area is:

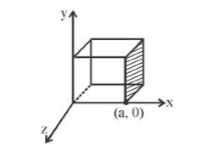A $E_{0} a^{2}$
BZero
C $E_{0} a^{3}$
D $- E_{0} a^{3}$
Question 2 - Select One
If unifrom electric filed $\to E = E_{0} ˆ i + 2 E_{0} ˆ j$ , where $E_{0}$ is a constant, exists in a region of space and at $(0, 0)$ the electric potential $V$ is zero, then the potential at $(x_{0}, 0)$ will be.
Azero
B $- E_{0} x_{0}$
C $- 2 E_{0} x_{0}$
D $- \sqrt{5} E_{0} x_{0}$
Question 3 - Select One
If uniform electric field $\to E = E_{0} ˆ i + 2 E_{0} ˆ j$ , where $E_{0}$ is a constant exists in a region of space and at (0,0) the electric potential V is zero, then the potential at $(x_{0}, 2 x_{0})$ will be -
Azero
B $- 5 E_{0} x_{0}$
C $- 3 E_{0} x_{0}$
D $- \sqrt{5} E_{0} x_{0}$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-ELECTROSTATICS-Comprehension Based Questions

Home
Ask Doubt
Profile