Consider a case of fixed smooth sphere of radius R . A block of mass m is placed at the most poit of the sphere . A sharp impulse is applied on the block to impart it a speed v .
What si the maximum possible value of v so that block does not lose contact with the sphere at the top point ?
√5gR
√3gR
√gR
0
The correct Answer is:C
To solve the problem, we need to analyze the forces acting on the block at the topmost point of the sphere and apply the principles of circular motion.
1. Identify the Forces Acting on the Block:
At the topmost point of the sphere, the forces acting on the block of mass m are:
- The weight of the block acting downwards: mg
- The normal reaction force N from the sphere acting upwards.
2. Apply the Condition for Circular Motion:
For the block to maintain circular motion, the net force acting towards the center of the sphere must provide the necessary centripetal force. This can be expressed as:
mg−N=mv2R
where v is the speed of the block at the topmost point and R is the radius of the sphere.
3. Condition for Maintaining Contact:
The block will lose contact with the sphere when the normal force N becomes zero. Therefore, for the block to just maintain contact, we set N=0:
mg−0=mv2R
Simplifying this gives:
mg=mv2R
4. Cancel Mass m:
Since m is non-zero, we can divide both sides by m:
g=v2R
5. Rearranging for v:
Rearranging the equation to solve for v gives:
v2=gR
Taking the square root of both sides, we find:
v=√gR
6. Conclusion:
The maximum possible value of v so that the block does not lose contact with the sphere at the top point is:
vmax=√gR
At the topmost point of the sphere, the forces acting on the block of mass m are:
- The weight of the block acting downwards: mg
- The normal reaction force N from the sphere acting upwards.
For the block to maintain circular motion, the net force acting towards the center of the sphere must provide the necessary centripetal force. This can be expressed as:
mg−N=mv2R
where v is the speed of the block at the topmost point and R is the radius of the sphere.
The block will lose contact with the sphere when the normal force N becomes zero. Therefore, for the block to just maintain contact, we set N=0:
mg−0=mv2R
Simplifying this gives:
mg=mv2R
Since m is non-zero, we can divide both sides by m:
g=v2R
Rearranging the equation to solve for v gives:
v2=gR
Taking the square root of both sides, we find:
v=√gR
The maximum possible value of v so that the block does not lose contact with the sphere at the top point is:
vmax=√gR
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (Assertion Reason Type Questions)
13 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (Matrix Match Type Questions)
2 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (C. Multiple Choice Questions)
16 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A motor cycle rides in a hollow sphere in a verticle circle of radius 30 m .What will be the minimum speed required so that he does not lose contact with the surface of sphere at the highest point?
View SolutionA particle of mass m is kept on the top of a smooth sphere of radius LR. It is given a sharp impulse whichimparts it a horizontal speed v. a. find thenormal force between the sphere and the particle just after the impulse. B. What should be the minimum value of v for which the particle does not slip on the sphere? c. Assuming the velocity v to be half the minimum calculated in part, d. find teh angle made by the radius through the particle with the vertical when it leaves the sphere.

View Solution
Knowledge Check
Consider a case of fixed smooth sphere of radius R . A block of mass m is placed at the most poit of the sphere . A sharp impulse is applied on the block to impart it a speed v .
What is the normal reaction between block and the sphere just after the impulse is applied ?Amv2RBmg−mv2RCmgD0Consider a case of fixed smooth sphere of radius R . A block of mass m is placed at the most poit of the sphere . A sharp impulse is applied on the block to impart it a speed v .
If a speed given at top is √gR3 , then what is the angle made by radius through block when it leaves the sphere ?Acos−1(23)Bcos−1(34)Ccos−1(59)Dcos−1(79)A motor cycle rides in a hollow sphere in a verticle circle of radius 30 m .What will be the minimum speed required so that he does not lose contact with the surface of sphere at the highest point?
A5.442km/sB17.422cm/sC17.32m/sD54.22m/s
A particle of mass m is kept at the top of a smooth fixed sphere. It is given a horizontal velocity v then:
View SolutionA cart of mass M has a block of mass m in contact with it as shown in the figure-2.133. The coefficient of friction between the block and the cart is μ. What is the minimum acceleration of the cart so that the block m does not fall ?

View SolutionA block of 0.5kg is placed on a horizontal platform. The system is making vertical oscillations about a fixed point with a frequency of 0.5Hz. The maximum displacement of oscillation, if the block does not lose contact with the horizontal platform is nearly
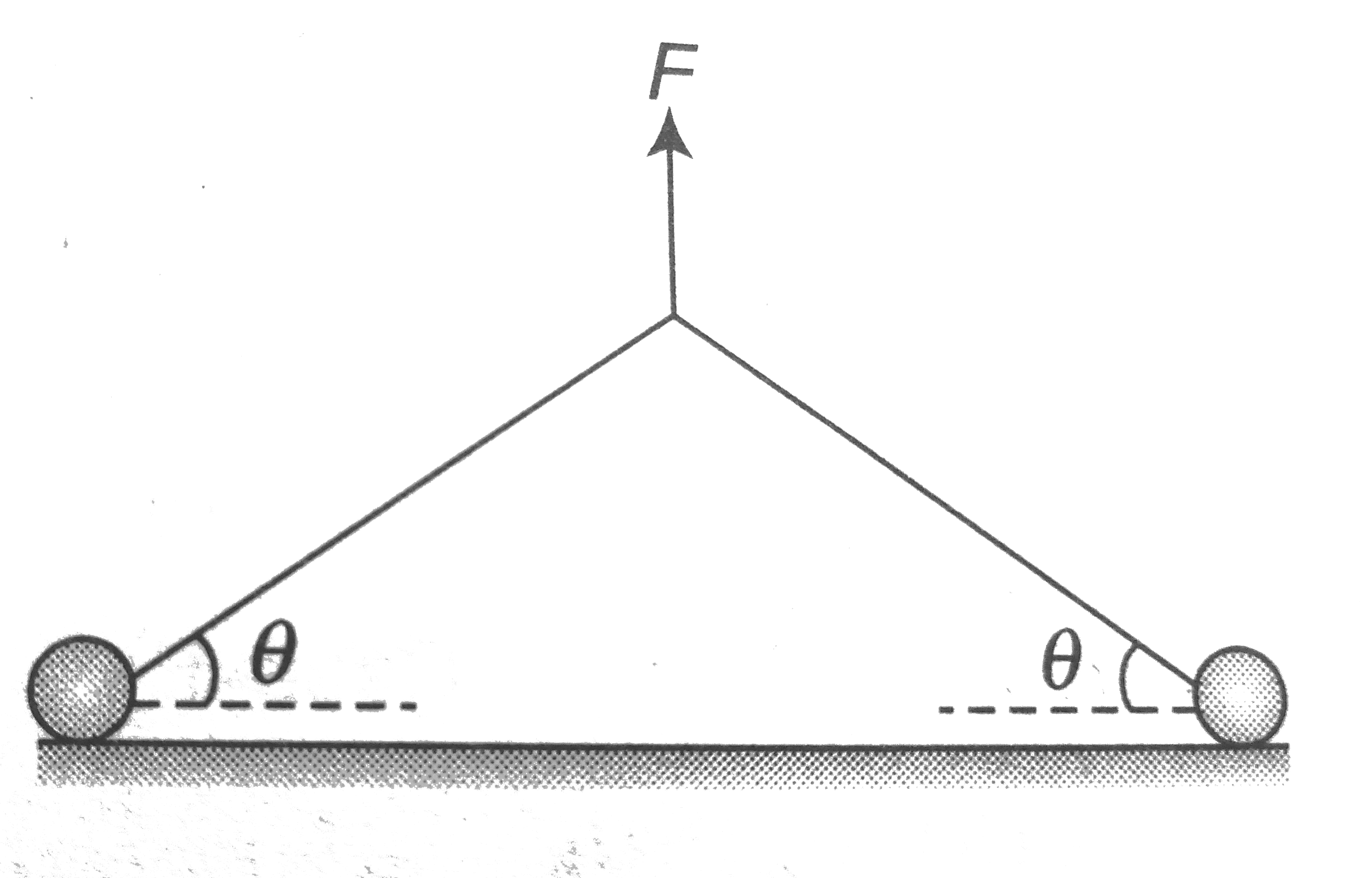
View SolutionTwo small sphere each of mass m connected by a string of length 2l are kept on a smooth horizontal surface. A vertical force F is applied at the middle of the string. What is maximum value of F for which the sphere do not lose contact with the surface?
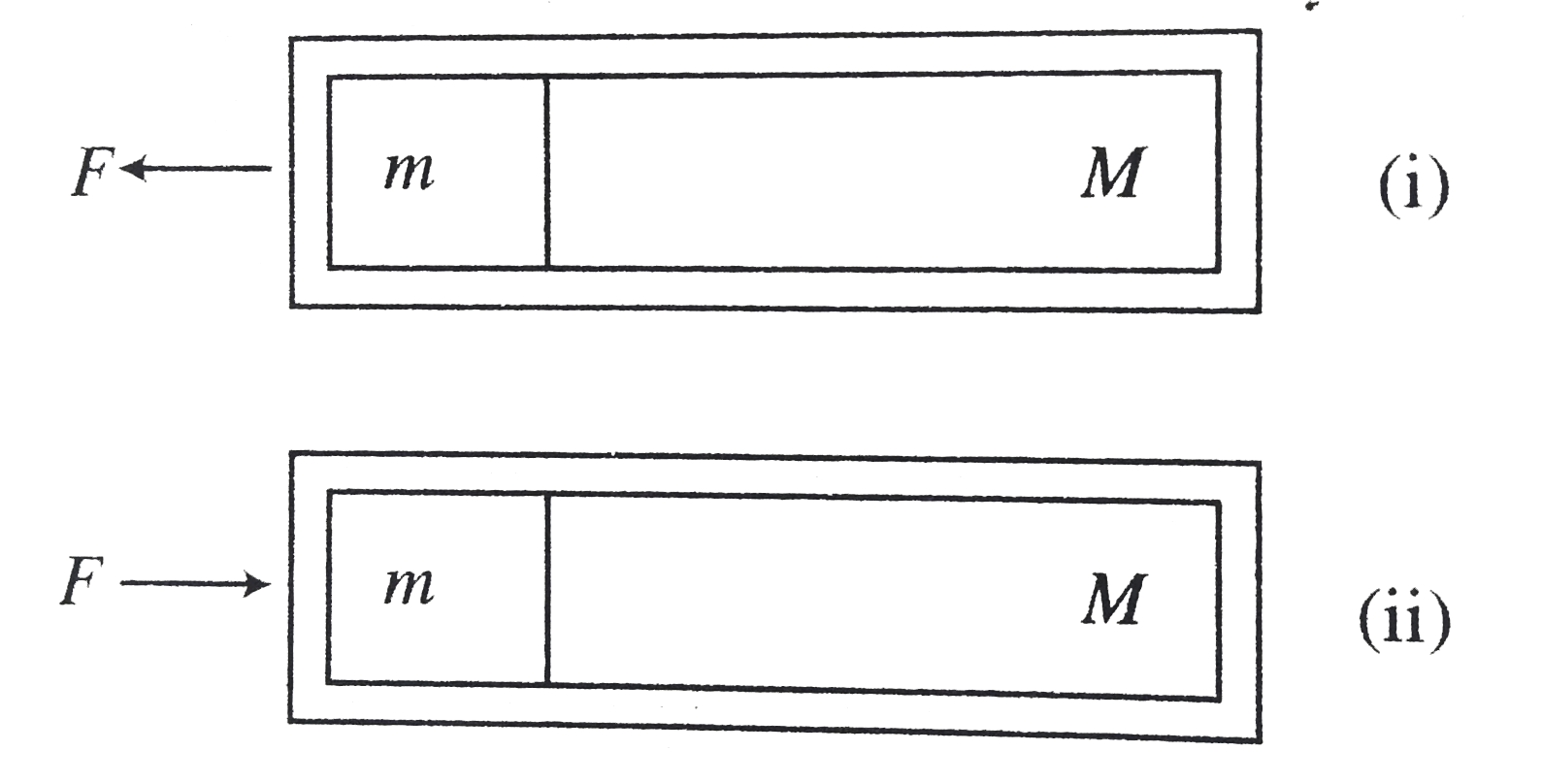
View SolutionA block of mass m is placed in contact with one end of a smooth tube of mass M. A horizontal force F acts on the tube in each case (i) and (ii). Then,
View Solution
