Consider slabs of three media A,B, and C. Arragned as shown inn figure . R.I of A is 1.5 and that of C is 1.4. If the number of waves in the combination B and C then refractive index of B is
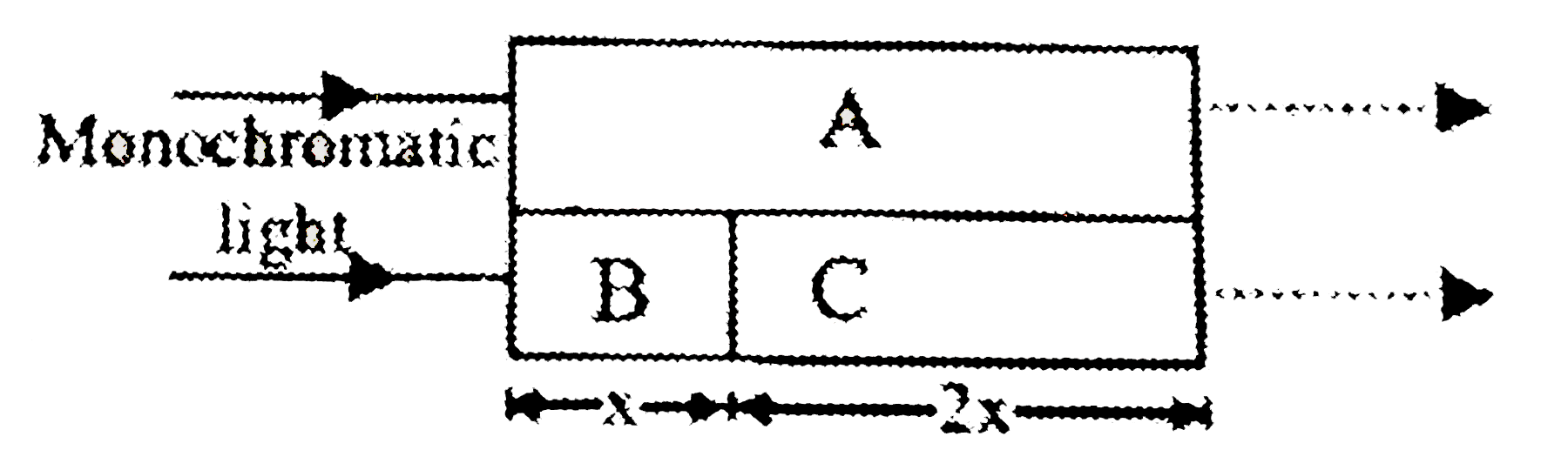
1.4
1.5
1.6
1.7
The correct Answer is:4
nA=nB+nc
μAdA=μBdB+μCdC
1.5(3x)=μB(x)+1.4(2x)
4.5=μ+2.8
μB=4.5−2.8=1.7
Topper's Solved these Questions
Similar Questions
a,b,c are three numbers such that the average of a and b is 4, that of b and c is 5 and that of a and c is 12. The average of all the three numbers is :
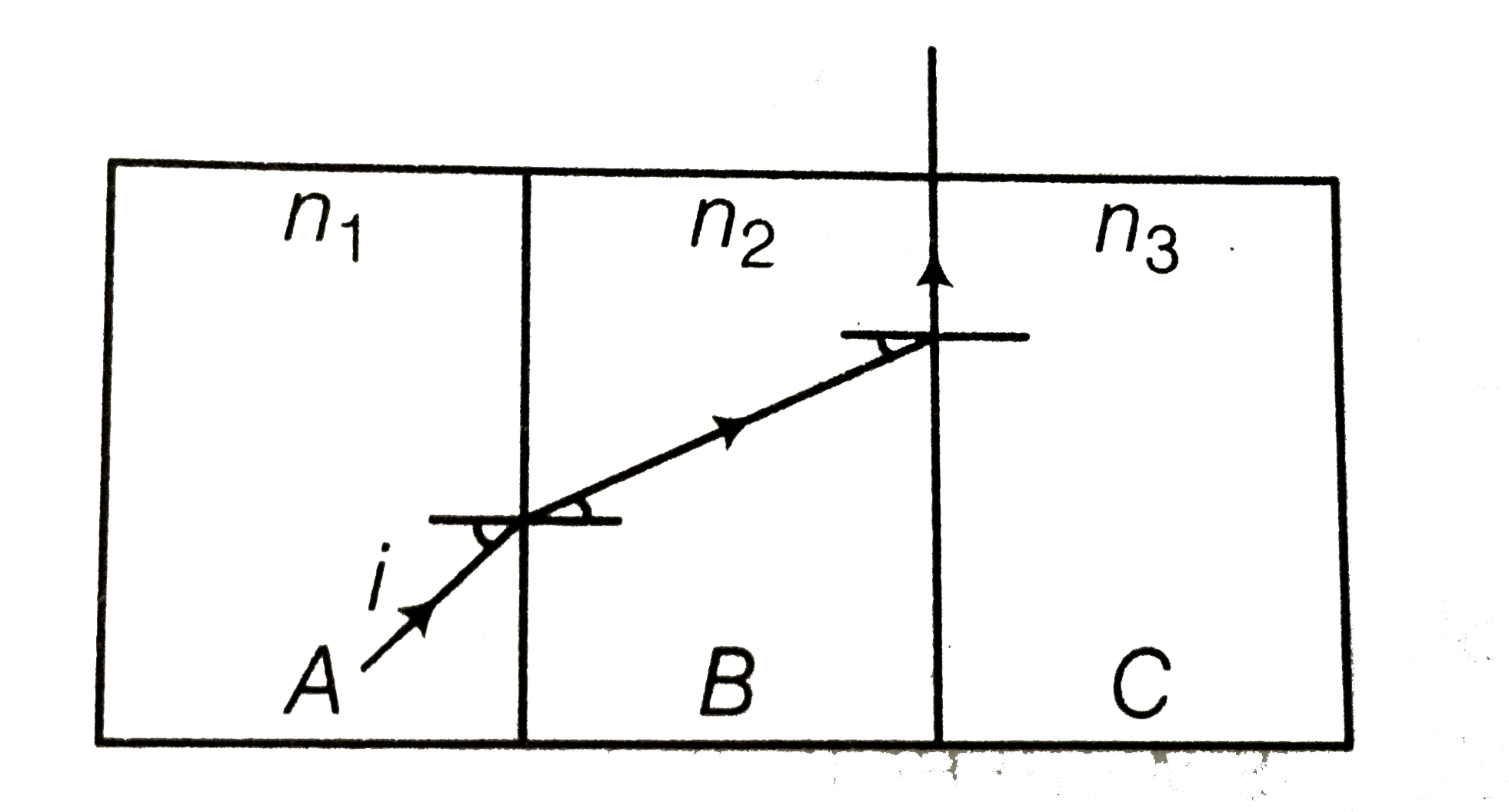
View SolutionA,B, and C are the parallel sided transparent media of refractive indices n1,n2 and n3 respectively. They are arranged as shown in the figure. A ray is incident at an angle i on the surface of separation of A and B which is as shown in the figure. After the refraction into the medium B the ray grazes the surfaces of separation of the media B and C. then sini equal to
View Solution
Knowledge Check
A slab of transparent material is made as shown in Fig. Monochromatic parallel beams of light are normally incident on the slab. The thickness of C is twice the thickness of B. If the number of waves in A = number of waves in combination of BandC, then the refractive index of B is :
 .A1.33B1.8C1.6D1.6
.A1.33B1.8C1.6D1.6Three progressive waves A,B and C are shown in figure. With respect to wave A
_S01_014_Q01.png) .AThe wave C lags behind in phase by π/2 and B leads by π/2BThe wave C leads in phase by π and B lags behind by πCThe wave C leads in phase by π/2 and B lags behind by π/2DThe wave C lags behind in phase by π and B leads by π
.AThe wave C lags behind in phase by π/2 and B leads by π/2BThe wave C leads in phase by π and B lags behind by πCThe wave C leads in phase by π/2 and B lags behind by π/2DThe wave C lags behind in phase by π and B leads by πTwo transparent slabs have the same thickness as shown in figure. One in made of material A of refractive index 1.5. The other is made of two materilas B and C with thickness in the ratio 1:2. The refractive index of C is 1.6. If a monochromatic parallel beam passing through the slabs has the same number of wavelengths inside both, the refractive index of B is
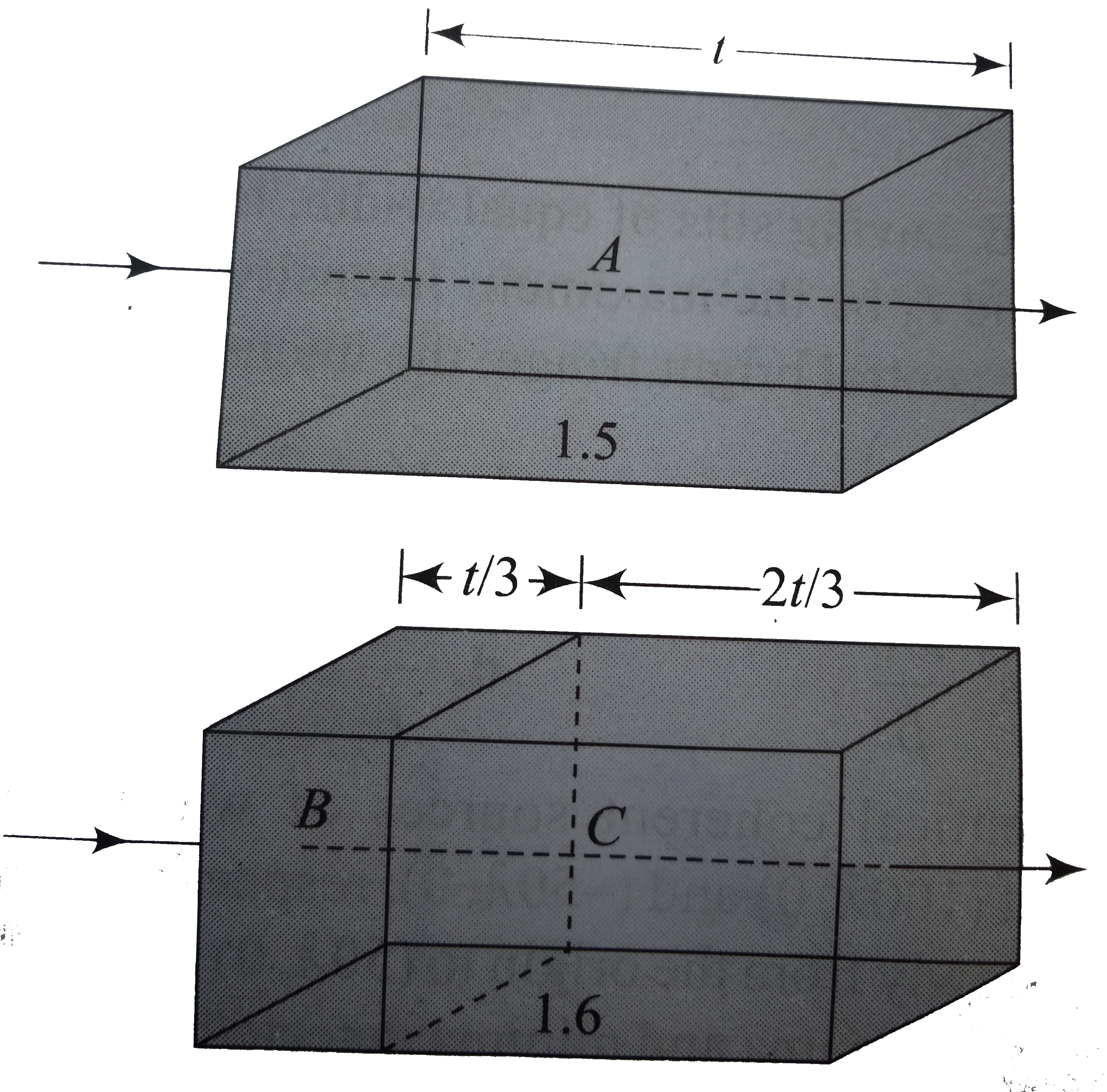 A1.1B1.2C1.3D1.4
A1.1B1.2C1.3D1.4
Refractive index of glass slab shown in figure is 1.5. Focal length of mirror is 20cm. Find
(a) total number of reflections before final image is formed.
(b) reduced steps.
(c) value of x, so that final image coincides with the object.

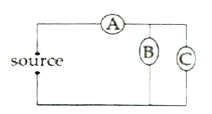
View SolutionThree similar bulbs A, B, C are connected to a voltage source as shown in the figure. If C is removed, how will the illumination of A and B be affected ?
View SolutionThree progressive waves A,B,C are shown in the figure
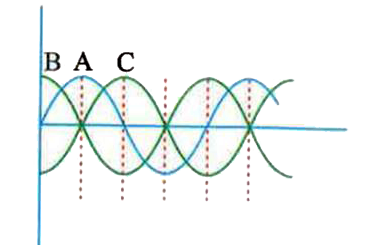
With respect to A, the progressive wave
View SolutionConsider three geometrically identical concave lenses A, B and C. A is made of material of refractive index μ1, B is made of material of refractive index μ2. Lens C is constructed in such a way that half of the lens above principal axis is made of material of refractive index μ1, and half of the lens below principal axis is made of material of refractive index μ2. Image of an object O as shown in figure is formed at I1 when lens A is used and the image is formed at I2 when lens B is used at the same location. Now the lens C is placed at the same location.

View SolutionSix resistance each of value r=5Ω are connected between points A, B and C as shown in figure. If R1,R2 and R3 are the net resistance between A and B, between B and C and between A and C respectively, then R1:R2:R3 will be equal to
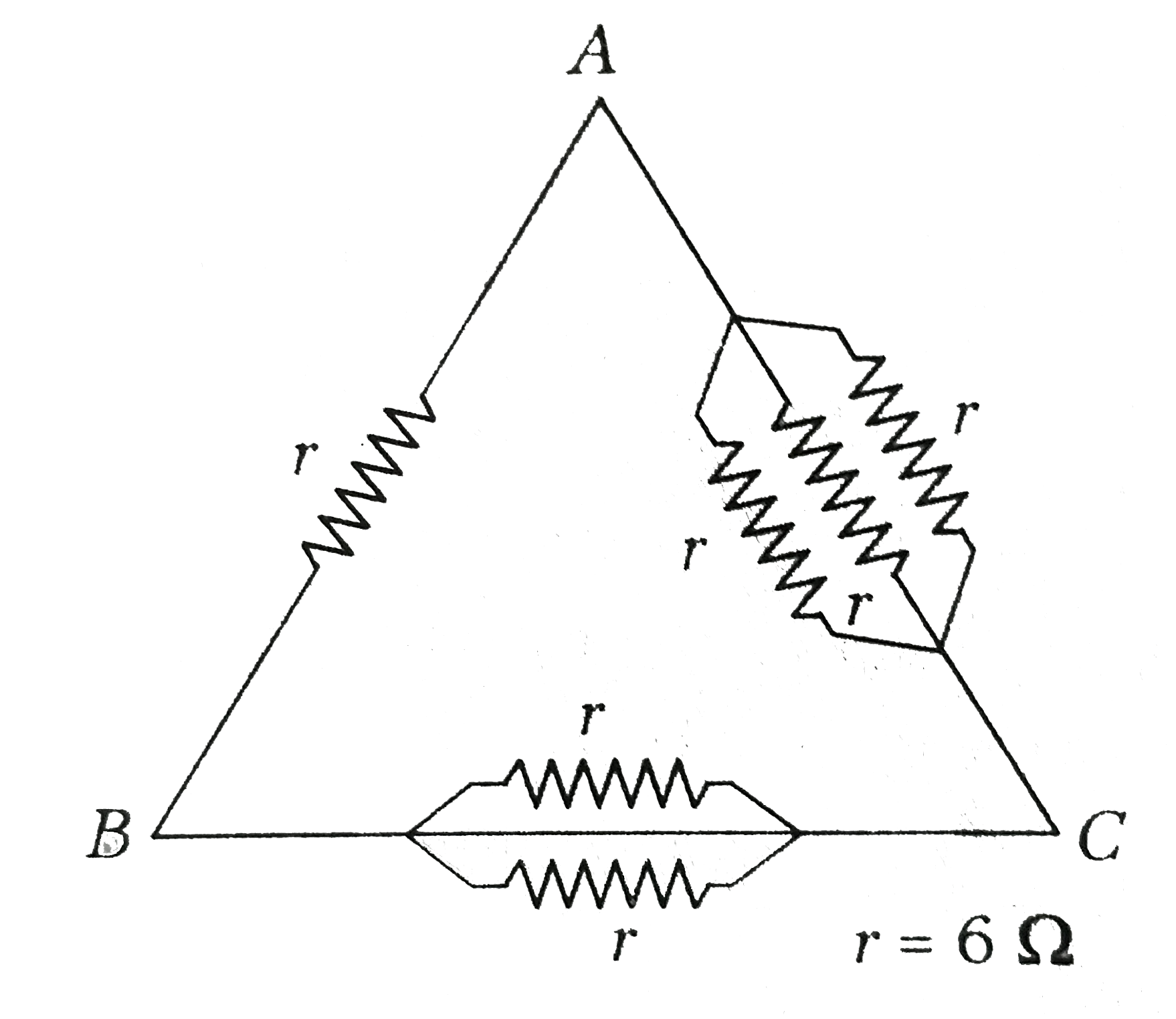
View Solution
