Consider the following two equations
L=Iω
dLdt=Γ
In noninertial frames
both A and B are true
A is true but B is false
B is true but A is false
both and B are false
The correct Answer is:B
To analyze the given equations in the context of non-inertial frames, let's break down the problem step by step.
Step 1: Understanding the First Equation
The first equation given is:
L=Iω
Where:
- L is the angular momentum,
- I is the moment of inertia,
- ω is the angular velocity.
In non-inertial frames, the definition of angular momentum remains valid. The moment of inertia I and angular velocity ω are still defined in the same way. Therefore, this equation holds true in non-inertial frames.
Step 2: Understanding the Second Equation
The second equation is:
dLdt=Γ
Where:
- dLdt is the rate of change of angular momentum,
- Γ is the torque.
In non-inertial frames, if there are no external torques acting on the body, the total torque would be zero, leading to:
dLdt=0
This means that the angular momentum L remains constant if no external torques are present. However, if we consider the presence of fictitious forces in non-inertial frames, the torque can be affected, and thus the equation may not hold as it does in inertial frames.
Conclusion
1. The first equation L=Iω is true in non-inertial frames.
2. The second equation dLdt=Γ is false in non-inertial frames because it does not account for the effects of fictitious forces and torques that can arise in such frames.
Final Answer
Based on the analysis:
- The first statement is true.
- The second statement is false.
Thus, the correct option is: A is true but B is false.
---
The first equation given is:
L=Iω
Where:
- L is the angular momentum,
- I is the moment of inertia,
- ω is the angular velocity.
The second equation is:
dLdt=Γ
Where:
- dLdt is the rate of change of angular momentum,
- Γ is the torque.
dLdt=0
This means that the angular momentum L remains constant if no external torques are present. However, if we consider the presence of fictitious forces in non-inertial frames, the torque can be affected, and thus the equation may not hold as it does in inertial frames.
1. The first equation L=Iω is true in non-inertial frames.
2. The second equation dLdt=Γ is false in non-inertial frames because it does not account for the effects of fictitious forces and torques that can arise in such frames.
Based on the analysis:
- The first statement is true.
- The second statement is false.
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 1
26 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
Knowledge Check
Consider the following statements : For any two sets A and B
(1)(A-B) ∪B=A
(2) (A-B)∪ A=A
(3) (A-B) ∩B=ϕ
(4) A⊆B⇒A∪B=B of these statements:A(A−B)∪B=AB(A−B)∪A=AC(A−B)nB=ϕDA⊆B⇒A∪B=BConsider the following two statements .
A : Kinetic energy depends on frame of reference .
B : Linear momentum does not depend on frame of reference .ABoth A and B are false .BBoth A and B are true .CA is true and B is false .DB is true and A is false .Consider following two reactions
A→Product,=d[A]dt=−k−(1)[A]0
B→Product,−d[B]dt=k2[B]
k1 and k2 are expressed in terms of molarity (molL−1 and time s−1) asAs−1,Ms−1L−1BMs−1,Ms−1Cs−1,M−1s−1DMs−1,s−1
Let α,βandγ be real numbers. Consider the following system of linear equations
x+2y+z=7
x+αz=11
2x−3y+βz=γ
Match each entry in List-I to the correct entries in List-II.
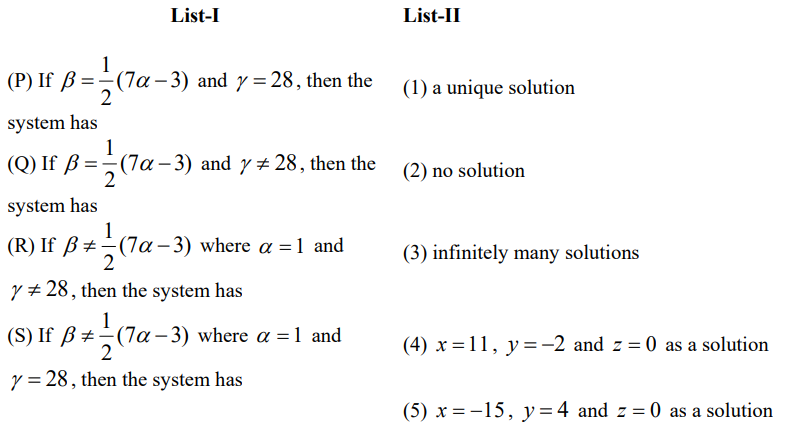
The correct option is:
View Solutionconsider the following two triangles as shown in the figure below .
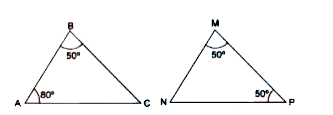
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion Earth is an inertial frame.
Reason A frame in motion is sometimes an inertial frame and sometimes a non-inertial.
View SolutionConsider the following two statements:
A The linear momentum of a particle is independent of the frame of reference.
B. The kinetic energy of a particle is independent of the frame of reference
View SolutionThe population growth is generally described by the following equation : dNdt=rN(K−NK) What does 'r' represent in the given equation ?
View Solution
