Consider the situation shown in figure. The wire which has a mass of 4.00 g oscillates in its second harmonic and sets the air column in the tube into vibrations in its fundamental mode. Assuming that the speed of sound in air is 40ms−1, find the tension in the wire.
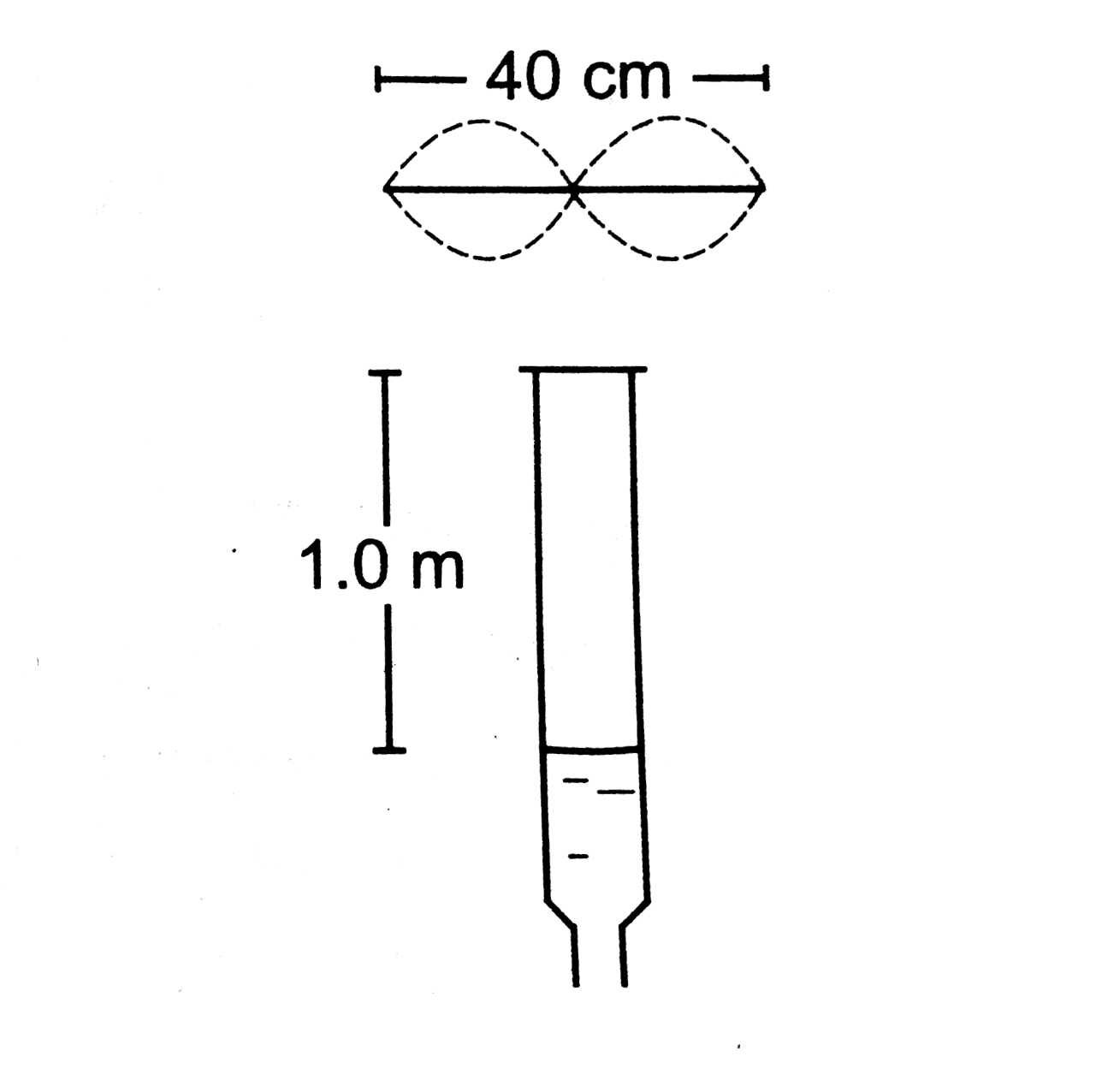 .
.
The correct Answer is:A
Let n0=frequency of the tuning fork,
T=Tension of the string
L=40cm=0.4m,m=4g
=4×10−3kg
So,m=MassUnitLen>h=10−2kgm
n0=12L√Tm
So, 2ndHarmonic2n0=(22L)√Tm
As it is tension with fundamental frequency of vibration in the air column.
→2n0=3404×1=85Hz
→85=22×0.4√T14
=T=(85)2×(0.4)2
=11.6New→n
Topper's Solved these Questions
- SOUND WAVESBOOK - HC VERMACHAPTER - SOUND WAVESEXERCISE - Objective -2
5 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
- SPECIFIC HEAT CAPACITIES OF GASESBOOK - HC VERMACHAPTER - SPECIFIC HEAT CAPACITIES OF GASESEXERCISE - Exercises
35 Videos
Similar Questions
A wire of length 40cm which has a mass of 4 g oscillates in its second harmonic and sets the air column in the tube to vibrations in its funrations in its fundamental mode as shows in figure. Assuming the speed of sound in air as 340m/s . Find the tension in the wire.

View SolutionThe separation between a node and the next antinode in a vibrating air column is 25 cm. If the speed of sound in air is 340ms−1, find the frequency of vibration of the air column.
View Solution
Knowledge Check
A steel wire 0.5 m long has a total mass of 0.02 kg and is stretched with a tension of 800N. The frequency when it vibrates in its fundamental mode is
A2HzB141HzC100HzD200HzA steel piano wire 0.5 m long has a total mass of 0.01 kg and is stretched with a tension of 800 N. The frequency, when it vibrates in its fundamental mode, is
A2 HzB4 HzC100 HzD200 Hz
A tube 1.0m long is closed at one end. A stretched wire is placed near the open end. The wire is 0.3m long and a mass of 0.01kg . It is held fixed at both ends and vibrates in its fundamental mode. It sets the air column in the tube into vibration at its fundamental frequency by resonance. Find
(a) the frequency of oscillation of the air column and
(b) the tension in the wire.
Speed of sound in air = 330m/s .
View SolutionAn air column enclosed in an open pipe is vibrating in its fundamental mode . The fundamental frequency is 30 Hz. If the velocity of sound air is 300ms−1 , find the length of the pipe and frequency of 3rd overtone .
View SolutionA tube 1.0m long is closed at one end. A stretched wire is placed near the open end. The wire is 0.3m long and a mass of 0.01kg . It is held fixed at both ends and vibrates in its fundamental mode. It sets the air column in the tube into vibration at its fundamental frequency by resonance. Find
(a) the frequency of oscillation of the air column and
(b) the tension in the wire.
Speed of sound in air = 330m/s .
View SolutionThe air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440 Hz. The speed of sound in air is 330 m/s. find the length of air column.
View SolutionA closed organ pipe can vibrate at a minimum frequency of 500 Hz. Find the length of the tube. Speed of sound in air =340ms−1.
View SolutionA string 25cm long and having a mass of 2.5gm is under tension. A pipe closed at one end is 40cm long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, 8 beats per second are heard. It is observed that decreasing the tension in the string decreases beat frequency. if the speed of sound in air is 320m/s, find the tension in the string.
View Solution
