Deduce an expression for the electric field due to the system of point charges.

Text Solution

Verified by Experts

(i) Suppose a number of point charges are distributed in space, to find the electric field at some point P due to this collection of point charges. Superposition principle is used.
(ii) The electric field due to a collection of point charges at an arbitrary point is simply equal to the vector sum of electric fields created by the individual point charges. This is called superposition of electric fields.
(iii) Consider a collection of point charges $q_{1}, q_{2}, q_{3}, ... ... ., q_{n}$ located various points in space. The total electric field at some point P due to all these n charges is given by
${\to E}_{tot} = {\to E}_{1} + {\to E}_{e} + {\to E}_{3} + ... ... + {\to E}_{0} ... . (1)$
${\to E}_{tot} = \frac{1}{4 π ε_{0}} {\frac{q_{1}}{r_{1 P}^{2}} {ˆ r}_{1 P} + \frac{q_{2}}{r_{2 P}^{2}} {ˆ r}_{2 P} + \frac{q_{3}}{r_{3 P}^{2}} {ˆ r}_{3 P} + ... . \frac{q_{n}}{r_{n P}^{2}} {ˆ r}_{n P}} ... . (2)$
(iii) Here $r_{1 P} r_{2 P} r_{3 P} ... ... . r_{n P}$ are the distance between the point P and the charges $1_{1 P} q_{2 P}, q_{3 P} ... . . q_{n P}$ respectively. Also ${ˆ r}_{1 P}, {ˆ r}_{2}, {ˆ r}_{3 P} ... ... . . {ˆ r}_{n P}$ ar the unit vectors directed from $q_{1 P}, q_{2 P}, q_{3 P}, ... . . q_{n P}$ respectively to P. Equation (2) can be re-written as
${\to E}_{tot} = \frac{1}{4 π ε_{0}} n \sum i = 1 (\frac{q_{1}}{r_{i P}^{2}} {ˆ r}_{i P})$
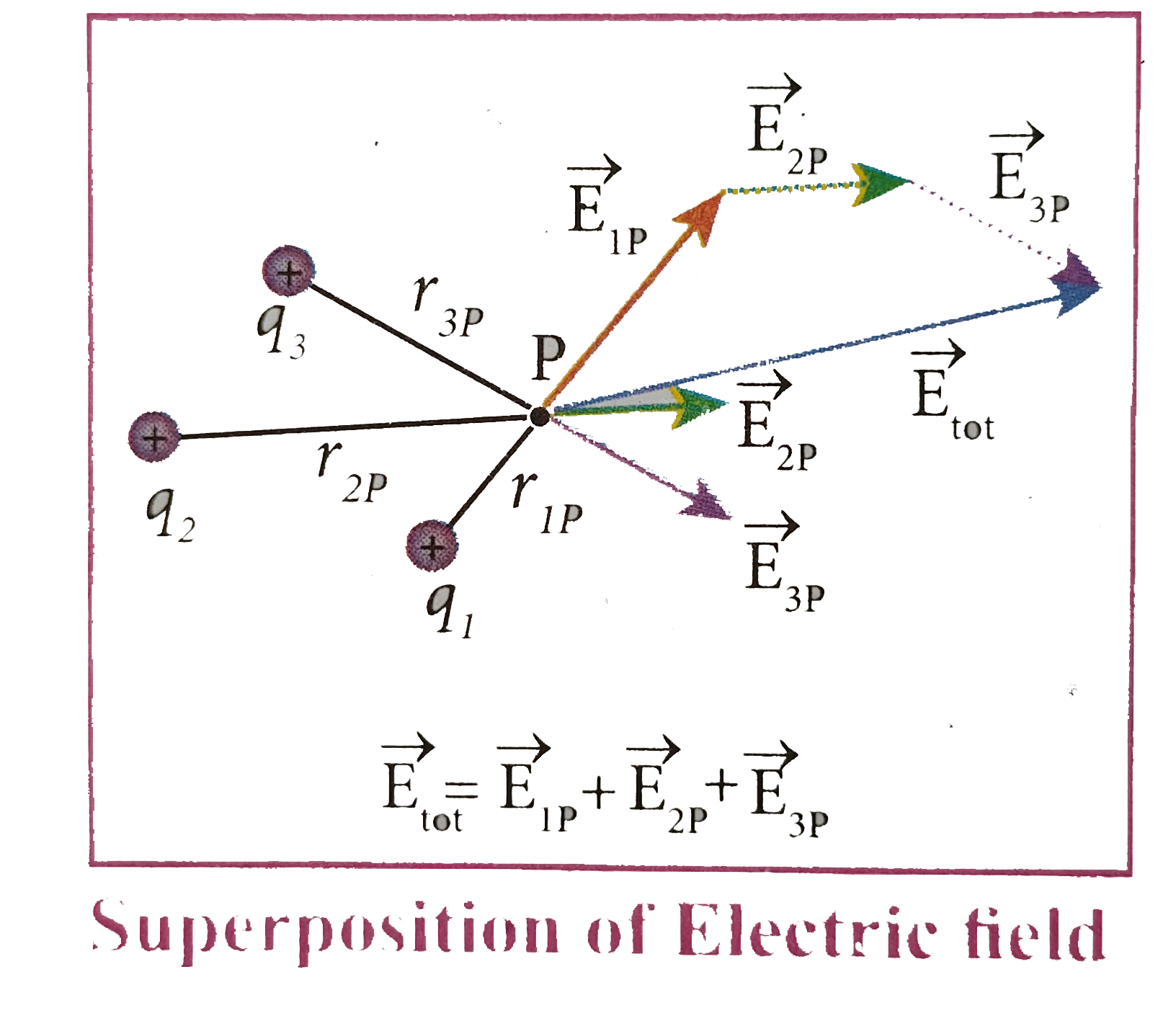
(iv) for example in Figure, the resultant electric field due to three point charges $q_{1}, q_{2}, q_{3}$ at point P is show.
Note that the relative lengths of the electric field vector for the charges depend on relative distance of the chargs to the point P.

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile