Determine the possible harmonics in the longitudinal vibrations of a rod clampred in the middle.

Video Solution

Text Solution

Verified by Experts

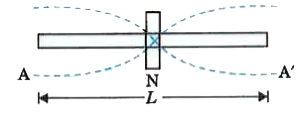
The rod of length L is clamped in the middle as shown in the adjoining figure. It has one node in the middle and two antinodes at its free ends in the fundamental mode.
$∴ L = 2 . \frac{λ_{1}}{4} or λ_{1} = 2 L$
Fundamental frequency or first harmonic is
$v = \frac{υ}{λ_{1}} = \frac{υ}{2 L}$

Now, in the second mode, as shown in the adjoining figure, an additional node and antinode appear on the two sides of the clamp
$∴ L = 6 . \frac{λ_{2}}{4} or λ_{2} = \frac{2 L}{3}$
Also, $v_{2} = \frac{υ}{λ_{2}} = \frac{3 υ}{2 L} = 3 v$
This is the third harmonic or first overtone.
Similarly for third mode, two additional nodes and antinoes appear on the two sides of the clamp.
$∴ v_{3} = \frac{5 υ}{2 L} = 5 v$
This is the fifth harmonic or second overtone.
So, odd no. of harmonic are possible.

Vibrations in a rod are
Atransverse
Blongitudinal
Ceither 'a' or 'b'
Dboth 'a' and 'b'
The longitudinal strain is only possible in
AGases
BFluids
CSolids
DLiquids
A particle executes simple harmonic motion. The amplitude of vibration of particle is 2 cm. The displacement of particle in one time period is
A1 cm
B2 cm
C4 cm
Dzero