Explain reversible and irreversible processes. Describe the working of carnot engine. Obtain an expression for the efficiency.
Text Solution
Reversible process : In reversible process a thermodynamic system can be retraced back in opposite direction to the changes that take place in the direct process or in forward process. Finally the system must be in thermal equilibrium with surroundings even after returning to original state with no other change any where else in the universe.
A reversible process is only an ideal concept.
Conditions for reversible process : A reversible process must obey the following conditions.
1. Changes must take place at an infinitesimally slow rate.
2. The system must always be in thermal and mechanical equilibrium with surroundings.
3. There should be no loss of energy in any form.
4. No amount of heat is to be converted into electric or magnetic forms.
Examples for reversible process :
1. Peltier effect and Seebeck effect.
2. Fusion of ice and vapourisation of water.
Irreversible process : A thermodynamic process that cannot be taken back in opposite direction is called an irreversible process.
Examples : 1. Work done against friction.
2. Magnetization of materials.
In an irreversible process the thermodynamic system cannot be operated in reverse direction without loss of energy or system cannot be taken to original state without loss of energy.
3. All spontaneous natural processes are irreversible.
4. Diffusion of gases.
5. Joule heating in a conductor by passing current through it.
Carnot.s Engine : Carnot.s engine works on the principle of reversible process within the temperatures T1andT2.
It consists of four continuous processes. The total process is known as Carnot Cycle.
Step 1 : In carnot cycle the 1st step consists of isothermal expansion of gases. So temperature T is constant P, V changes are shown as P1V1T1to−−→P2V2T1
Work done in isothermal process
W1→2=Q1=μRT1loge(V2V1) ...(1)
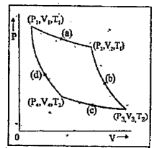
Step 2 : In this stage gases will expand adiabatically. So energy to the system Q is constant. So P, V relation is
P2V2T1to−−→P3V3T2
Work done in adiabatic process
W2→3=μR(T1−T2)γ−1 ...(2)
Step 3 : In this stage gases will be compressed isothermally. So P1V changes are
P3V3T2to−−→P4V4T2
Work done in isothermal compression
W3→4=Q2=μRTloge(V3V4) ...(3)
Step 4 : In the fourth stage the gas suffers adiabatic compression and returns to original stage.
So P, V changes are P4V4T2to−−→P1V1T1
Work done W4→1=μR(T2−T1)γ−1 ...(4)
Total work done in Carnot Cycle W=W1,2+W2,3+W3,4+W4,1
Total work done
W=μRT1log(V2V1)+μRγ−1(T1−T2)−μRT2log(V3V4)−μRγ−1(T1−T2)
∴W=μRT1log(V2V1)−μRT2log(V3V4)
The total work done W=Q1−Q2 i.e., the difference to heat energy absorbed from source and heat energy given to sink.
Efficiency of Carnot engine η=work done by Carnot engineheat energy supplied
∴η=Q1−Q2Q1orη=1−Q2Q1=1−T2T1
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The efficiency of carnot engine depends on
Atemperature of sink onlyBtemperature of source and sinkCvolume of cylinder of engineDtemperature of sourceThe efficiency of a Carnot heat engine
Ais independent of the temperature of the source and the sinkBis independent of the working substanceCcan be 10%Din not affected by the thermal capacity of the source of the sinkEfficiency of Carnot engine is 100% if
AT2=273KBT2=0KCT1=273KDT1=0K
Explain
(i) Reversible and irreversible process
(ii) Isothermal and adiabatic process.
View SolutionThe efficiency of carnot engine depends on
View SolutionReversible and Irreversible Processes || Comparision OF Work Done in Reversible and Irreversible Processes
View SolutionExplain why a Carnot engine cannot have 100% efficiency?
View SolutionThe effiency of reversible engine is___the irreversible engine.
View Solution
