Fig. shows the plot of the function $y (x)$ representing a fraction of the total power of thermal radiation falling within the spectral interval from $0$ to $x$ . Here $x = λ / λ_{m} (λ_{m}$ is the wavelength corresponding to the maximum of spectral radiation density).
Using this plot, find:
(a) the wavelength which divides the radiation spectrum into two equal (in terms of energy) parts at the temperature $3700 K$ ,
(b) the fraction of the total radiation power falling within the visible range of the spectrum $(0.40 - 0.76 μ m)$ at the temperature $5000 K$ ,
(c) how manu times the power radiated at wavelengths exceeding $0.76 μ m$ will increase if the temperature rises form $3000$ to $5000 K$ .
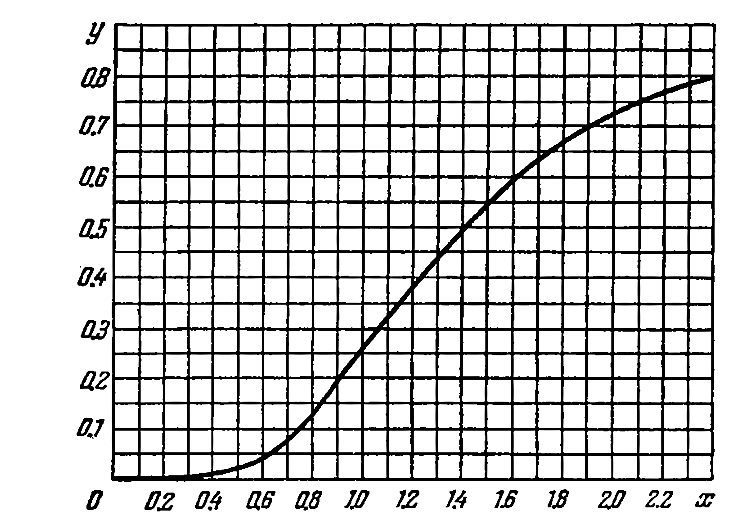
$Fig. shows the plot of the function y(x) representing a fraction of the total power of thermal radiation falling within the spectral interval from 0 to x. Here x = lambda//lambda(m)(lambda(m) is the wavelength corresponding to the maximum of spectral radiation density). Using this plot, find: (a) the wavelength which divides the radiation spectrum into two equal (in terms of energy) parts at the temperature 3700K, (b) the fraction of the total radiation power falling within the visible range of the spectrum (0.40-0.76 mu m) at the temperature 5000K, (c) how manu times the power radiated at wavelengths exceeding 0.76mu m will increase if the temperature rises form 3000 to 5000K.$

Text Solution

Verified by Experts

(a) From the curve of the function $y (x)$ we see that $y = 0.5$ when $x = 1.41$
Thus $λ = 1.41 \times \frac{0.29}{3700} c m = 1.105 μ m$
(b) At $5000 K$
$λ = \frac{0.29}{5} \times 10^{- 6} m = 0.58 μ m$
So the visible range $(0.40$ to $0.70) μ m$ corresponds to a range $(0.69$ to $1.31)$ of $x$ . From the curve
$y (0.69) = 0.07$
$y (1.31) = 0.44$
so the fraction is $0.37$
(c ) The value of $x$ corresponding to $0.76$ are
$x_{1} = 0.76 / \frac{0.29}{0.3} = 0.786$ at $3000 K$
$x_{2} = 0.76 / \frac{0.29}{0.5} = 1.31$ at $5000 K$
The requisite fraction is then
$(\frac{P_{2}}{P_{1}}) = {(\frac{T_{2}}{T_{1}})}^{4} ↑ ratio of t otal power \times \frac{1 - y_{2}}{1 - y_{1}} ↑ ratio of the fra ction of required wavel eng ths i n the radiated power$
$= {(\frac{5}{3})}^{4} \times \frac{1 - 0.44}{1 - 0.12} = 4.91$

$λ_{m}$ is the wavelength of the radiations corresponding to maximum intensity of a very very hot body at temperature T. Which of the following correctly represents the relations between $λ_{m}$ and T:
A $λ_{m}$ decreases with increase in T
B $λ_{m}$ increases with increase in T
C $λ_{m}$ is independent of T
DNone of the above
if $λ_{v'}, λ_{x}$ and $λ_{m}$ represent the wavelengths of visible light X-rays and microwaves respectively then:
A $λ_{m} > λ_{x} > λ_{y}$
B $λ_{x} > λ_{m} > λ_{y}$
C $λ_{m} > λ_{y} > λ_{x}$
D $λ_{y} > λ_{x} > λ_{m}$
The thermal emissivitites of two bodies $A$ and $B$ are in the ratio of $1 / e$ . The outer surface area of the bodies are same and they radiate the energy at the same rate. Find the ratio of the wavelength corresponding to the maximum spherical radiance in the radiation from $A$ to maximum spectral radiance in the radiation from $B$ .
A $e^{1 / 4}$
B $\frac{1}{e^{1 / 4}}$
C ${(1 + e)}^{1 / 4}$
D $\frac{1}{{(1 + e)}^{1 / 4}}$