Figure shows an arragement of four identical rectangular plates A, B, C and D each of area S.Thickness of plates is negligible. Then :
Potential difference between plates A and B is independent of Q1
Potential difference between plates C and D is independent of Q1
Potential difference between plates A and B is independent of Q2
Potential difference between plates C and D is indpendent of Q2
The correct Answer is:A, B
To solve the problem regarding the potential differences between the plates A, B, C, and D, we will follow a systematic approach.
1. Understanding the Arrangement:
- We have four identical rectangular plates A, B, C, and D, each with an area S. The thickness of the plates is negligible.
- The arrangement is such that the plates are parallel to each other, and we need to analyze the potential differences between them.
2. Potential Difference Formula:
- The potential difference V between two plates can be expressed as:
V=E⋅d
where E is the electric field between the plates, and d is the distance between them.
3. Electric Field Calculation:
- The electric field E between two plates with charge Q is given by:
E=Qϵ0A
- Here, ϵ0 is the permittivity of free space, and A is the area of the plates.
4. Analyzing Potential Difference Between Plates A and B:
- Let’s denote the charge on plate A as Q1 and on plate B as −Q1.
- The potential difference VAB between plates A and B can be calculated as:
VAB=E⋅dAB=Q1ϵ0S⋅a
- Here, a is the distance between plates A and B.
5. Independence from Charge Q1:
- The potential difference VAB is directly proportional to Q1, but since we are looking for independence from Q1, we need to consider the net effect of other charges in the system.
- The potential difference between A and B can be shown to be independent of Q1 when considering the contributions from other charges in the system.
6. Analyzing Potential Difference Between Plates C and D:
- Similarly, for plates C and D, let’s denote the charge on plate C as Q2 and on plate D as −Q2.
- The potential difference VCD can be expressed as:
VCD=E⋅dCD=Q2ϵ0S⋅d
- Here, d is the distance between plates C and D.
7. Independence from Charge Q2:
- The potential difference VCD can also be shown to be independent of Q2 by analyzing the total charge distribution and the resultant electric field.
8. Final Conclusion:
- After analyzing both potential differences, we conclude that:
- The potential difference between plates A and B is independent of Q1.
- The potential difference between plates C and D is independent of Q2.
- Therefore, the correct options are:
- Potential difference between A and B is independent of Q1.
- Potential difference between C and D is independent of Q2.
- We have four identical rectangular plates A, B, C, and D, each with an area S. The thickness of the plates is negligible.
- The arrangement is such that the plates are parallel to each other, and we need to analyze the potential differences between them.
- The potential difference V between two plates can be expressed as:
V=E⋅d
where E is the electric field between the plates, and d is the distance between them.
- The electric field E between two plates with charge Q is given by:
E=Qϵ0A
- Here, ϵ0 is the permittivity of free space, and A is the area of the plates.
- Let’s denote the charge on plate A as Q1 and on plate B as −Q1.
- The potential difference VAB between plates A and B can be calculated as:
VAB=E⋅dAB=Q1ϵ0S⋅a
- Here, a is the distance between plates A and B.
- The potential difference VAB is directly proportional to Q1, but since we are looking for independence from Q1, we need to consider the net effect of other charges in the system.
- The potential difference between A and B can be shown to be independent of Q1 when considering the contributions from other charges in the system.
- Similarly, for plates C and D, let’s denote the charge on plate C as Q2 and on plate D as −Q2.
- The potential difference VCD can be expressed as:
VCD=E⋅dCD=Q2ϵ0S⋅d
- Here, d is the distance between plates C and D.
- The potential difference VCD can also be shown to be independent of Q2 by analyzing the total charge distribution and the resultant electric field.
- After analyzing both potential differences, we conclude that:
- The potential difference between plates A and B is independent of Q1.
- The potential difference between plates C and D is independent of Q2.
- Therefore, the correct options are:
- Potential difference between A and B is independent of Q1.
- Potential difference between C and D is independent of Q2.
Similar Questions
Figure shows an arrangement of four identical rectangular plates A,B,C, and D each of area S. Find the charges appearing on each face (from left to right) of the plates. Ignore the separation between the plates in comparison to the plate dimensions.
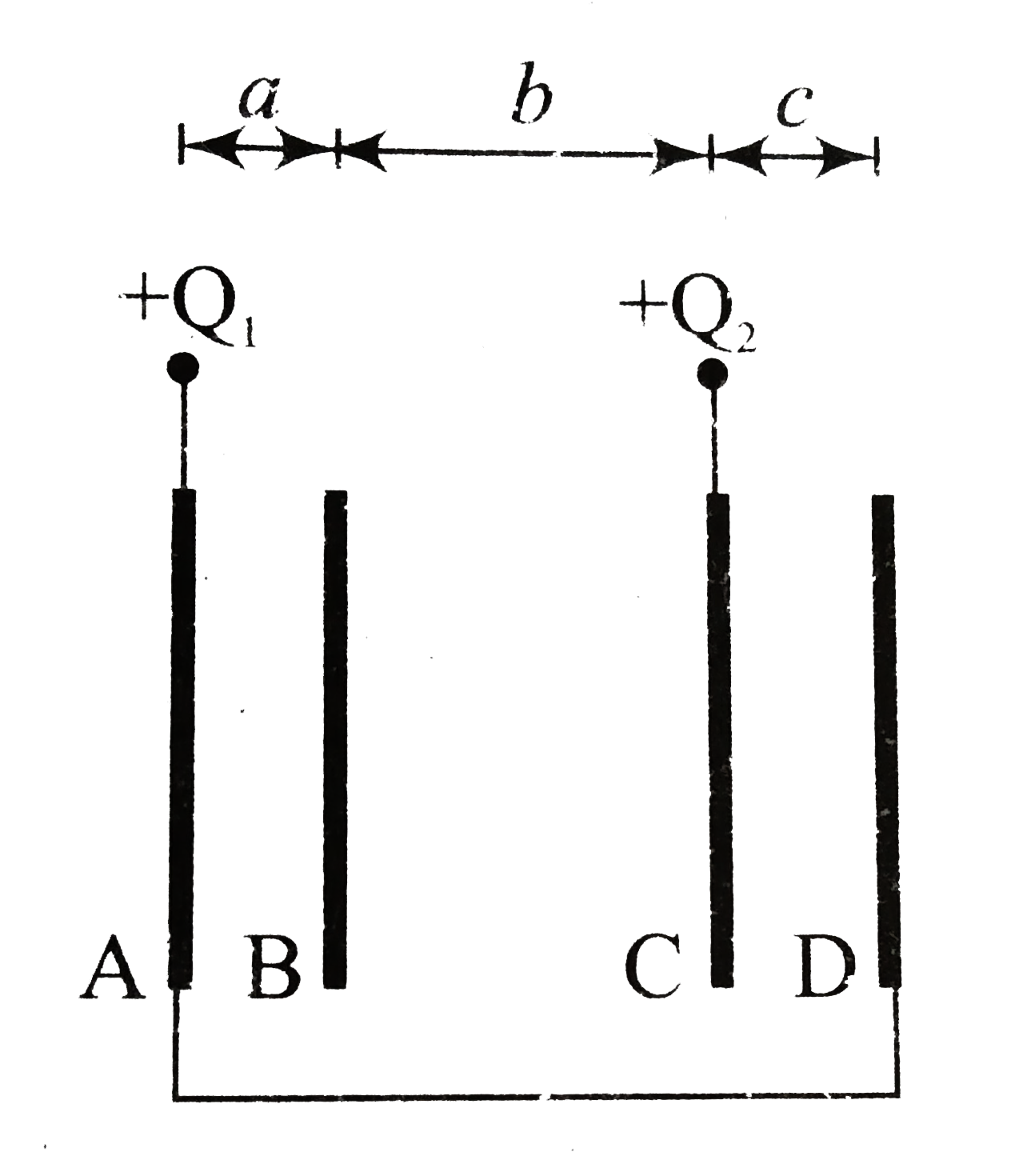
View SolutionThe diagram shows an arrangement of identical metal plates placed parallel to each other and the variation of potential between the paltes by dotted lilne. Using the details given in diagram, the equivalent capacitance connected across the plate No. 2 and plate No. 4 is
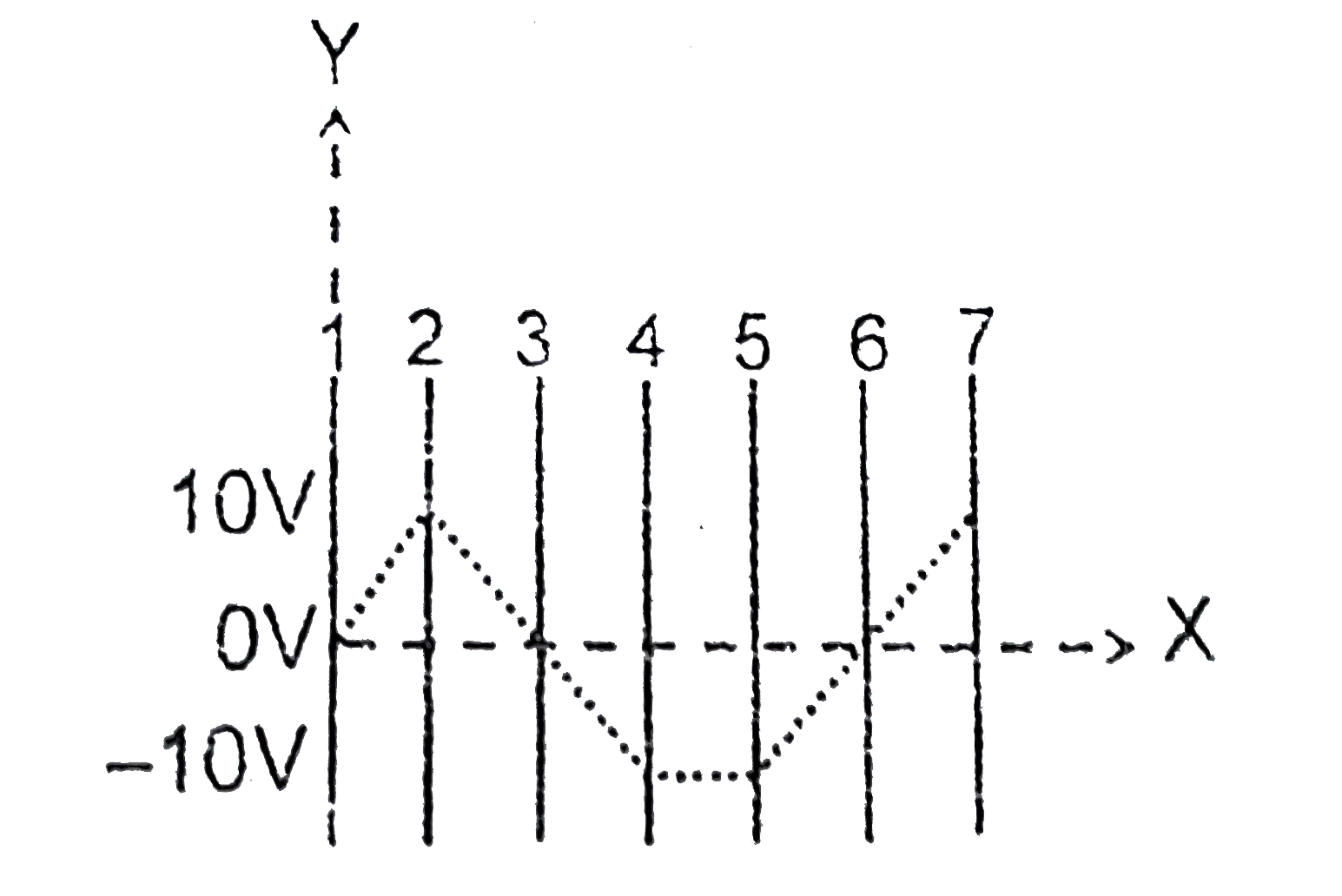
(Separation between the consecutive plates =L, area of each plate =A)
View SolutionThe following arrangement consists of five identical metal plates parallel to each other. Area of each plate is A and separation between the successive plates is d. The capacitance between P and Q is

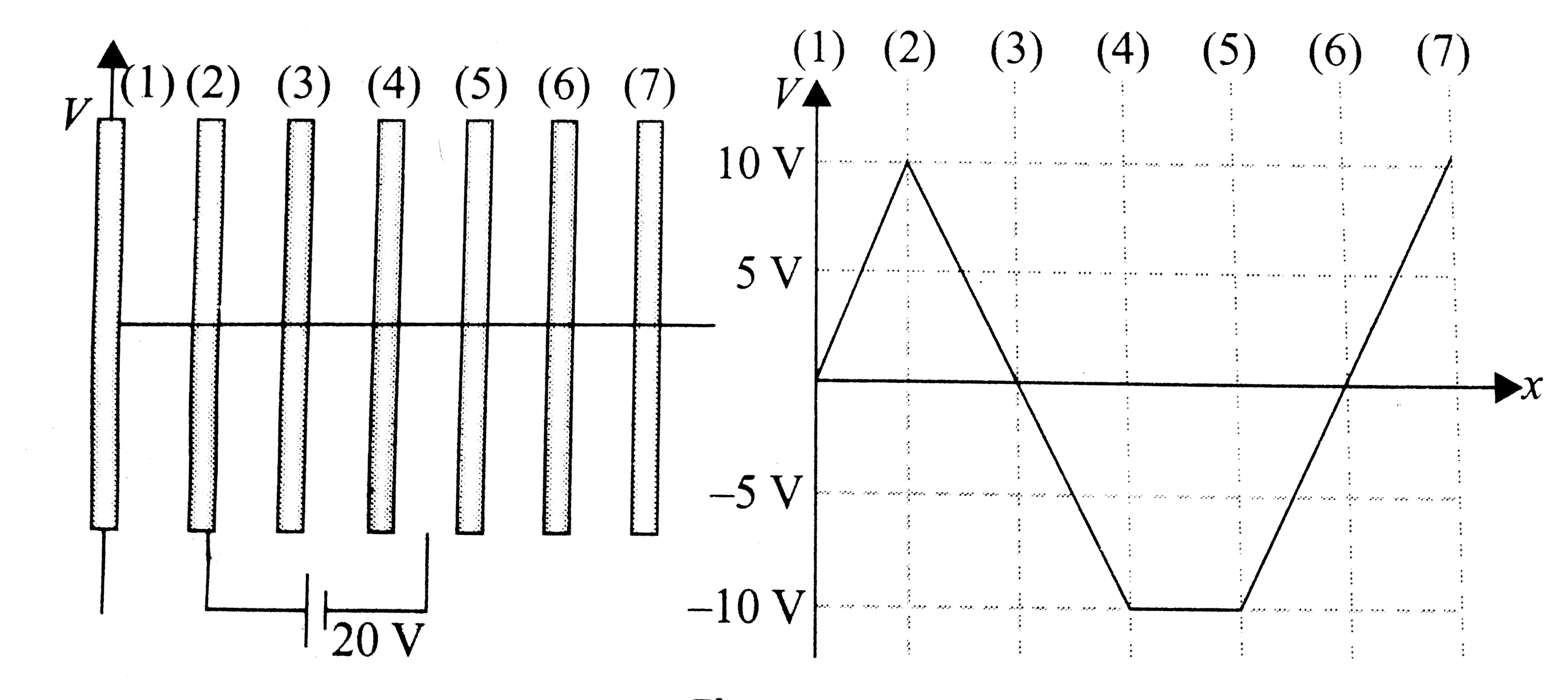
View SolutionShows an arrangement of identical metal plates paraed parallel to each other. The diagram also shows the variation of potential berween the plates . Using the details given in the diagram, find the equivalent capacitance connected across the battery (sepaaration between the consecutive plates is equal to l and the cross-sectional area of each plate is A).
 .
.
View SolutionFour initially uncharaged thin, large, plane idential metallic plates A,B,C and D are arranged parallel to each other as shown. Now plates A,B,C and D are given charges Q,2Q,3Q and 4Q respectively. Plates A and D are connected by a mtallic wire while plates B and C connected by other metallic wire then after Electrostatic equilibrium is reached.

Total charge on plates after earthing plates A and B will be
View SolutionFour initially uncharaged thin, large, plane idential metallic plates A,B,C and D are arranged parallel to each other as shown. Now plates A,B,C and D are given charges Q,2Q,3Q and 4Q respectively. Plates A and D are connected by a mtallic wire while plates B and C connected by other metallic wire then after Electrostatic equilibrium is reached.

Total charge on plates after earthing plates A and B will be
View SolutionCharges 2q and −3q are given to two identical metal plates of area of cross section A. the distance between the plates is d. Find the capacitance and potential difference between the plates.
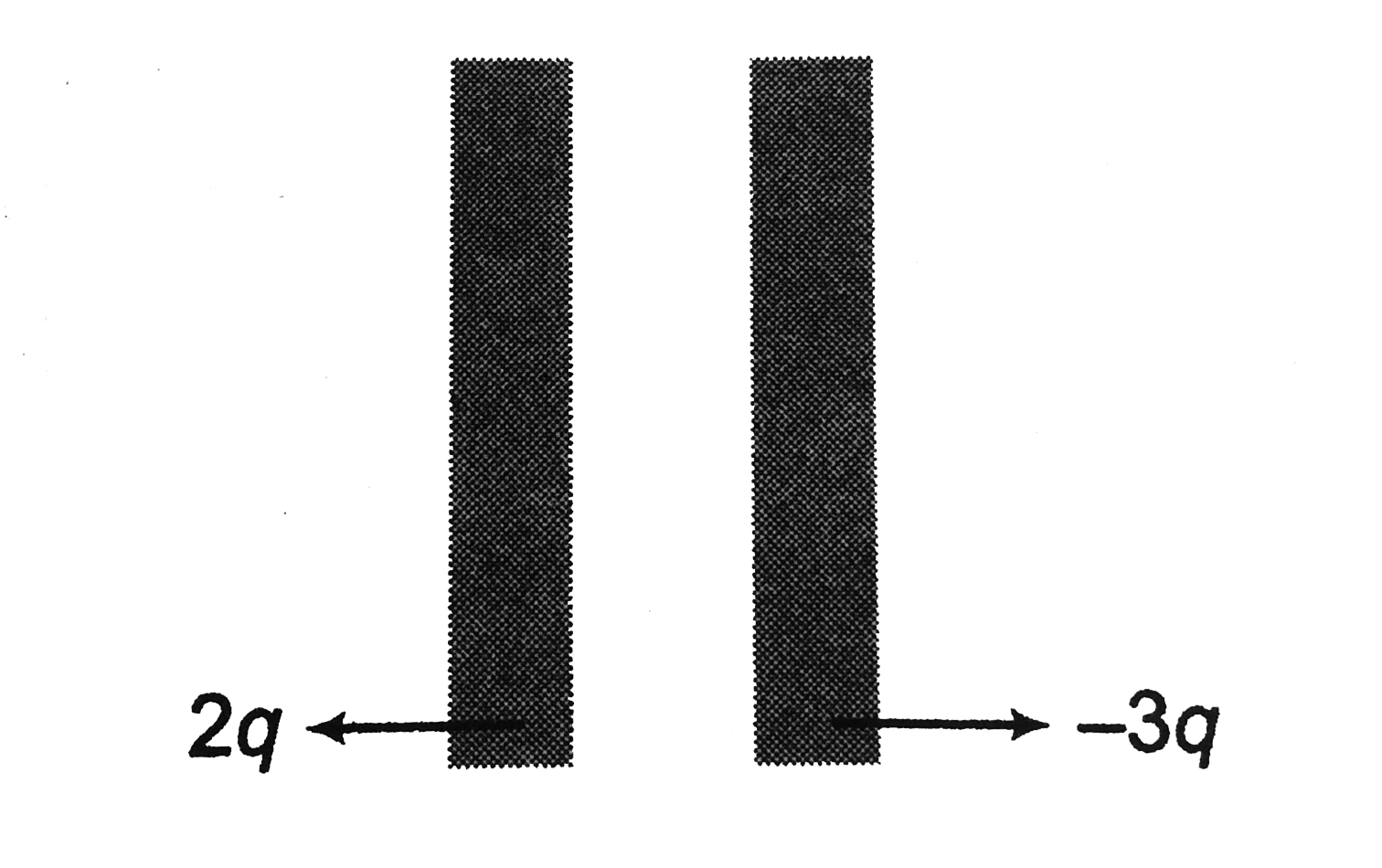
View SolutionAll the plates shown in the figure are parallel to each other, the area of each plate is A, and the separation between each pair of facing plates is d. The equivalent capacitance between points A and B is
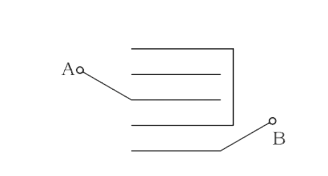
View SolutionTwo plates of equal area are placed in contact with each other. The thickness of the plates are 2.0 cm and 3.0 cm respectively. The outer face of first plate is at −25∘C and that of second plate is at +25∘C. The conductivities of the plates are in the ratio 2:3. Calculate the temperature of the common surface of the plates.
View SolutionFour metallic plates each with a surface area A of one side and placed at a distance d from each other. the plates are connected as shown in the fig. Then the capacitance of the system between a and b is -
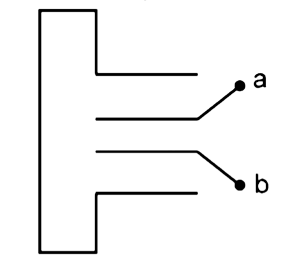
View Solution
