Find the angular momentum of a disc about the axis shown in figure in the following situations.
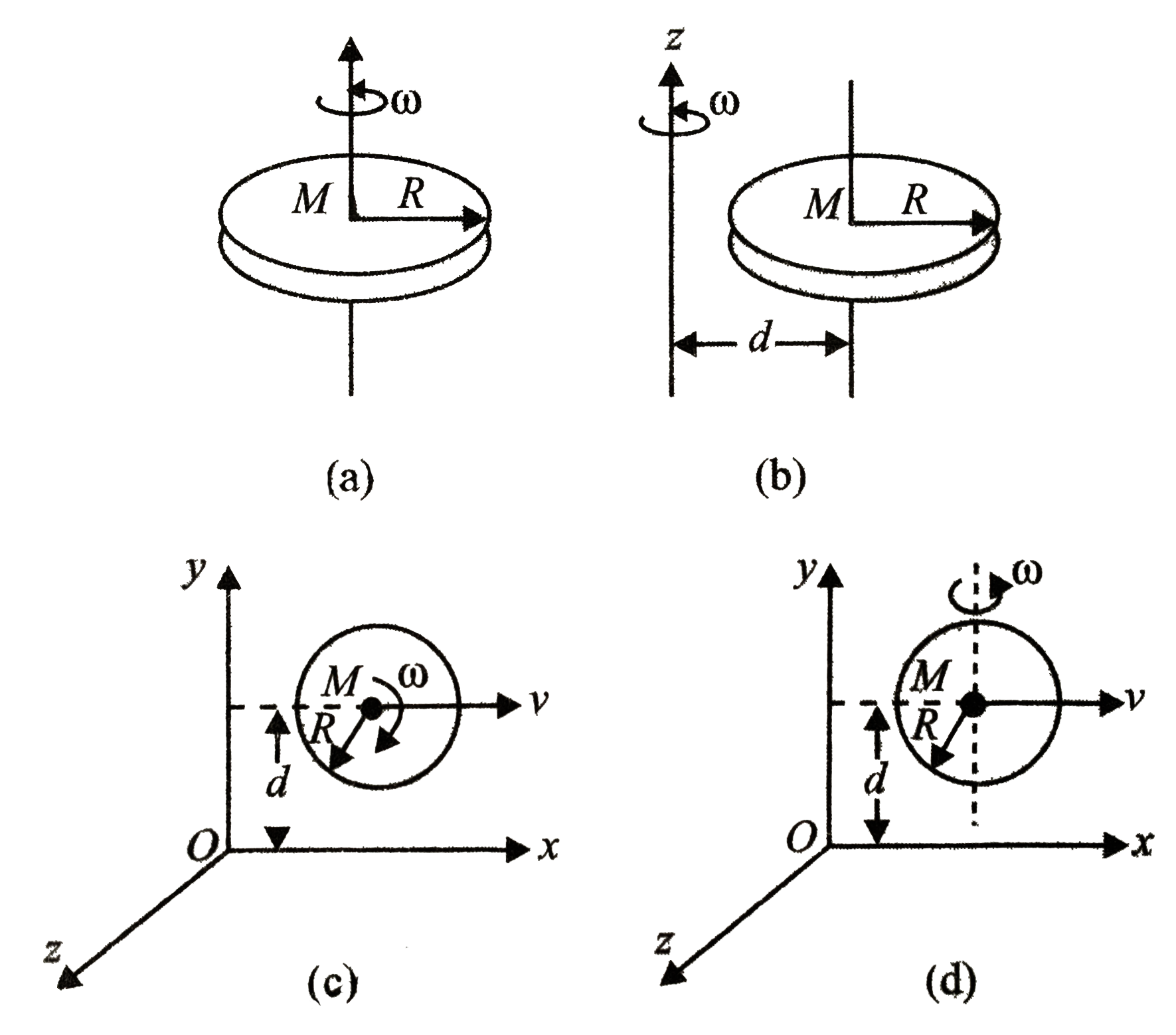
Case a: In this case the disc is rotating about fixed axis which is parallel to the -axis.
direction can be determined using right hand rule.
Case b: In this case also the disc rotates about fixed axis. We can write angular momentum for this situation.
Uisnng parallel axis we can find the value of Hence
Case c: In this case the disc is rotating about an axis parallel to the -axis and centre of disc is also translating.
Hence, angular momentum,
Case d: In the case also the the disc is rotating about an axis passing through it centre and parallel to -axis and centre of mass of disc is translating with velocity parallel to -axis
Here
Hence ,
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples
12 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.1
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
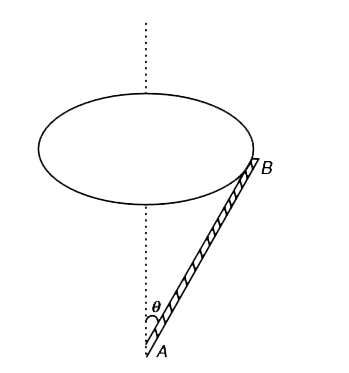
A uniform rod of mass m and length L is fixed to an axis, making an angle θ with it as shown in the figure. The rod is rotated about this axis so that the free end of the rod moves with a uniform speed ‘v’. Find the angular momentum of the rod about the axis. Is the angular momentum of the rod about point A constant?
View SolutionFind the angular momentum of the earth
(a) about the sun due to its orbital motion and
(b) about its axis due to its spinning motion.
View Solution
Knowledge Check
A disc of mass M and radius R is rolling with angular speed ω on a horizontal plane. The magnitude of angular momentum of the disc about a point on ground along the line of motion of disc is :
A(1/2)MR2ωBMR2ωC(3/2)MR2ωD2MR2ωA disc of mass m and radius R moves in the x−y plane as shown in Fig. The angular momentum of the disc about the origin O at the instant shown is
 A−52mR2ωˆkB73mR2ωˆkC−92mR2ωˆkD52mR2ωˆk
A−52mR2ωˆkB73mR2ωˆkC−92mR2ωˆkD52mR2ωˆk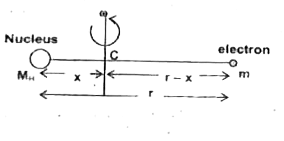
The angular momentum of the atom will be constant about an axis passing throughAnucleusBelectronCcentre of mass of the atomDany axis perpendicular to the line joining the electron and nucleus
In example number 12.16 suppose the disc starts rotating anticlockwise with the sae angular velocity ω=vR, then what will e the angular momentum of te disc about bottommost in this new situation?
View SolutionA smooth horizontal disc rotates with a constant angular velocity ω about a stationary vertical axis passing through its centre, the point O. At a moment t=0 a disc is set in motion from that:
point with velocity v0. Find the angular momentum M(t) of the disc relative to the point O in the reference frame fixed to the disc. Make sure that this angular momentum is caused by the Coriolis force.
View SolutionIn the situation shown in figure, find the velocity of image.
View SolutionA disc of mass m and radius R is rolling on horizontal ground with linear velocity v. What is the angular momentum of the disc about an axis passing through bottommost point and perpendicular to the plane of motion ?
View Solution
The angular momentum of the atom will be constant about an axis passing through
View Solution
