Find the expresstion of potential energy U(x,y,z) for a conservative force in a force field where force is given as →F=yzˆi+xyˆk Consider the zero of the potential energy chosen at the point (2,2,2).
Step by step video & image solution for Find the expresstion of potential energy U(x,y,z) for a conservative force in a force field where force is given as vec(F)=yzhat(i)+xyhat(k) Consider the zero of the potential energy chosen at the point (2,2,2). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - Practice Exercise
42 Videos
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - Discussion Question
31 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
Similar Questions
A conservative force in a region is given by →F=(Ax3)ˆi .An expression for the potential energy in the region, assuming the potential at infinity to be zero, is
View SolutionThe potential energy for a conservative force system is given by U=ax2−bx. Where a and b are constants find out (a) The expression of force (b) Potential energy at equilibrium.
View Solution
Knowledge Check
The relation between conservative force and potential energy U is given by :–
A→F=dUdxB→F=∫UdxC→F=−dUdxD→F=d2Udx2The potential energy U=3ax3−2bx2. The force constant is represented by
A8bB6bC4bD2b.The potential energy for a conservative system is given by
U=ax2−bx
where a and b are positive constants. The law of the force governing the system isAF = constantBF=bx–2aCF=b−2axDF=2ax
The potential energy of a particle under a conservative force is given by U(x)=(x2−3x) J. The equilibrium position of the particle is at
View SolutionA potential energy function for a two-dimensional force is the form U=3x2y−7x. Find the force that acts at the point (x,y).
View SolutionThe potential energy function of a particle in a region of space is given as U=(2xy+yz) J Here x,y and z are in metre. Find the force acting on the particle at a general point P(x,y,z).
View SolutionThe potential energy U for a force field →F is such that U=−kxy where K is a constant . Then
View SolutionFigure shows a plot of the conservative force F in a unidimensional field. The plot representing the function corresponding to the potential energy (U) in the field is
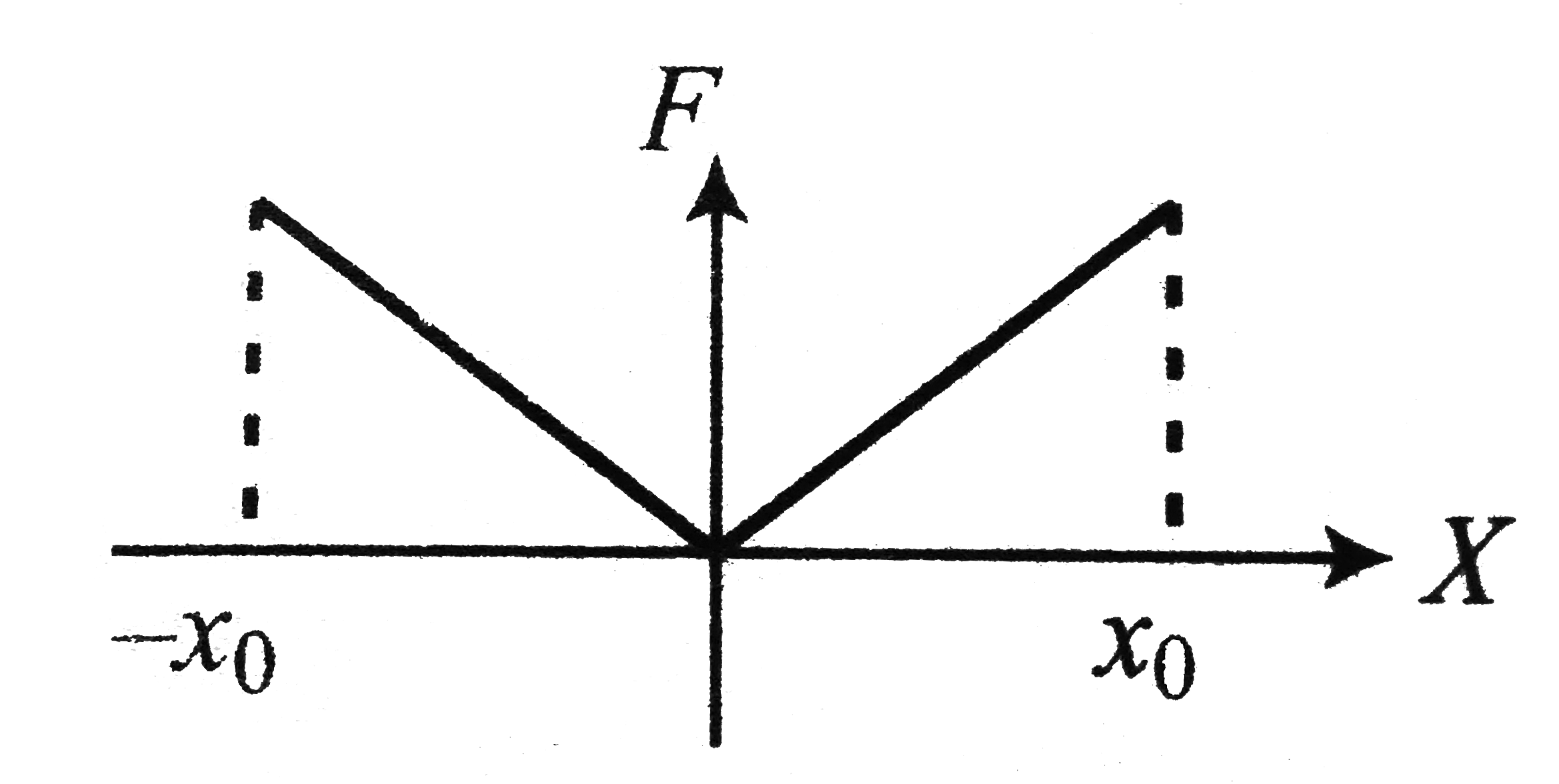
View Solution
