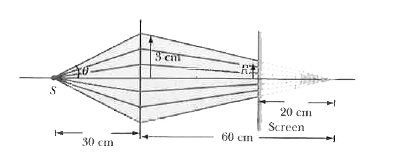
Find the radius of the light spot on the screen as shown in Fig. 34-44. Consider only the light rays refracted from the lens. Find the average intensity on the screen. The source is point source of power 100W. The lens has an aperture of 6cm.
(1) Only a real image can be focused on a screen. A virtual image cannot be focused on a screen but can be seen with naked eyes. To see a real image with a naked eye, we should place the eye behind the image.
(2) Here, we will try to locate the image formed by the lens. This image will be formed by all the rays collected by the lens. So, if we consider the extreme rays which are being focused at the aperture (Fig. 35-45), we will get the field of view for the image. This will also show us the region in which the refracted light will be present.
Calculation : If the screen was not present, the image would have formed at
v=ufu+f=−30×20−30+20=60cm
From the lens,
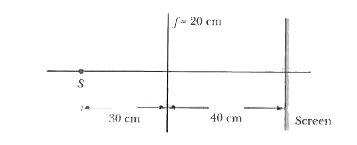
From similar triangles , we can estimate the radius of the image formed :
R3=2060
R=1cm
The amount of light used to form the image can be estimated with the help of solid angle subtened by the lens at the location of the source :
Power of image100W=1−cosθ2
Here, tanθ=3/30=0.1, for a small angle and cosθ=(1−θ2/2). The power of the image 50×(0.1)2/2=0.25W
Intensity =Powerarea
=0.25π×(0.01)2=2500πW/m2.
Learn : Here we can see how the itensity can be quite high even though the power transmitted by the lens is very small .
Topper's Solved these Questions
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Check point
6 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Problems
44 Videos
- GEOMETRICAL OPTICS : REFLECTION BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
4 Videos
Similar Questions
A glass wedge with a small angle of refraction θ is placed at a certain distance from a converging lens with a focal length f ,one surface of the wedge being perpendicular to the optical axis of the lens. A point sources S of light is on the other side of the lens at its focus. The rays reflected from the wedge (not from base) produce, after refraction in the lens , two images of the source displaced with respect to each other by d. Find the refractive index of the wedge glass. [Consider only paraxial rays.]
_E01_162_Q01.png)
View SolutionA glass wedge with a small angle of refraction θ is placed at a certain distance from a converging lens with a focal length f ,one surface of the wedge being perpendicular to the optical axis of the lens. A point sources S of light is on the other side of the lens at its focus. The rays reflected from the wedge (not from base) produce, after refraction in the lens , two images of the source displaced with respect to each other by d. Find the refractive index of the wedge glass. [Consider only paraxial rays.]
_E01_162_Q01.png)
View Solution
Knowledge Check
_S01_029_Q01.png)
In the figure shown S is a point monochromatic light source of frequency 6×1014Hz.M is a concave mirror of radius of curvature 20 cm and L is a thin converging lens of focal length 3.75 cm AB is the principal axis of M and L.
Light reflected from the mirror and refracted from the lens in succession reaches the screen. An interference pattern is obtained on the screen by this arrangement.
Q. Fringe width isA1 mmB0.5 mmC1.5 mmD0.25 mm_S01_030_Q01.png)
In the figure shown S is a point monochromatic light source of frequency 6×1014Hz.M is a concave mirror of radius of curvature 20 cm and L is a thin converging lens of focal length 3.75 cm AB is the principal axis of M and L.
Light reflected from the mirror and refracted from the lens in succession reaches the screen. An interference pattern is obtained on the screen by this arrangement.
Q. If the lens is replaced by another converging lens of focal length 103cm and the lens is shifted towards right by 2.5 cm thenAfringe width remains sameBintensity of pattern will remain sameCfringe width will changeDNo interference pattern will form.The light intensity 10 m from a point source is 1000 W/m2 . The intensity 100 m from the same source is
A1000W/m2B100W/m2C10W/m2D1W/m2
A parallel beam of light falls on the surface of a convex lens whose radius of curvature of both sides of 20cm. The refractive index of the material of the lens varies as μ=1.5+0.5r , where r is the distance of the point on the aperture from the optical centre in cm. Find the length of the region on the axis of the lens where the light will appear. The radius of aperture of the lens is 1cm.
View SolutionA parallel beam of light falls on the surface of a convex lens whose radius of curvature of both sides of 20cm. The refractive index of the material of the lens varies as μ=1.5+0.5r , where r is the distance of the point on the aperture from the optical centre in cm. Find the length of the region on the axis of the lens where the light will appear. The radius of aperture of the lens is 1cm.
View SolutionA thin convex lens of refractive index μ=1.5 is placed between a point source of Light S and a screen A, shown in figure,. Light rays from the source S are brought to focus on the screen A, forming a point image P. The distance SP is equal to 50cm. Water (μ=4/3) is now poured into a vessel interposed between the object and the lens, and it is observed that when the water level is 8cm the screen has to be moved up by a distance of 6cm in order to get a sharp image. Find the focal length of the lens.
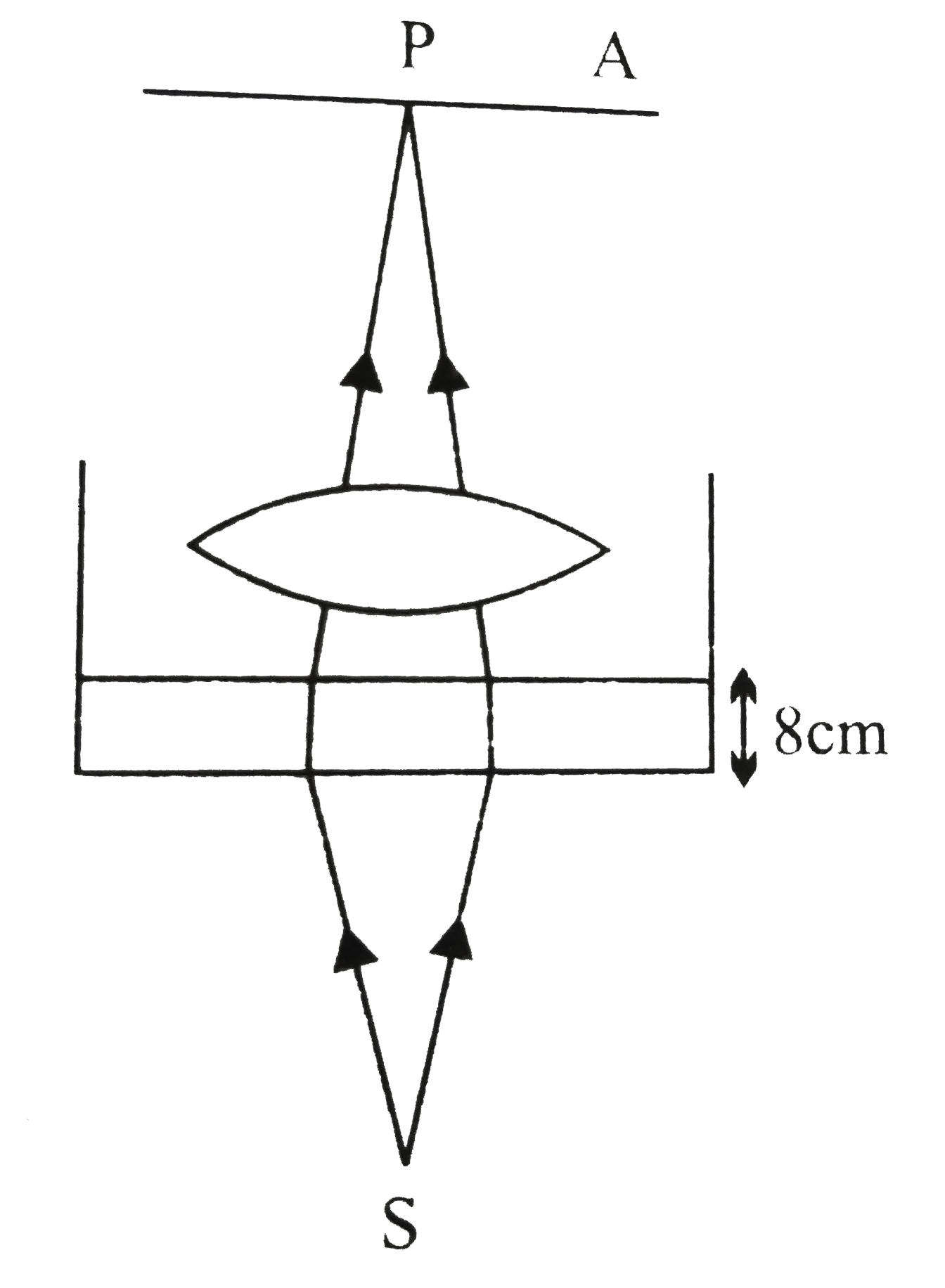
View SolutionA thin convex lens of refractive index μ=1.5 is placed between a point source of Light S and a screen A, shown in figure,. Light rays from the source S are brought to focus on the screen A, forming a point image P. The distance SP is equal to 50cm. Water (μ=4/3) is now poured into a vessel interposed between the object and the lens, and it is observed that when the water level is 8cm the screen has to be moved up by a distance of 6cm in order to get a sharp image. Find the focal length of the lens.

View Solution_S01_029_Q01.png)
In the figure shown S is a point monochromatic light source of frequency 6×1014Hz.M is a concave mirror of radius of curvature 20 cm and L is a thin converging lens of focal length 3.75 cm AB is the principal axis of M and L.
Light reflected from the mirror and refracted from the lens in succession reaches the screen. An interference pattern is obtained on the screen by this arrangement.
Q. Fringe width is
View Solution
