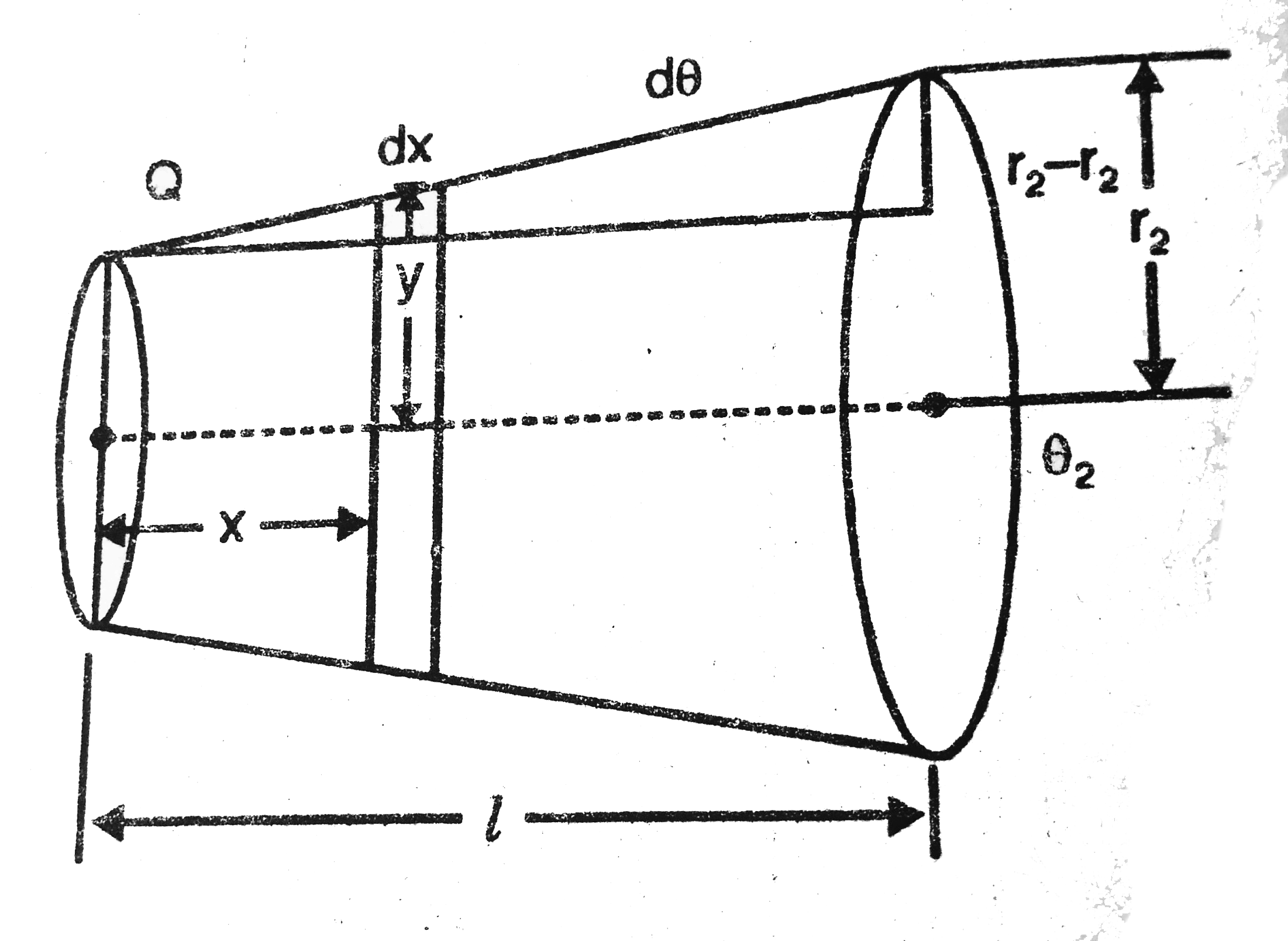
Find the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1) . Thermal conductivity of the material of the rod is K.
 Solution in Tamil
Solution in Tamil
The correct Answer is:A, B
TenΦ=r2−r1L=y−r1x
D⇔erentat∈gw,r→x<br>r_2-r_1=(Ldy)/(dx)-0<br>implies(dy)/(dx)=(r_2-r_1)/L<br>implies(r_2-r_1)/(L)dx=(dyL)/(r_2-r_1) …(i)<br>(Q/t)=(k pi y^2 d theta )/(dx)<br>Now (Qdx)/t=(k pi y^2 d theta )/(dx)<br>implies(QLdy)/(t(r-2-r_1))=(k pi y^2 Delta theta )<br>[Fromequation(i)]
⇒dθ=QLdyt(r2−r1)kπy2
Intrgrating both side
⇒∫θ2θ1dθ=QLt(r2−r1)kπ∫r1r2(dy)/(y2)
⇒(θ2−θ1)=QLt(r2−r1)kπ×[−1y]r2r1
⇒(θ2−θ1)=QLt(r2−r1)kπ×[1r1−1r2]
⇒(θ2−θ1)=QLt(r2−r1)kπ(r2−r1)r2r1
⇒(Qt)=(kπ(r2r1)θ1−θ2L.
Topper's Solved these Questions
Similar Questions
A centigrade and a Fahrenheit thermometer are dipped in boiling water. The water temperature is lowered until the Fahrenheit thermometer registered at 140°F. What is the fall in temperature as registered by the centigrade thermometer ?
View SolutionThe steam point and the ice point of a mercury thermometer are marked as 80∘C and 20∘C. What will be the temperature in centigrade mercury scale when this thermometer reads 32∘C?
View SolutionFind the dimensions of
a. linear momentum
b. frequency and
c. pressure
View SolutionFind the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionThe end of two rods of different materials with their thermal conductivities, area of cross-section and lengths all in the ratio 1:2 are maintained at the same temperature difference. If the rate of flow of heat in the first rod is 4 cal//s . Then, in the second rod rate of heat flow in cal//s will be
View SolutionA metal rod of area of cross section A has length L and coefficient of thermal conductivity K the thermal resistance of the rod is .
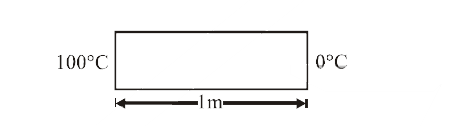
View SolutionA rod of 1m length and area of cross-section 1cm^(2) is connected across two heat reservoirs at temperature 100^(@)C and 0^(@)C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm^(-1)s^(-1)('^(@)C) ]
View SolutionAn irregular rod of same uniform material as shown in figure is conducting heat at a steady rate. The temperature gradient at various sections versus area of cross section graph will be
_S01_094_Q01.png)
View SolutionFind the thermal resistance of an aluminium rod of length 0.20 m and area of cross section 1×10−4m2. The heat current is along the length of the rod. [Thermal conductivity of aluminium=200Wm−1K−1]
View SolutionThe ends of two rods of different materials with their thermal conductivities, radii of cross sections and lengths all in the ratio 1 : 2 are maintained at the same temperature difference, if the rate of flow of heat in the larger rod is 4 cal/ sec, the rate of flow of heat in the shorter rod will be
View SolutionA rod of 1 m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperatures 100∘Cand0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod = 0.09 kilocal m−1s−1(∘C)]
View SolutionTwo different metal rods of the same length have their ends kept at the same temperature theta_(1) , and theta_(2) with theta_(2) gt theta_(1) . If A_(1) and A_(2) are their cross-sectional areas and K_(1) and K_(2) their thermal conductivities, the rate of flow of heat in the two rods will be the same it:
View SolutionThe ends of the two rods of different materials with their lengths, diameters of cross-section and thermal conductivities all in the ratio 1 : 2 are maintained at the same temperature difference. The rate of flow of heat in the shorter rod is 1cals^(-1) . What is the rate of flow of heat in the larger rod:
View Solution" A metal rod of area of cross section A has length L and coefficient of thermal conductivity K The thermal resistance of the rod is "
View SolutionFind the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionThe end of two rods of different material with their thermal conductivities, area of cross-section and lengths all in the ratio 1 : 2 are maintained at the same temperature difference. If the rate of flow of heat in the first rod is 4 cal/s. Then in the second rod rate of heat flow in cal/s is 8×p. Then p is equal to
View Solution
