Find the ratio of de-Broglie wavelengths, associated with
(i) Protons, accelerated through a potential of 128 V, and
(ii) α-particles, accelerated through a potential of 64 V.Solution in Kannada
λ=h√2meV
(i) for problem, λ1=h√2mpe×128….(1)
(ii)For α-particle , λ2=h√2mα.(2e)×64…(2)
Divide eq.(1) by eq.(2)
λ1λ2=√mα×128mp×128
=√mαmp
=√4mpmp=√4=21
Topper's Solved these Questions
- BOOK - OSWAAL PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - TOPIC-2 DE-BROGLIE RELATION (SHORT ANSWER TYPE QUESTIONS-II)2videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - OSWAAL PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - TOPIC-2 DE-BROGLIE RELATION (LONG ANSWER TYPE QUESTIONS)1videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - OSWAAL PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - TOPIC-2 DE-BROGLIE RELATION (VERY SHORT ANSWER TYPE QUESTIONS)8videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - OSWAAL PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - TOPIC-3 (POTENTIOMETER & APPLICATIONS) (NUMERICAL PROBLEMS)2videos
CURRENT ELECTRICITY
- BOOK - OSWAAL PUBLICATIONCHAPTER - ELECTRIC CHARGES & FIELDSEXERCISE - (TOPIC -2)Numerical Problems5videos
ELECTRIC CHARGES & FIELDS
Similar Questions
An electron and a photon each have a wavelength of 1.00 nm. Find (i) their momentum (ii) the energy of the photon and (iii) K.E of electron
02:46View SolutionWhat are matter waves? Derive an expression for the de Broglie wave length.
02:47View SolutionA proton and an electron have same kinetic energy. Which one has smaller de-Broglie wavelength ?
04:49View SolutionA resistance of RΩ draws current from a potentiometer. The potentiometer has a total resistance R0Ω.A voltage V is supplied to the potentiometer. Derive an expression for the voltage across R when the sliding contact is in the middle of the potentiometer.
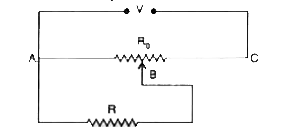 08:35View Solution
08:35View SolutionAn atom was earlier assumed to be sphere of radius a having a positively charged point nucleus of charge +Ze at its centre . This nucleus was believed to be surrounded by a uniform desity of negative charge that made the atom neutral as a whole.
Use this theorem to find the electric field of this atom at a distance r(r>a) from the centre of the atom.03:37View SolutionA proton and an α - particle are accelerated through same potential difference. Then, the ratio of de-Broglie wavelength of proton and α-particle is
03:33View SolutionA proton and and alpha - particle are accelerated through a potential difference of 100 V . The ratio of the wavelength with the proton to that associated to that associated with an alpha - particle is
02:59View SolutionAn α-particle and a proton are accelerated from rest through the same potential difference V. Find the ratio of de-Broglie wavelength associated with them.
View SolutionAn alpha -particle and a proton are accelerated from rest through the same potential difference V. Find the ratio of de Broglie wavelengths associated with them.
04:44View SolutionThe ratio between the de Broglie wavelength associated with protons, accelerated through a potential of 512 V and that of alpha particles accelerated through a potential of X volts is found to be one. Find the value of X.
04:01View SolutionA proton when accelerated through a potential difference of V, has a De-Broglie wavelength λ associated with it.if an α-particle is to have the same De-Broglie wavelength λ, it must be accelerated through a potential difference of
02:15View SolutionA proton and an α particle are accelerated through the same potential difference V. The ratio of their de Broglie wavelengths is
03:34View SolutionA protom and an alpha particle are both accelerated through a potential difference of 100 V. the ratio of the de-Broglie wavelength associated with the progom to that associated with the alpha particle is
03:47View SolutionA proton and an alpha - particle are accelerated through same potential difference. Then, the ratio of de-Broglie wavelength of proton and alpha-particle is
05:50View SolutionThe ratio of de-Broglie wavelength associated with a proton and a mu -particle accelerated through the same potential difference 'V' is
View Solution

