Finding total energy stored in a magnetic field for a length of coaxial cable from the energy density
Finding total energy stored in a magnetic field for a length of coaxial cable from the energy density
(b) What is the stored energy per unit length of the cable if a= 1.2 mm, b = 3.5 mm, and i = 2.7 A?
Video Solution
Text Solution
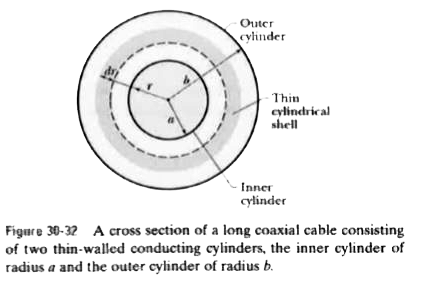
(a) Finding B: To apply these ideas, we begin with Ampere.s law, using a circular path of integration with radius r such that a < r < b (between the two cylinders, as indicated by the dashed line in Fig. 30-32). The only current enclosed by this path is current ion the inner cylinder. Thus, we can write Ampere.s law as
(30-59)
Next, we simplify the integral: Because of the circular symmetry, at all points along the circular path, is tangent to the path and has the same magnitude B. Let us take the direction of integration along the path as the direction of the magnetic field around the path. Then we can replace with B ds ds and then move magnitude B in front of the integration symbol. The integral that remains is ds, which just gives the circumference 2m- of the path. Thus, Eq. 30-59 simplifies to
or
(30-60)
Finding : Next, to obtain the energy density, we substitute Eq. 30-60 into Eq. 30-57:
(30-61)
Finding : Note that is not uniform in the volume between the two cylinders, but instead depends on the radial distance r. Thus, to find the total energy stored between the cylinders, we must integrate over that volume.
Because the volume between the cylinders has circular symmetry about the cable.s central axis, we consider the volume dV of a cylindrical shell located between the cylinders, the shell has inner radius r, outer radius r + dr (Fig. 30-32), and length l. The shell.s cross-sectional area is the product of its circumference and thickness dr. Thus, the shell.s volume dV is (dr)(l), that is, dV = dr.
Because points within this shell are all at approximately the same radial distance r, they all have approximately the same energy density . Thus, the total energy contained in the shell of volume dV is given by
energy =(energy per unit volume)(volume)
or
Substituting Eq. 30-61 for and for dV, we obtain
To find the total energy contained between the two cylinders, we integrate this equation over the volume between the two cylinders:
(30-62)
(b) From Eq. 30-62 we have
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHECKPOINTS9videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PROBLEMS67videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type1videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT EXERCISE - Practice Questions (Integer)7videos
ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT
Similar Questions
Knowledge Check
- Question 1 - Select One
Find out total energy stored in the capacitors.
A500μJB300μJC600μJD1300μJ - Question 2 - Select One
Magnetic field energy stored in a coil is
ALi2B12LiCLiD12Li2 - Question 3 - Select One
The energy density in magnetic field B is proportional to
A1BB1B2CBDB2
In the Q. No. 9. find average energy density corresponding to electric and magnetic fields.
View SolutionFind the total energy stored in the capacitors in the given network.
 View Solution
View SolutionFind the total energy stored in the capacitors in the given network of Fig.
View SolutionFind the total energy stored in capacitors in the network shown in Fig.
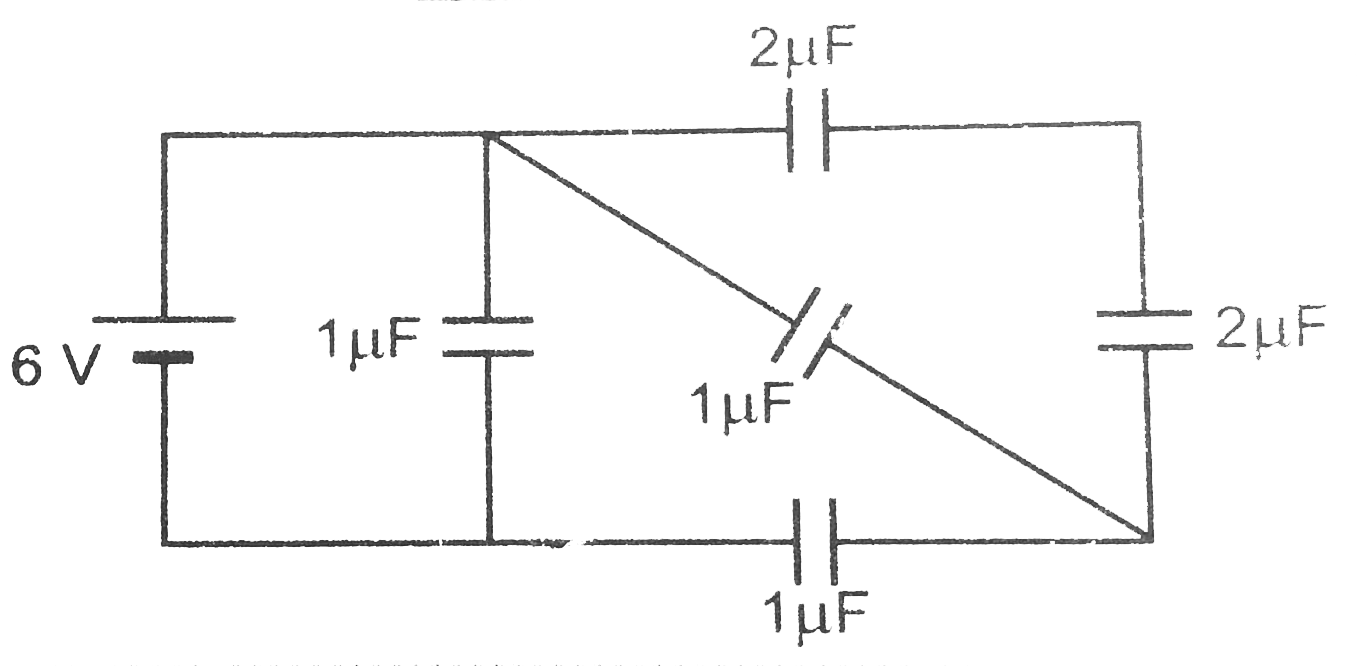 View Solution
View SolutionWhich of the following is proportional to energy density in magnetic field B:
View Solution