Following are four different relations about displacement, velocity and acceleration for the motion of a particle in general. Choose the incorrect one (s)
A
→vav=12[→v(t1)+→v(t2)]
B
→vav=(→r(t2))−→rt1t2−t1
C
→r=12(→v(t2)−→v(t1))(t2−t1)
D
→aav=→v(t2)−→v(t1)t2−t1
Video Solution
Text Solution
The correct Answer is:A, C
(a,c) : Option (a) is true omly when aceleration is constant but not is general, so it is incorrect.
Option (c) is not correct, because right hand sid is change in velocity multiplied by time interval and does not give displacement vector.
|
Topper's Solved these Questions
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - COMPETITION FILE OBJECTIVE TYPE QUESTIONS (INTEGER TYPE QUESTIONS)
10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Chapter Practise Test
16 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
Similar Questions
Knowledge Check
Which of the following diagrams correctly relate displacement velocity and acceleration with time for a particle executing SHM
A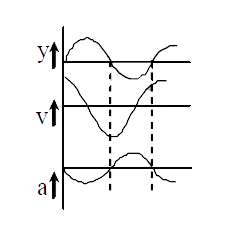 B
B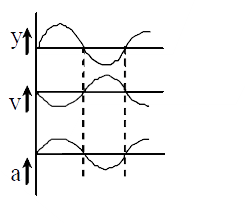 C
C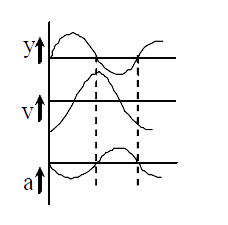 D
D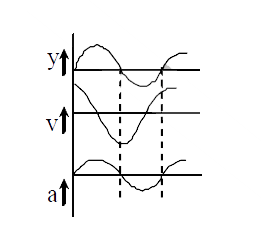
The diplacement , velocity and acceleration in a simple harmonic motion are related as the
Adisplacement , velocity and acceleration all act in the same directionbBdisplacement and velocity act in the same direction but acceleration in the opposite direction.Cvelocity and acceleration are parallel and both are perpendicular to the displacementDdisplacement and acceleration are anti-parallel and both perpendicular to the velocityThe phase difference between displacement and acceleration of a particle in a simple harmonic motion is:
A3π2 radBπ2 radCZeroDπ rad
Discuss the general relations for velocity and acceleration for motion of an one dimension in a plane.
View SolutionThe phase difference between displacement and acceleration of particle in a simple harmonic motion is
View SolutionDeduce the relations for (a) particle velocity (b) particle acceleration
View SolutionThe phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is
View SolutionThe phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is
View Solution
