For the given combination of gates, if the logic states of inputs A,B,C, are as follows A=B=C=0 and A=B=1,C=0 then the logic states of output D are

A
0, 0
B
0, 1
C
1, 0
D
1, 1
Video Solution
Text Solution
The correct Answer is:D
The output D for the given combination
D=¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯(A⋅B)⋅C=¯¯¯¯¯¯¯¯¯¯¯¯¯¯(A+B)+¯¯¯C
If A=B=C=0, then D=¯¯¯¯¯¯¯¯¯¯¯¯(0+0)=¯0=¯0+¯0=1+1=1
IfA=B=1, then D=¯¯¯¯¯¯¯¯¯¯¯¯(1+1)=¯0=¯1+¯0=0+1=1
|
Topper's Solved these Questions
- SEMICONDUCTORSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 1 ( MISCELLANEOUS PROBLEMS )
33 Videos
- SEMICONDUCTORSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SEMICONDUCTORSEXERCISE - MHT CET Corner
25 Videos
- SEMICONDUCTORSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SEMICONDUCTORSEXERCISE - MHT CET Corner
25 Videos
- SCALARS AND VECTORS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SCALARS AND VECTORS EXERCISE - Exercise 2 (Miscellaneous Problems)
20 Videos
- STATIONARY WAVES BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - STATIONARY WAVES EXERCISE - MHT CET Corner
28 Videos
Similar Questions
Knowledge Check
In the following combinations of logic gates, the outputs A, B and C are respectively
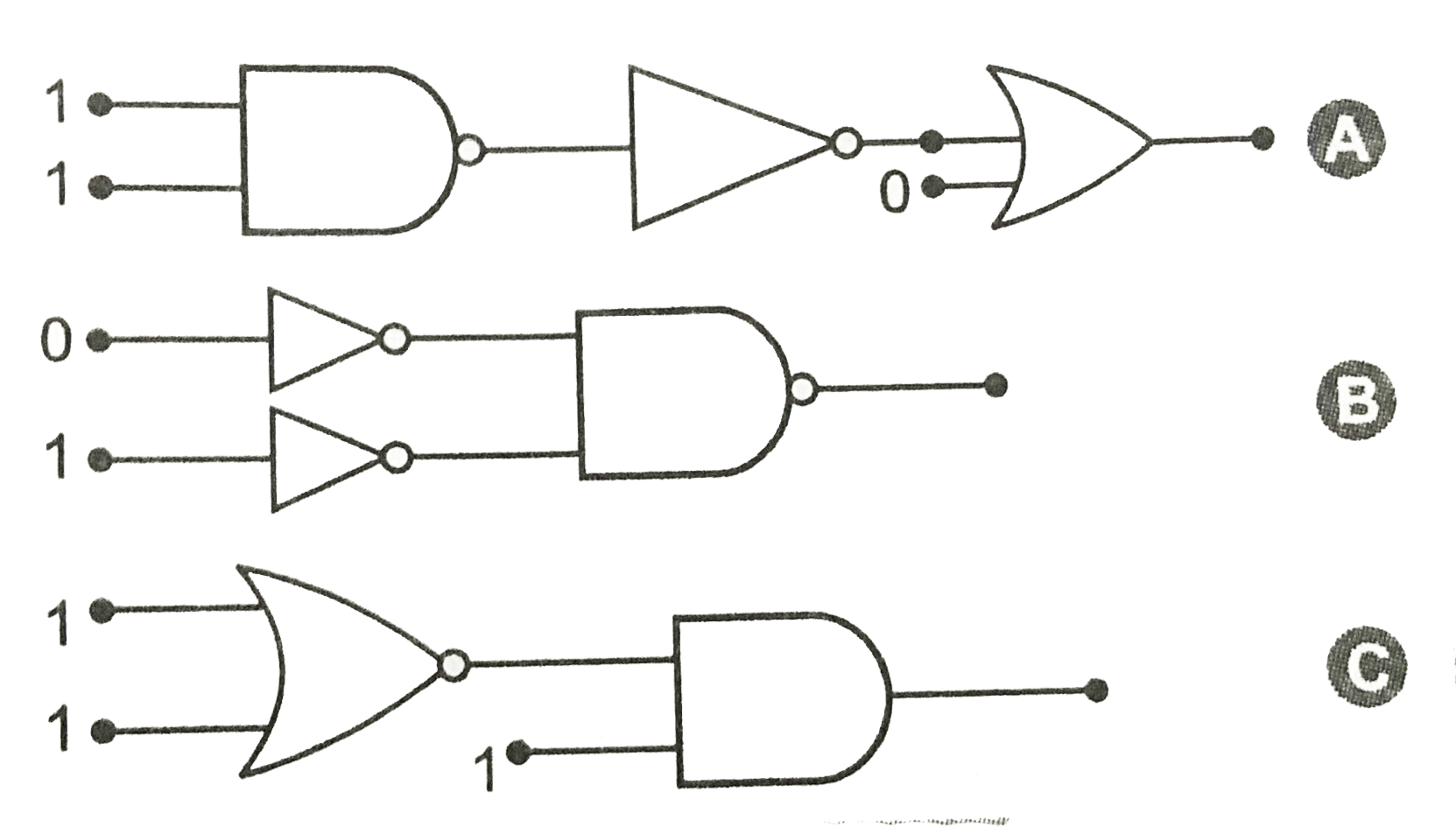 A0, 1, 1B0, 1, 0C1, 1, 0D1, 0, 1
A0, 1, 1B0, 1, 0C1, 1, 0D1, 0, 1In the following combination of logic gates, the outputs of A,B and C are respectively
(i)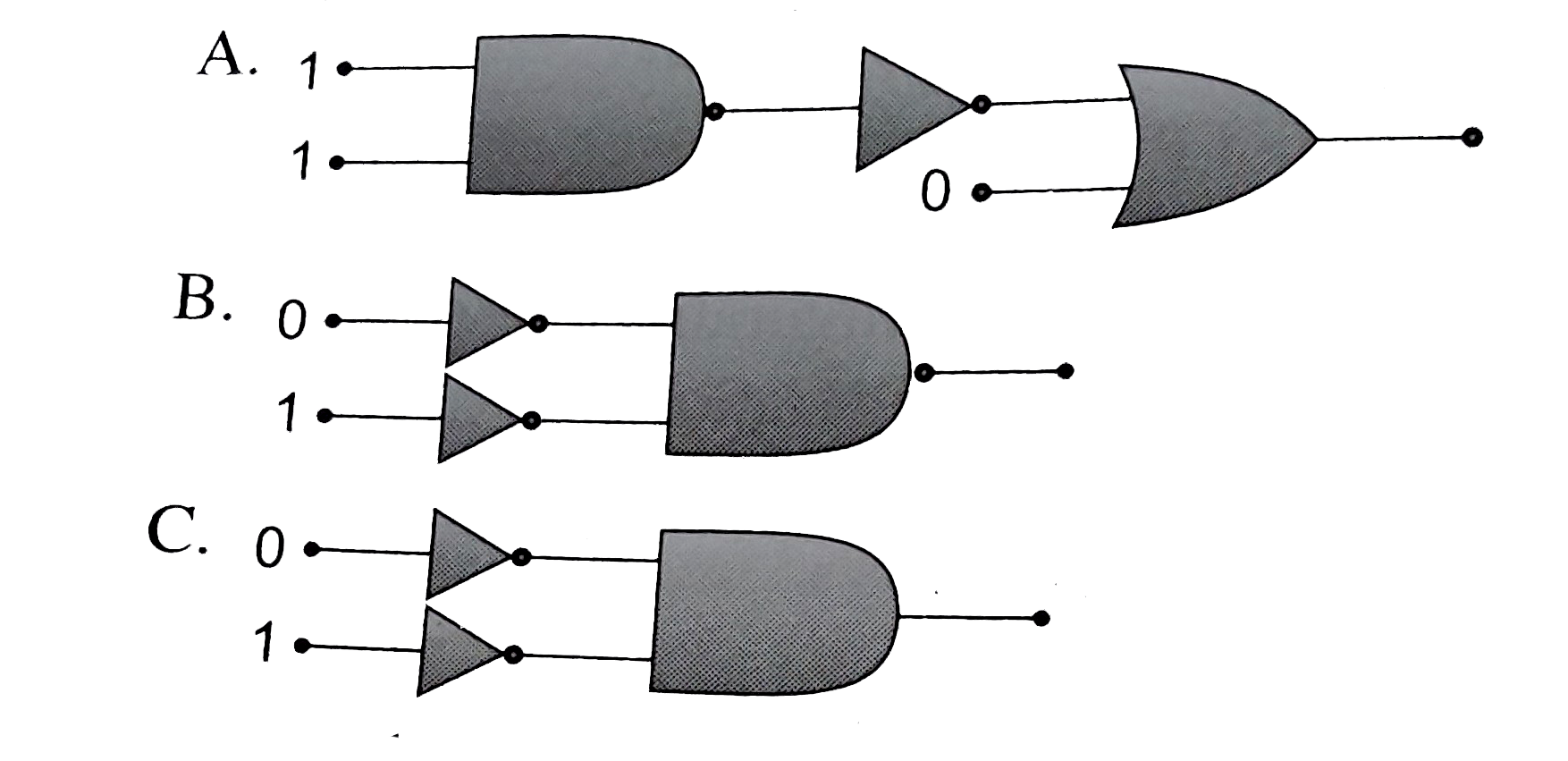 A0,1,1B0,1,0C1,1,0D1,0,1
A0,1,1B0,1,0C1,1,0D1,0,1The output of the given logic gate is 1 when inputs A,B and C are such that :-
_E01_413_Q01.png) AA=1,B=0,C=1BA=1,B=1,C=0CA=B=C=0DA=B=C=1
AA=1,B=0,C=1BA=1,B=1,C=0CA=B=C=0DA=B=C=1
In the combination of the following gates the output Y can be written in terms of inputs A and B as:
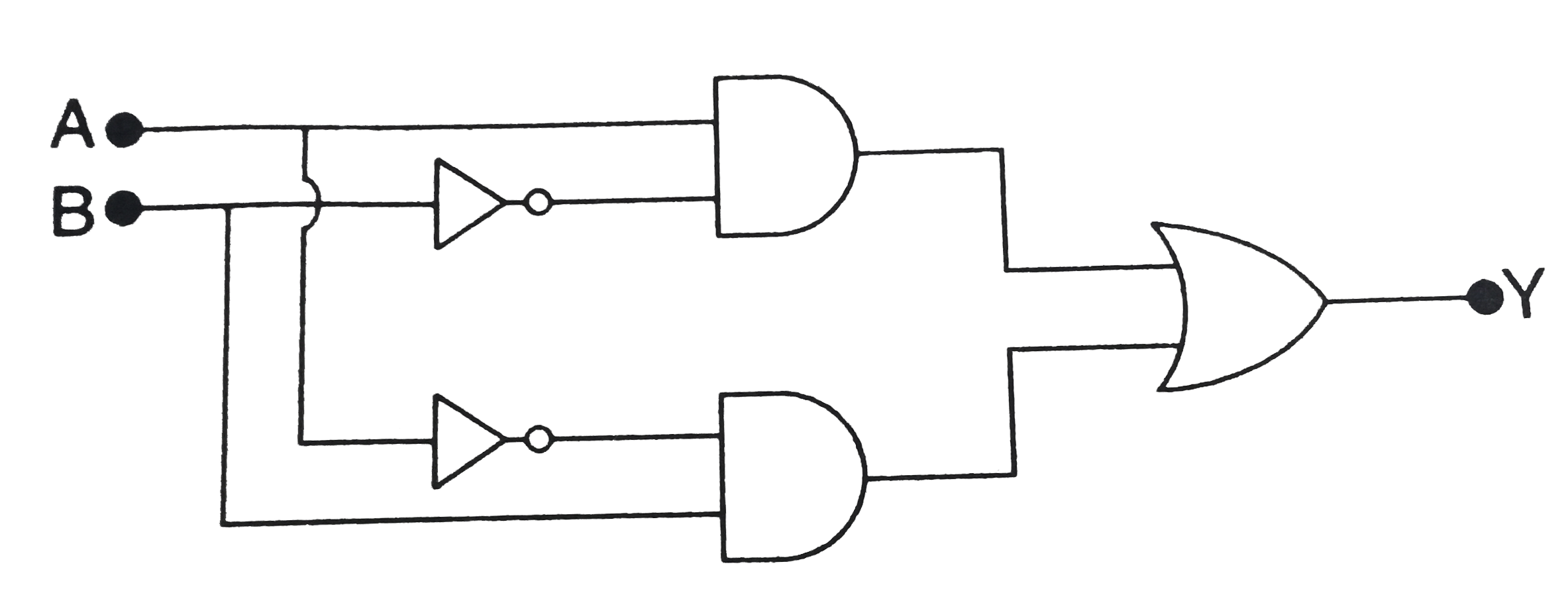
View Solutionfor the logic circuit give below , the outputs Y for A=0,B=0 and A=1,B=1 are-

View SolutionSelect the outputs Y of the combination of gates shown below for inputs A=1,B=0,A=1,B=1 and A=0,B=0 respectively :-
_E01_437_Q01.png)
View SolutionIn the combination of the following gates the output Y can be written in terms of inputs A and B as:

View SolutionIn AND gate, the output Y is 1 when the inputs A and B are:
View Solution
