Doubtnut Promotions Sticky

Fore a transverse wave on a string, the string displacement is describrd by
y(x,t)=f(x−at)
where f represent a function and a is a negative constant. Then which of the following is//are correct statement(S)?

A

the shape of the string at time t=0 is given by f(x)

B

the shape of wave form does not change as it moves along the string

C

waveform moves in +ve x-direction

D

the speed of waveform is a

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:a.,b.,d.

y(x,0)=f(x). So, shape of string at t=0 is given by y=f(x)
As velocity of wave dx/dt=+a, is constant, so shape of string does not change, or we can say (x−at) is constant. Thus, the shape of string remains the same.
As a is −ve and constant, so dx/dt=−ve and hence, wave is moving along −vex−direction.
speed =−a and not a as speed cannot be−ve

|
Updated on:21/07/2023
Class 11PHYSICSTRAVELLING WAVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    A transverse harmonic wave on a string is described by y(x, t) = 3sin ( 36t + 0.018x + π/4) where x and Y are in cm and t is in s. Which of the following statements is incorrect?

    AThe waves is travelling in negative x-direction
    BThe amplitude of the wave is 3 cm
    CThe speed of the wave is 20ms−1
    DThe frequency of the wave is9πHz.
  • Question 2 - Select One

    The transverse displacement of a string clamped at its both ends is given by
    y(x,t)=2sin(2π3x)cos(100πt)
    where x and y are in cm and t is in s.
    Which of the following statements is correct?

    AAll the points on the string between two consecutive nodes vibrate with same frequency, phase and amplitude.
    BAll the points on the string between two consecutive nodes vibrate with same frequency and phase but different amplitude.
    CAll the points on the string between two consecutive nodes vibrate with different frequency and phase but same amplitude.
    DAll the points on the string between two consecutive nodes vibrate with different frequency, phase and amplitude
  • Question 3 - Select One

    A transverse wave is travelling along a string from left to right. The figure below represents the shape of the string at a given instant. At this instant, among the following, choose the wrong statement.
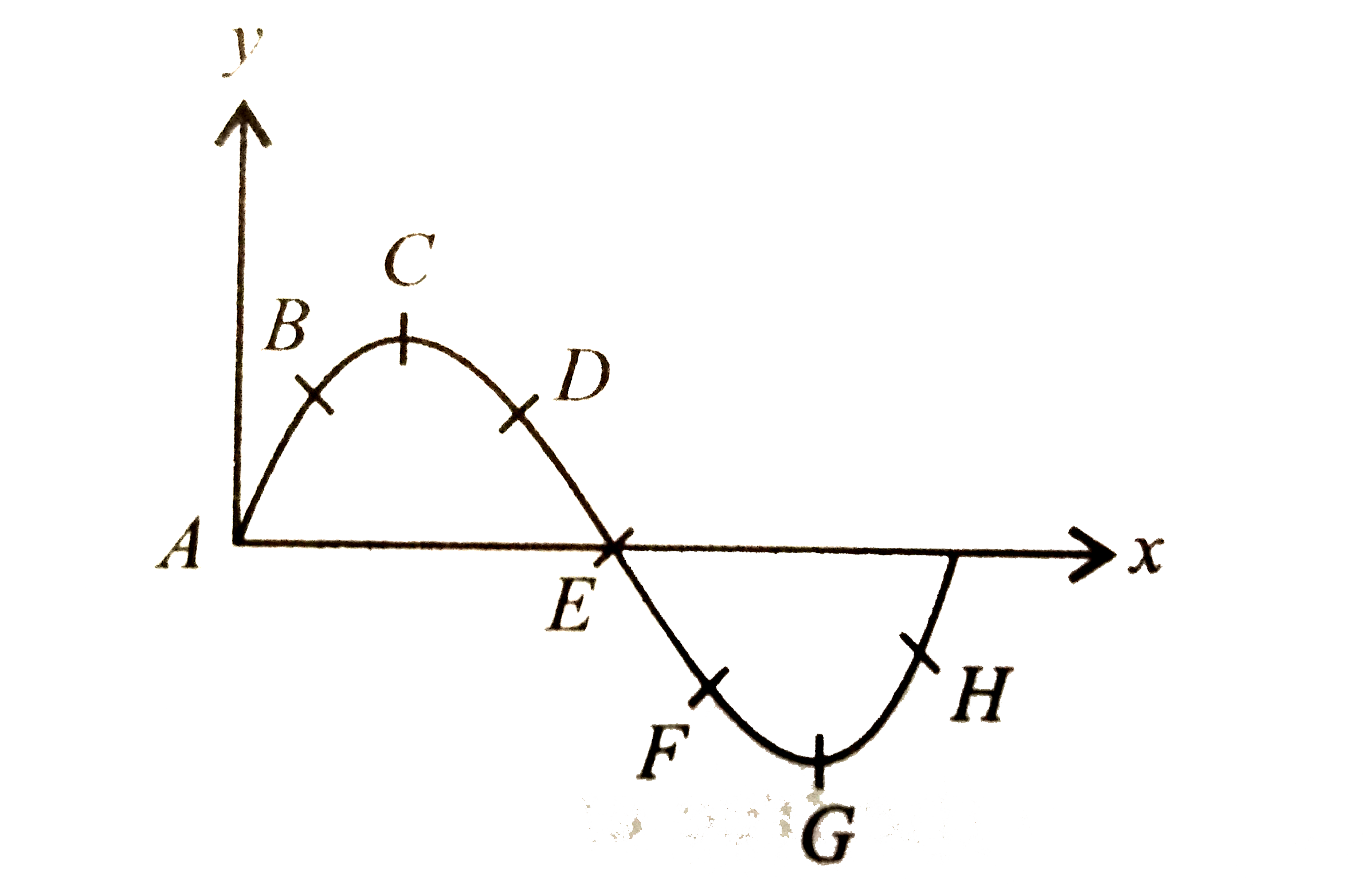    ocr_image

    APoints D, E, F have upward positive velocity.
    BPoints A, B and H have downward negative velocity
    CPoint C and G have zero velocity.
    DPoints A and E have minimum velocity.