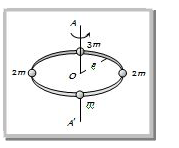
Four masses are joined to a light circular frame as shown in the figure. The radius of gyration of this system about an axis passing through the centre of the circular frame and perpendicular to its plane would be

$a / \sqrt{2}$

$a / 2$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

Radius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
AR
B $\frac{R}{2}$
C $\sqrt{2} R$
D $\frac{R}{\sqrt{2}}$
Radius of gyration of a ring about a transverse axis passing through its centre is
A $0.5 \times$ diameter of ring
Bdiameter of ring
C $2 \times$ diameter of ring
D ${(diameter of ring)}^{2}$
Four particles each of mass (M) are held rigidly by a very light circular frame of radius b. The radius of gyration of the system for an axis through the centre of the circle and perpendicular to the plane is
Ab
B2b
C $b / \sqrt{2}$
D $\sqrt{2} b$