From a disc of radius RandmassM, a circular hole of diameter R, whose rim passes through the centre is cut. What is the moment of inertia of remaining part of the disc about a perependicular axis, passing through the centre ?
13MR2/32
11MR2/32
9MR2/32
15MR2/32
The correct Answer is:A
Considering the information given in the question, let us draw the figure
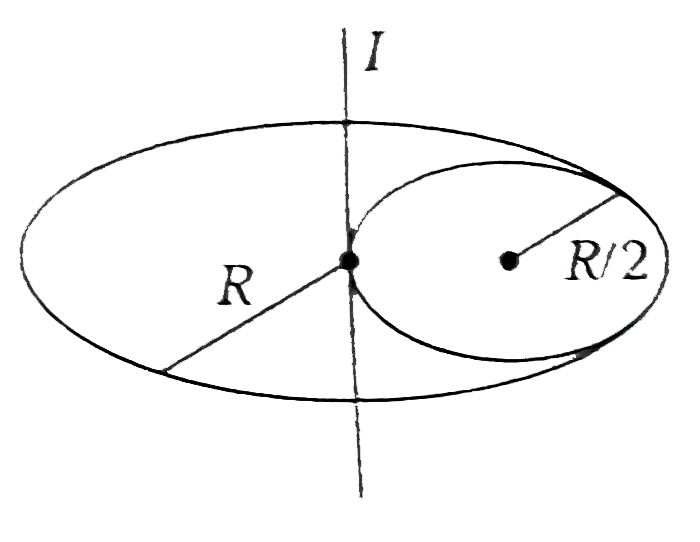
If the above figure is considered, then moment of inertia o disc will be given as I=Iremain+I(R/2)⇒Iremain=I−I(R/2) Putting the values, we get
=MR22−⎡⎢
⎢
⎢⎣M4(R2)22+M2(R2)2⎤⎥
⎥
⎥⎦
=MR22−[MR232+MR216]=MR22−[MR2+2MR232]
=MR22−3MR232=16MR2−3MR232
Iremain=13MR232.
Topper's Solved these Questions
Similar Questions
From a circular disc of radius R and mass 9M, a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View SolutionFrom a circular disc of radius R and mass 9M, a small disc of radius R/3 is removed as shown in figure. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View Solution
Knowledge Check
From a disc of radius R and mass M , a circular hole of diameter R , whose rim passes through the centre is cut . What is the moment of inertia of the remaining part of the disc about a perpendicular axis , passing through the centre ?
A11 MR2/32B9MR2/32C15MR2/32D13MR2/32From a disc of radius R and mass m, a circular hole of diamter R, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc about a perpendicular axis, passing through the centre?
A11MR2/32B9MR2/32C15MR2/32D13MR2/32From a disc of radius R and mass m, a circular hole of diamter R, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc about a perpendicular axis, passing through the centre?
A15MR2/32B13MR2/32C11MR2/32D9MR2/32
From a circular disc of radius R and mass 9 M , a small disc of mass M and radius R3 is removed concentrically . The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through its centre is
View SolutionFrom a disc of radius R, a concentric circular portion of radius r is cut out so as to leave an annular disc of mass M. The moment of inertia of this annular disc about the axis perpendicular to its plane and passing through its centre of gravity is
View SolutionFrom a circular disc of radius R and 9M , a small disc of mass M and radius R3 is removed concentrically .The moment of inertia of the remaining disc about and axis perpendicular to the plane of the disc and passing through its centre is
View SolutionMoment of inertia of a uniform circular disc about a diameter is I. Its moment of inertia about an axis perpendicular to its plane and passing through a point on its rim will be.
View SolutionThe moment of inertia of a uniform circular disc is maximum about an axis perpendicular to the disc and passing through.
 .
.
View Solution
