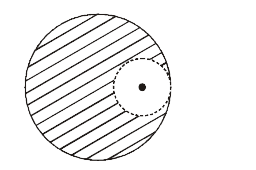
From a solid sphere of mass M and radius R, a spherical portion of radius R/2 is removed, as shown in the figure Taking gravitational potential V=0atr=∞, the potential at (G = gravitational constant)

−2GM3R
−GMR
−GM2R
−2GMR
The correct Answer is:B
M is the mass of sphere, lat ρ be the density of
sphere. So M=V×ρ=43πR3ρ
Similary if M' is the mass of cavity
M'=43π(R2)3ρ
or M'=18(43πR3ρ)=M8
Gravitational potential at a point lying inside the sphere at disatnce r from centre is
V=−GM2R3(3R2−r2)
∴VP=−GM2R3[3R2−(R2)2]
=−Gm2R3[11R24]=−11Gm8R
Gravitational potential due to cavity portion of sphere at its centre is VP'
=−3GM'2r=32G(M/8)R/2=−3GM8R
Net gravitational pot. at P
=−11GM8R−(−3GM8R)=−GMR
Similar Questions
From a solid sphere of mass M and radius R, a spherical portion of radiu R2 is removed as shown in the figure. Taking gravitational potential V = 0 at r=∞, the potential at the centre of the cavity thus formed is (G = gravitational constant)
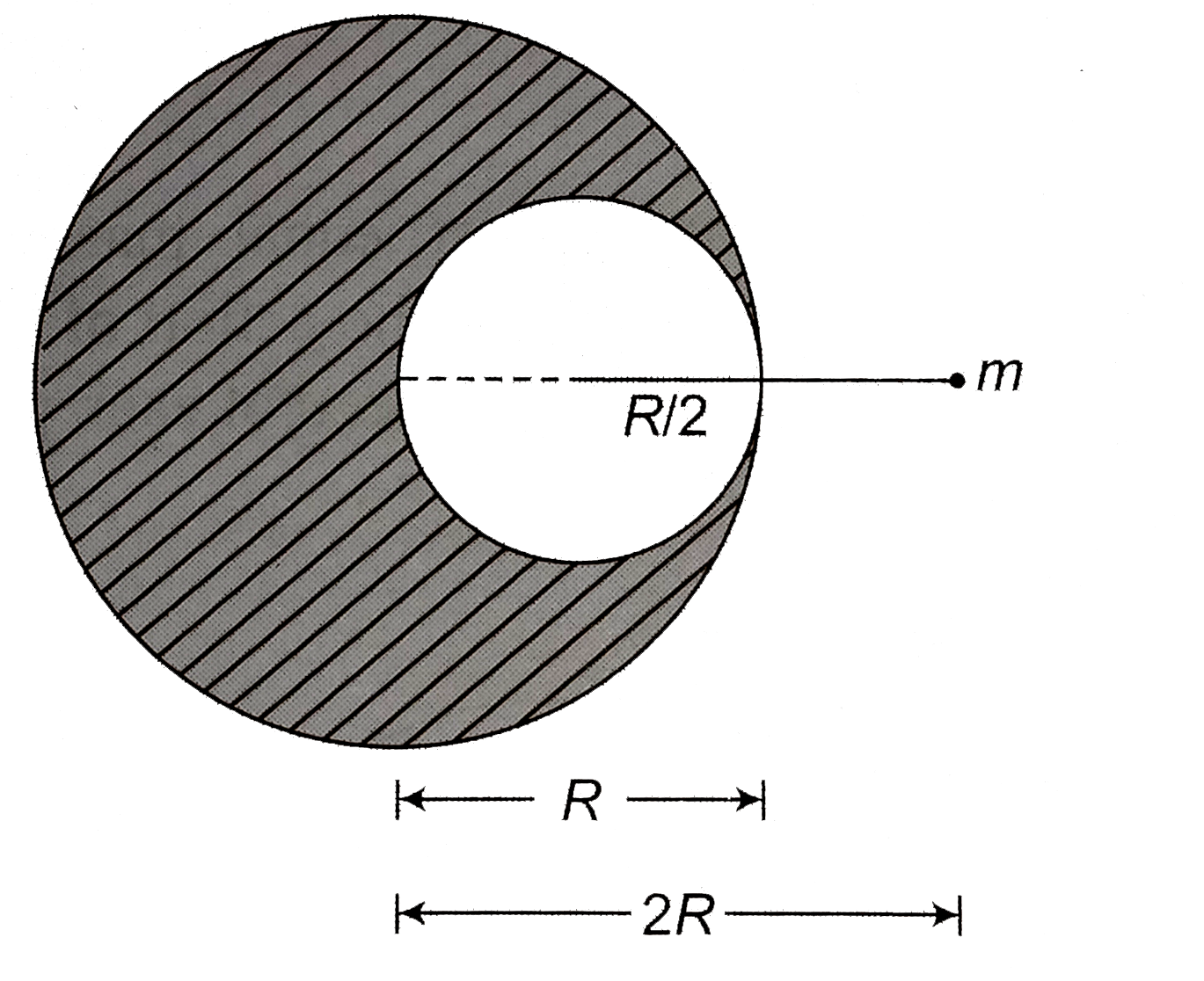
View SolutionFrom a solid sphere of mass M and radius R, a solid sphere of radius R/2 is removed as shown. Find gravitational force on mass m as shown
View Solution
Knowledge Check
From a solid sphere of mass M and radius R, a spherical portion of radius R/2 is removed, as shown in the figure Taking gravitational potential V=0atr=∞, the potential at (G = gravitational constatn)
 A−2GM3RB−2GMRC−GM2RD−GMR
A−2GM3RB−2GMRC−GM2RD−GMRA shell of mass M and radius R has point mass m placed at a distance r from its centre. The gravitational potential energy U(r) vs r will be
A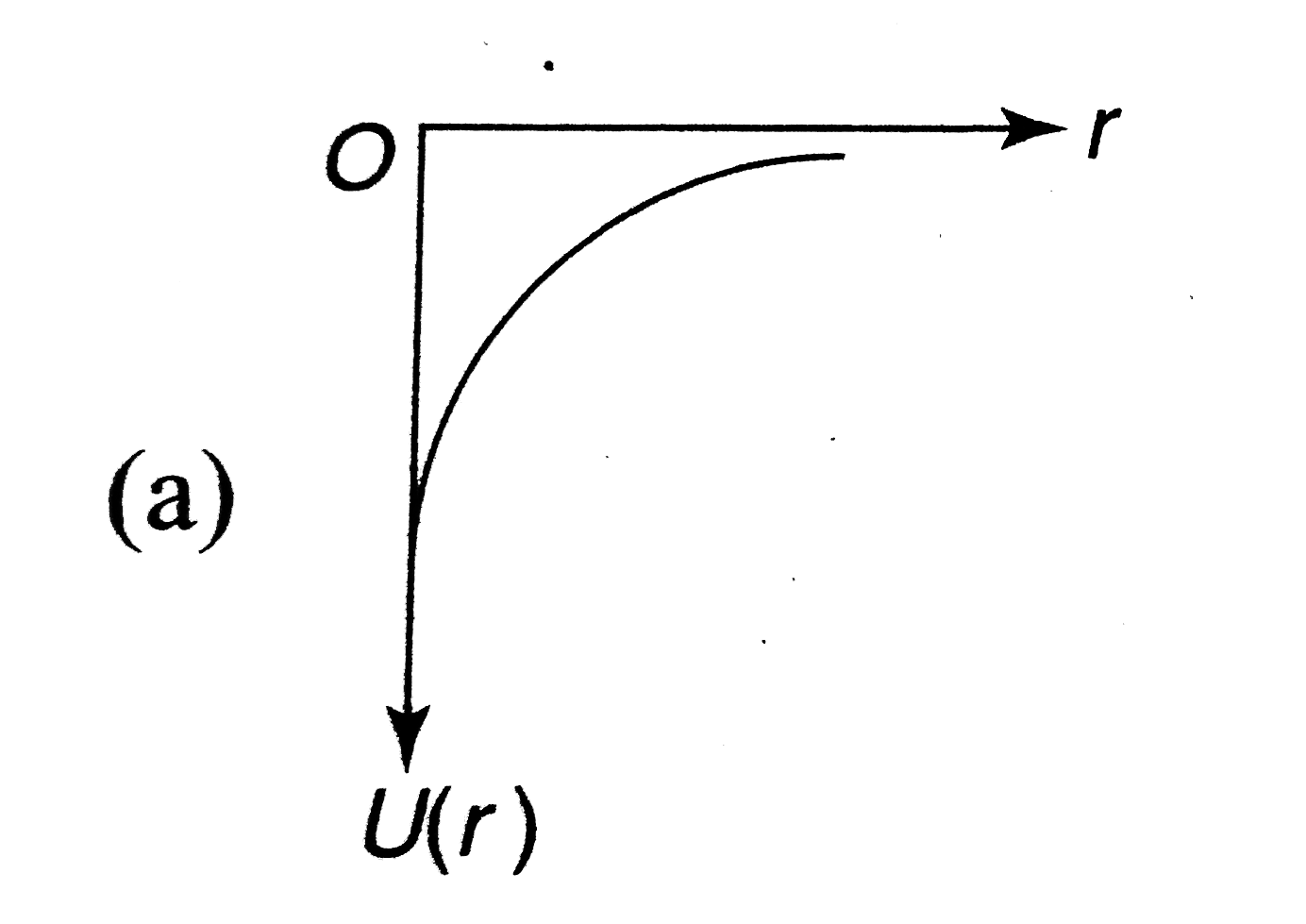 B
B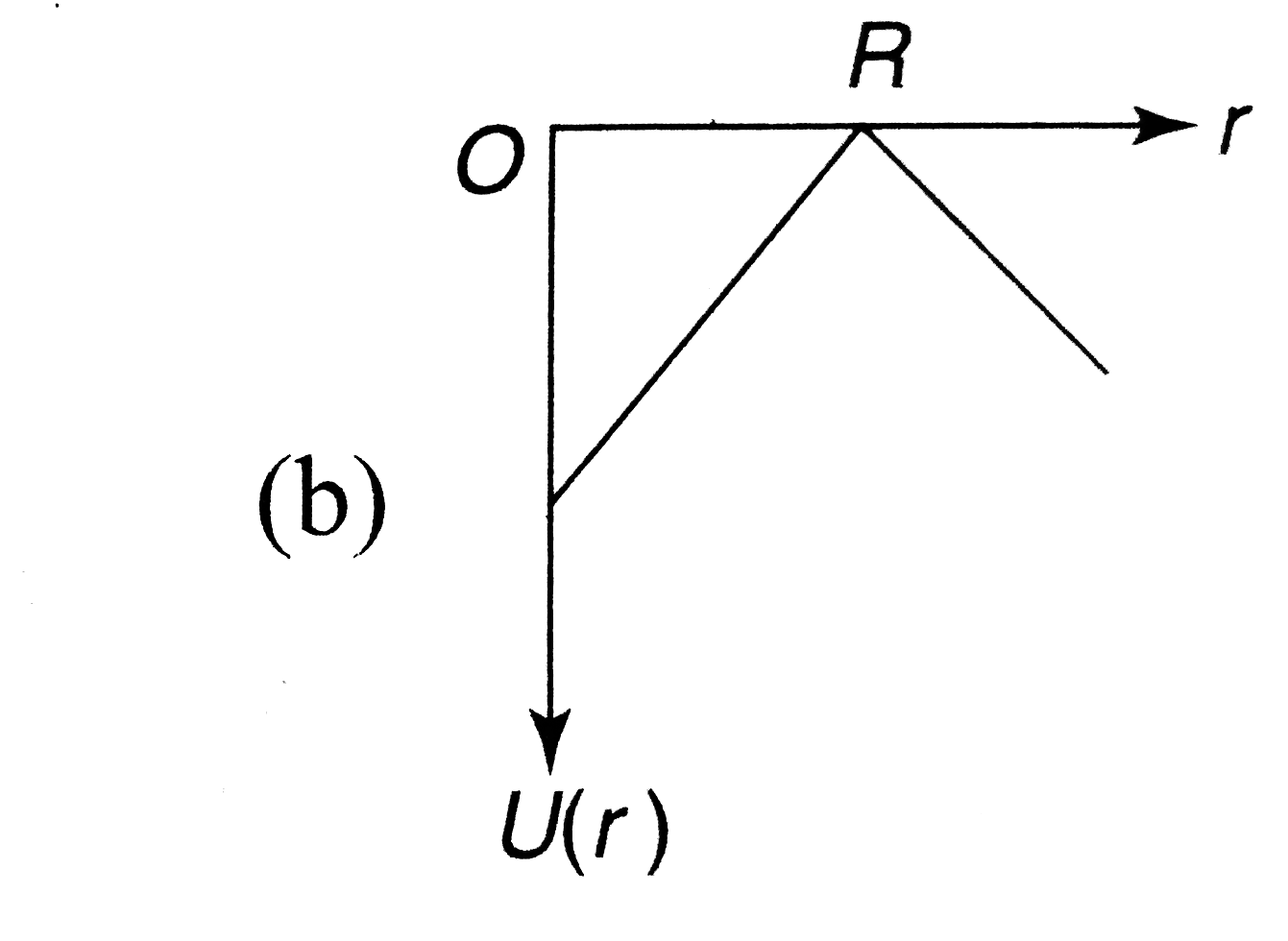 C
C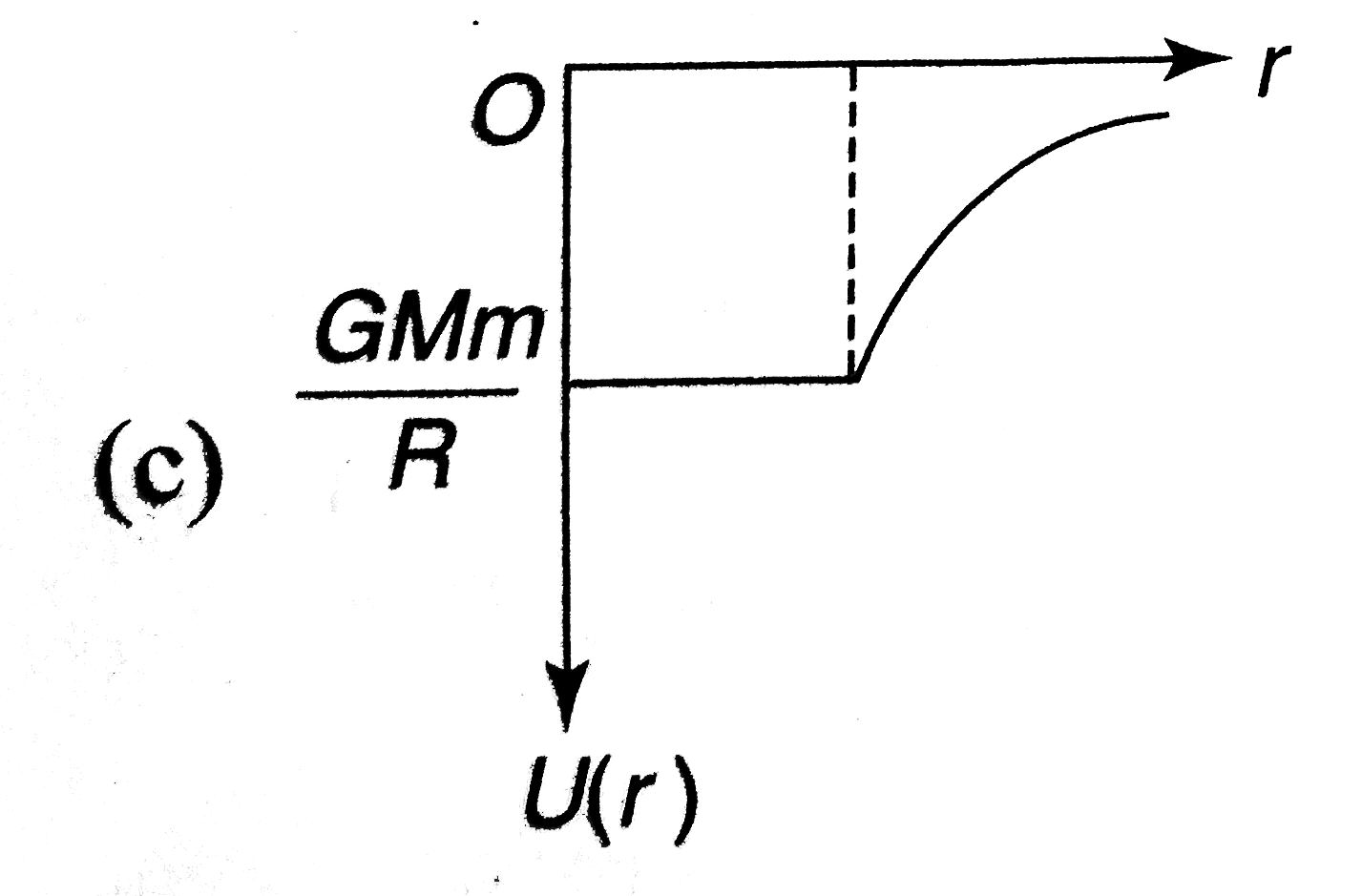 D
D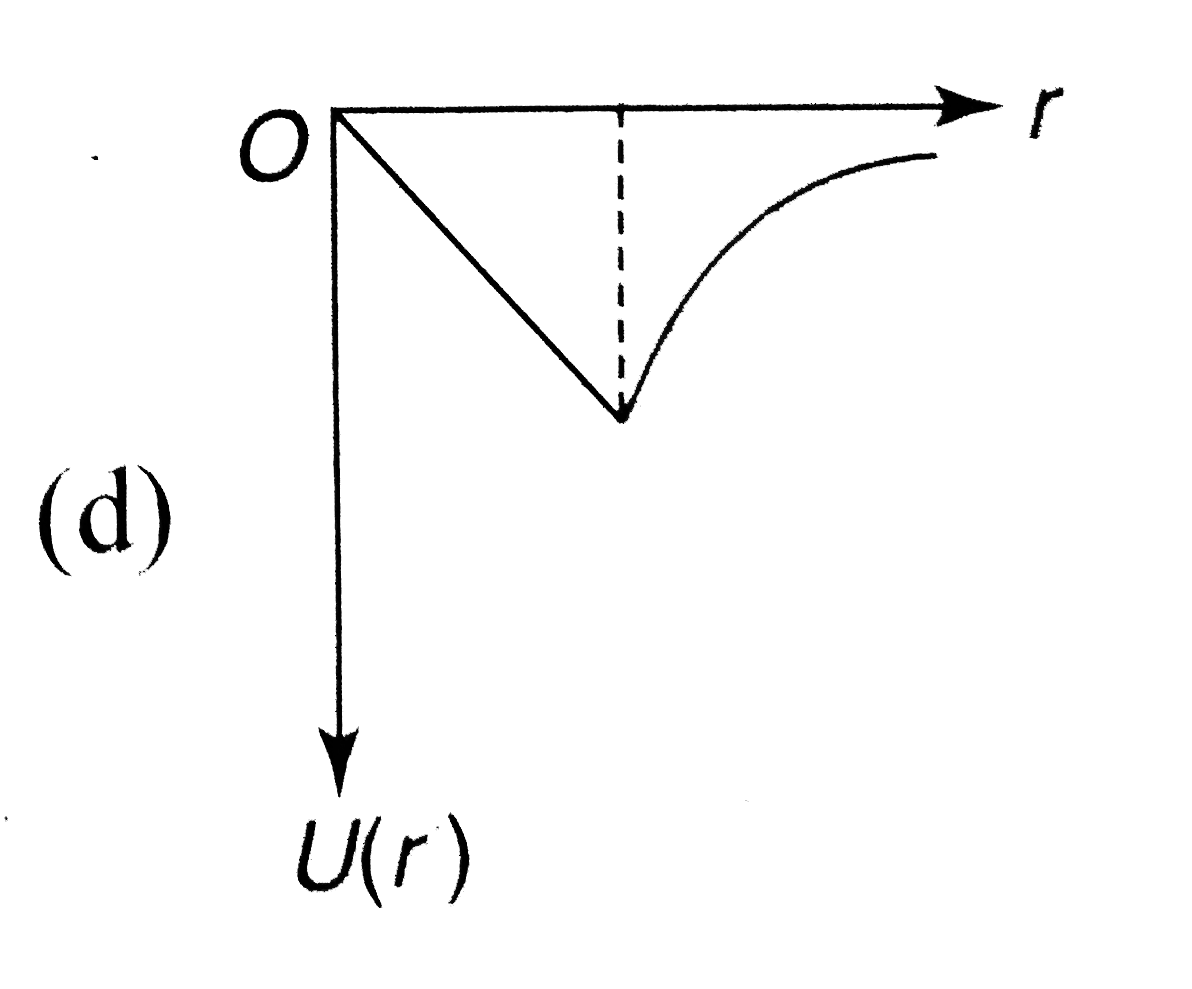
A hemi-spherical cavity is created in solid sphere (of radius 2R) as shown in the figure. Then
 Axcm=−R/15Bycm=−R40Czcm=zeroDNone of these
Axcm=−R/15Bycm=−R40Czcm=zeroDNone of these
A particle of mass m is placed at a distance of 4R from the centre of a huge uniform sphere of mass M and radius R. A spherical cavity of diameter R is made in the sphere as shown in the figure. If the gravitational interaction potential energy of the system of mass m and the remaining sphere after making the cavity is λGMm28R. Find the value of λ
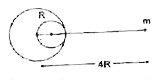
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionThree solid spheres of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionThree solid sphere of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionThe figure represents a solid uniform sphere of mass M and radius R. A spherical cavity of radius r is at a distance a from the centre of the sphere. The gravitational field inside the cavity is

View Solution
