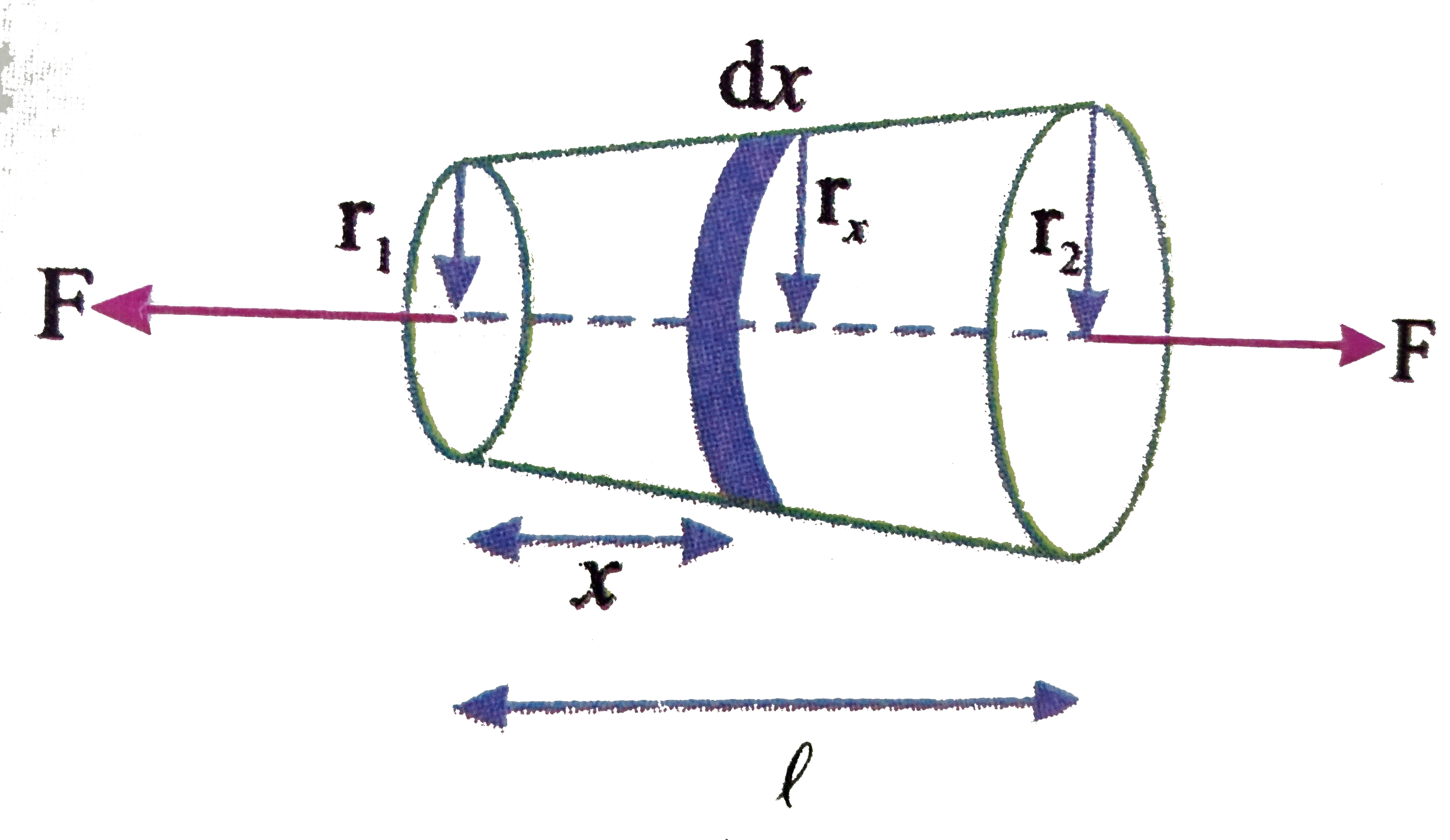
If a conical wire is streatched by two forces F applied parallel to its length and in opposite direction . Normal to end faces . The length of wire is L and its end radius are $r_{1}$ and $r_{2}$ . Find out the extension produced .
(Given , Y= Young's moleulus of wire )

A

$\frac{F L}{π r_{1}^{2} Y}$

B

$\frac{F L}{π r_{1} Y}$

C

$\frac{F L}{π r_{1} r^{2} Y}$

D

$\frac{F L}{π r_{1} r^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

(c) $Δ L = \frac{F L}{Y A} = \frac{F L}{Y \sqrt{A_{1} A_{2}}} = \frac{F L}{Y \sqrt{π r_{1}^{2} π r_{2}^{2}}}, Δ L = \frac{F L}{Y π r_{1} r_{2}}$

|

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The young's modulus of a wire of length (L) and radius (r ) is Y. If the length is reduced to $\frac{L}{2}$ and radius $\frac{r}{2}$ , then its young's modulus will be
A $\frac{Y}{2}$
B $Y$
C $2 Y$
D $4 Y$
Question 2 - Select One
Two wires of the same length $l$ and radius are joined end to end and loaded. If the Young's moduli of the materials of the wires are $Y_{1}$ and $Y_{2}$ , the combination behaves as a single wire of Young's modulus will be
A $(Y_{1} + Y_{2})$
B $\sqrt{Y_{1} Y_{2}}$
C $\frac{2 Y_{1} Y_{2}}{(Y_{1} + Y_{2})}$
D $\frac{Y_{1} Y_{2}}{(Y_{1} + Y_{2})}$
Question 3 - Select One
The young's modulus of a wire of length (L) and radius (r ) is Y. If the length is reduced to $\frac{L}{2}$ and radius $\frac{r}{2}$ , then its young's modulus will be
AY/2
BY
C2Y
D4Y

BITSAT GUIDE-ELASTICITY-BITSAT ARCHIVES

Home
Ask Doubt
Profile