If a spring extends by x on loading, then the energy stored by the spring is (if T is tension in the spring and k is spring constant)
T22x
T22k
2xT2
2T2k
The correct Answer is:B
To find the energy stored in a spring when it extends by x under a load, we can follow these steps:
1. Understand the Energy Stored in a Spring:
The energy (U) stored in a spring when it is extended by a distance x is given by the formula:
\(
U = \frac{1}{2} k x^2
\)
where k is the spring constant.
2. Relate Tension and Spring Constant:
When a spring is stretched, the tension (T) in the spring is related to the spring constant (k) and the extension (x) by Hooke's Law:
\(
T = kx
\)
From this, we can express x in terms of T and k:
\(
x = \frac{T}{k}
\)
3. Substitute x in the Energy Formula:
Now, substitute the expression for x into the energy formula:
\(
U = \frac{1}{2} k \left(\frac{T}{k}\right)^2
\)
4. Simplify the Expression:
Simplifying the equation:
\(
U = \frac{1}{2} k \cdot \frac{T^2}{k^2}
\)
\(
U = \frac{1}{2} \cdot \frac{T^2}{k}
\)
5. Final Expression for Energy Stored:
Thus, the energy stored in the spring can be expressed as:
\(
U = \frac{T^2}{2k}
\)
Conclusion:
The energy stored by the spring when it extends by x under a load T is:
\(
U = \frac{T^2}{2k}
\)
The energy (U) stored in a spring when it is extended by a distance x is given by the formula:
\(
U = \frac{1}{2} k x^2
\)
where k is the spring constant.
When a spring is stretched, the tension (T) in the spring is related to the spring constant (k) and the extension (x) by Hooke's Law:
\(
T = kx
\)
From this, we can express x in terms of T and k:
\(
x = \frac{T}{k}
\)
Now, substitute the expression for x into the energy formula:
\(
U = \frac{1}{2} k \left(\frac{T}{k}\right)^2
\)
Simplifying the equation:
\(
U = \frac{1}{2} k \cdot \frac{T^2}{k^2}
\)
\(
U = \frac{1}{2} \cdot \frac{T^2}{k}
\)
Thus, the energy stored in the spring can be expressed as:
\(
U = \frac{T^2}{2k}
\)
The energy stored by the spring when it extends by x under a load T is:
\(
U = \frac{T^2}{2k}
\)
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - NEET Questions17videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test29videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Chapter Test29videos
ROTATIONAL DYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
If a spring extends by x on loading, then then energy stored by the spring is (if T is tension in the spring and k is spring constant)
AT22xBT22kC2xT2D2T2k - Question 2 - Select One
If a spring extends by x on loading, then the energy stored by the spring is (if T is tension in the spring and k is spring constant)
AT22kBT22k2C2kT2D2T2k - Question 3 - Select One
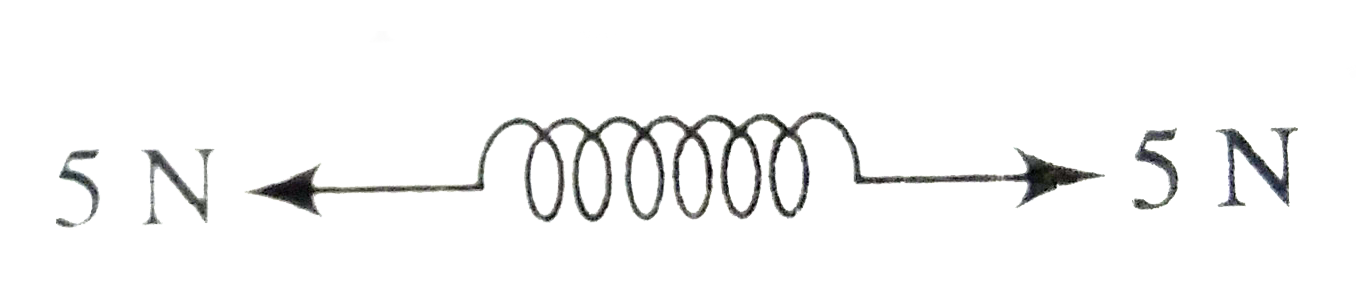
The tension is the spring isAzeroB2.5 NC5 ND10 N
The tension in the spring is.
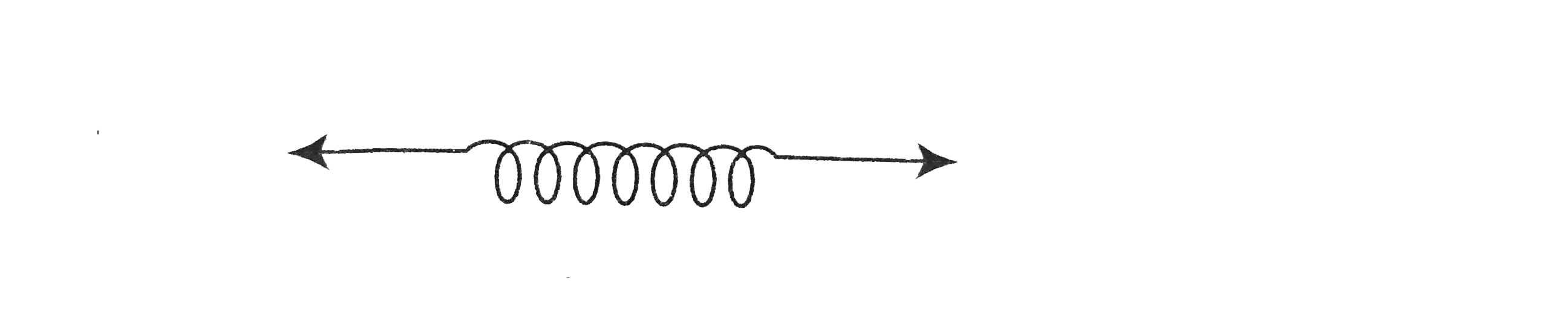 View Solution
View SolutionThe energy stored in wound watch spring is
View SolutionForce constants K1andK2 of two springs are in the ratio 5:4. They are stretched by same length. If potential energy stored in one spring is 25 J then potential energy stored in second spring is
View SolutionWhen a long spring is stretched by 1 cm, the potential energy stored in the spring is U. What will be the potential energy stored in it if it is stretched by 4 cm ?
View SolutionIn a winding (spring) watch, the energy is stored in the form of :
View Solution

