If the intensity of an X-ray becomes I02 from I0 after travelling 2.0 cm inside a target, then its intesity after travelling a distance of 4 cm will be
I02
I04
2I0
I0
The correct Answer is:B
To solve the problem, we need to determine the intensity of the X-ray after it has traveled a distance of 4 cm, given that its intensity reduces from I0 to I02 after traveling 2 cm.
1. Understand the relationship between intensity and distance:
The intensity of X-rays as they travel through a material can be described by the equation:
I=I0e−μx
where:
- I is the intensity after traveling distance x,
- I0 is the initial intensity,
- μ is the material's attenuation coefficient,
- x is the distance traveled.
2. Set up the equation for the first distance (2 cm):
We know that after traveling 2 cm, the intensity becomes I02. Plugging into the equation:
I02=I0e−μ⋅2
Dividing both sides by I0 (assuming I0≠0):
12=e−2μ
3. Take the natural logarithm:
To solve for μ, take the natural logarithm of both sides:
ln(12)=−2μ
This simplifies to:
−ln(2)=−2μ⇒μ=ln(2)2
4. Set up the equation for the second distance (4 cm):
Now, we want to find the intensity after traveling 4 cm:
I=I0e−μ⋅4
Substituting μ=ln(2)2:
I=I0e−4⋅ln(2)2=I0e−2ln(2)
5. Simplify using properties of exponents:
Recall that eln(ab)=ab:
I=I0(eln(2))−2=I0(12)2
This results in:
I=I0⋅14=I04
6. Conclusion:
Therefore, the intensity of the X-ray after traveling 4 cm is:
I04
The intensity of X-rays as they travel through a material can be described by the equation:
I=I0e−μx
where:
- I is the intensity after traveling distance x,
- I0 is the initial intensity,
- μ is the material's attenuation coefficient,
- x is the distance traveled.
We know that after traveling 2 cm, the intensity becomes I02. Plugging into the equation:
I02=I0e−μ⋅2
Dividing both sides by I0 (assuming I0≠0):
12=e−2μ
To solve for μ, take the natural logarithm of both sides:
ln(12)=−2μ
This simplifies to:
−ln(2)=−2μ⇒μ=ln(2)2
Now, we want to find the intensity after traveling 4 cm:
I=I0e−μ⋅4
Substituting μ=ln(2)2:
I=I0e−4⋅ln(2)2=I0e−2ln(2)
Recall that eln(ab)=ab:
I=I0(eln(2))−2=I0(12)2
This results in:
I=I0⋅14=I04
Therefore, the intensity of the X-ray after traveling 4 cm is:
I04
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - ASSERTION AND REASON10videos
ATOMS
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MATCH THE COLUMNS4videos
ATOMS
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - Check point 12.315videos
ATOMS
- BOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN62videos
ALTERNATING CURRENT
- BOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
Similar Questions
If the intensity of an X-ray becomes I03 from I0 after travelling 3.5cm inside a target then its intensity after travelling next 7 cm will be
View SolutionFigure shows a string of linear mass density 1.0gcm−1 on which a wave pulse is travelling. Find the time taken by pulse in travelling through a distance of 50cm on the string. Take g=10ms−2
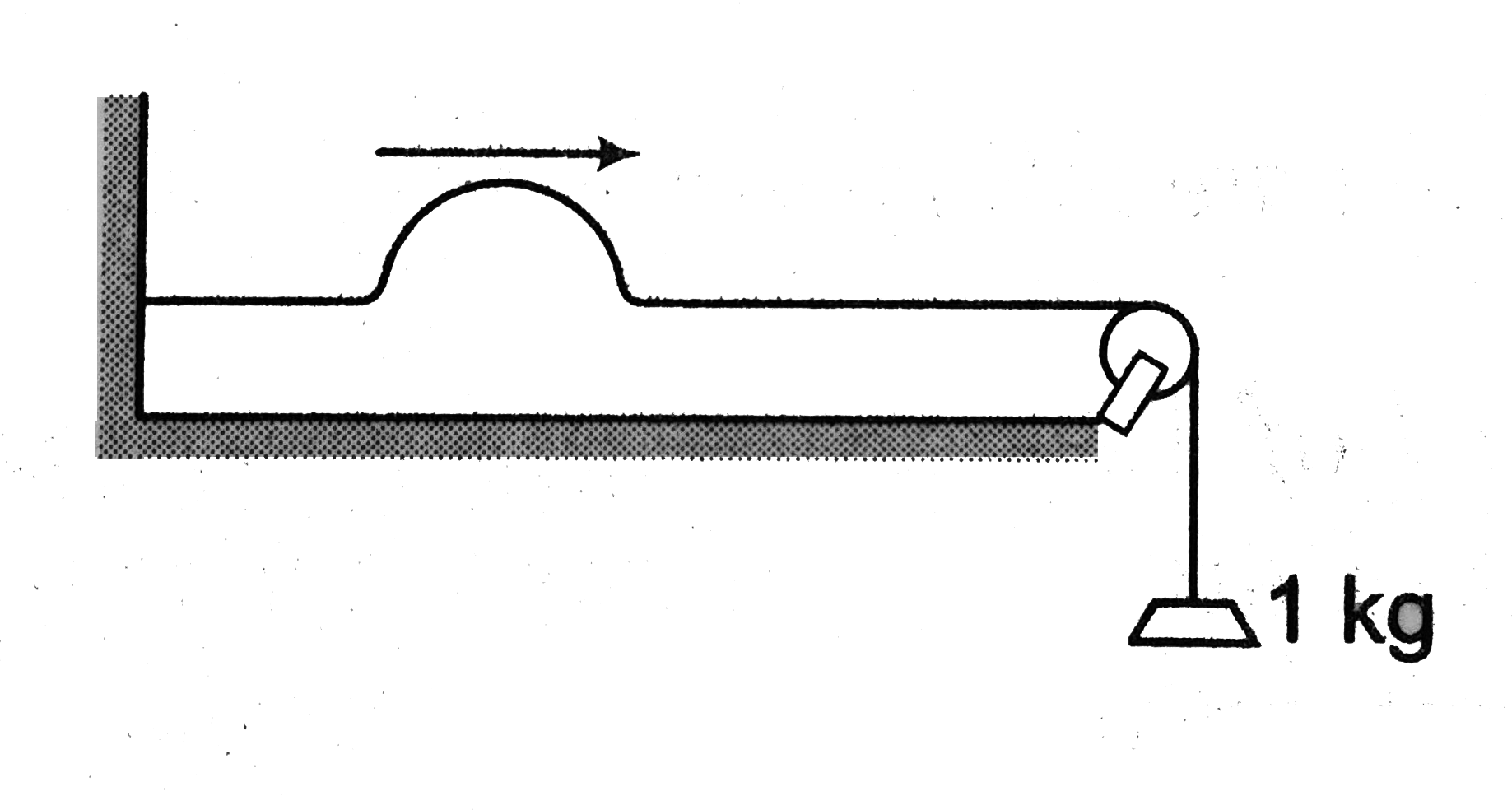 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In Young's double slit experiment, the slits are horizontal. The intensity at a point P as shown in figure is 34I0,whereI0 is the maximum intensity.
Then the value of θ is,
(Given the distance between the two slits S1 and S2 is 2λ)
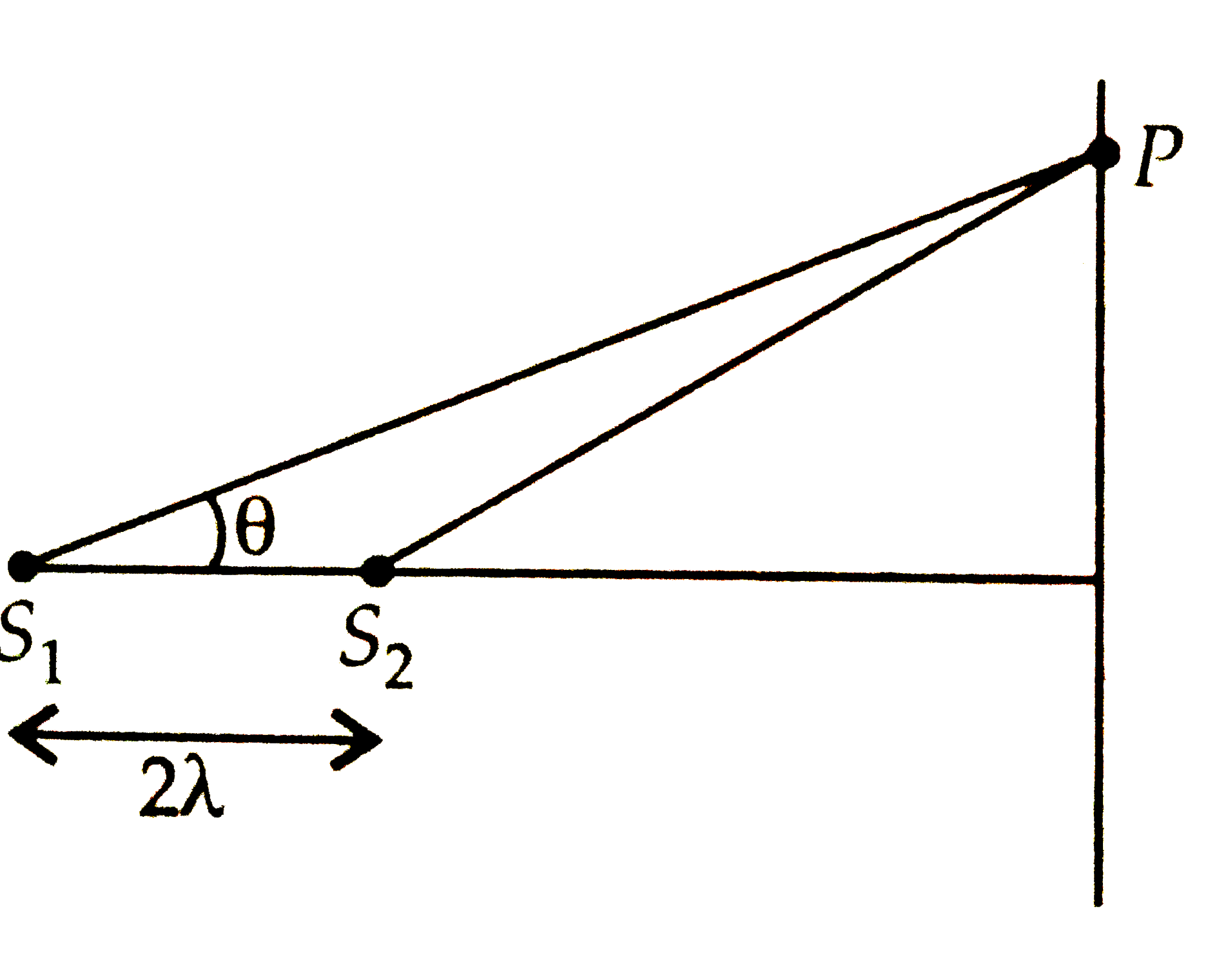 Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35)
Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35) - Question 2 - Select One
The intensity of a light pulse travelling along a communication channel decreases exponetially with distance x according to the relation I=I0e−ax where I0 is the intensity at x=0 and α is the attenuation constant. The percentage decrease in intensity after a distance of (In4α) is
A0.7B0.75C0.8D0.85 - Question 3 - Select One
(i) The intensity of a light pulse travelling along a communication channel decreases exponentially with distance x according to the relation I=I0e−αx, where I0 is the intensity at x=0 and α is the attenuation constant.
Show that the intensity reduces by 75 percent after a distance of ln4α
(ii) Attenuation of a signal can be expressed in decibel (dB) according to the relation
dB =10log10(I/I0). What is the attenuation in dB/km for an optical fibre in which the intensity falls by 50 percent over a distance of 50 km?A0.7B0.75C0.8D0.85
if the intensity of light is made 4I0, then the saturation current will become
View SolutionA ray of light enters a rectangular glass slab of refractive index √3 at an angle of incidence 60∘. It travels a distance of 5cm inside the slab and emerges out of the slab. The perpendicular distance between the incident and the emergent rays is
View SolutionThe intensity of a light pulse travelling along an optical fibre decreases exponentially with distance according to the relation l=i0e−0.0693x where x is in km and l0 is intensity of incident pulse. The intensity of pulse reduces to 14 after travelling a distance
View SolutionAn intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
The speed of light in the medium isView SolutionAn intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
As the beam enters the medium, it willView Solution

