If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
1.22 cm
2.44 cm
3.12 cm
3.86 cm
The correct Answer is:B
To solve the problem of finding the capillary rise in a tube of diameter 1 mm with a surface tension of water of 0.06 N/m, we can follow these steps:
Step 1: Identify the given values
- Surface tension (T) = 0.06 N/m
- Diameter of the tube (d) = 1 mm = 1 x 10^-3 m
- Radius of the tube (R) = d/2 = (1 x 10^-3 m) / 2 = 0.5 x 10^-3 m = 0.0005 m
- Angle (θ) = 0° (cos 0° = 1)
- Density of water (ρ) = 1000 kg/m³ (or 10^3 kg/m³)
- Acceleration due to gravity (g) = 10 m/s² (approximately)
Step 2: Use the formula for capillary rise
The formula for the height of capillary rise (h) is given by:
h=2TcosθρRg
Step 3: Substitute the values into the formula
Substituting the known values into the formula:
h=2×0.06N/m×cos(0°)1000kg/m3×0.0005m×10m/s2
Since cos(0°)=1, we can simplify this to:
h=2×0.061000×0.0005×10
Step 4: Calculate the denominator
Calculating the denominator:
1000×0.0005×10=5
Step 5: Calculate the height (h)
Now substituting back into the equation for h:
h=2×0.065
Calculating this gives:
h=0.125=0.024m
Step 6: Convert to centimeters
To convert meters to centimeters:
h=0.024m×100cm/m=2.4cm
Final Result
Thus, the capillary rise in the tube is 2.4 cm.
---
- Surface tension (T) = 0.06 N/m
- Diameter of the tube (d) = 1 mm = 1 x 10^-3 m
- Radius of the tube (R) = d/2 = (1 x 10^-3 m) / 2 = 0.5 x 10^-3 m = 0.0005 m
- Angle (θ) = 0° (cos 0° = 1)
- Density of water (ρ) = 1000 kg/m³ (or 10^3 kg/m³)
- Acceleration due to gravity (g) = 10 m/s² (approximately)
The formula for the height of capillary rise (h) is given by:
Substituting the known values into the formula:
Calculating the denominator:
Now substituting back into the equation for h:
To convert meters to centimeters:
Thus, the capillary rise in the tube is 2.4 cm.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Higher Order Thinking Skills
8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Exemplar Problems
5 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Surface tension of water is 0.072 Nm−1. The excess pressure inside a water drop of diameter 1.2 mm is :-
View SolutionA glass rod of radius 1mm is inserted symmetrically into a glass capillary tube with inside radius 2mm. Then the whole arrangement is brought in contact of the surface of water. Surface tension of water is 7×10−2N/m. To what height will the water rise in the capillary? (θ=0∘)
View Solution
Knowledge Check
Surface tension of mercury is 0.465Nm−1. The excess pressure inside a mercury drop of diameter 6mm is
A310 paB410 paC510 paD610 pa
A long capillary tube of radius 0.2mm is placed vertically inside a beaker of water.
If the surface tension of water is 7.2×10−2N/m the angle of contact between glass and water is zero, then determine the height of the water column in the tube.
View SolutionA vertical capillary is brought in contact with the water surface (surface tension = T). The radius of the capillary is r and the contact angle θ=0∘. The increase in potential energy of the water (density =ρ) is
View SolutionFigure shows a capillary tube of radius r dipped into The atmospheric pressure is P0 and the capillary rise of water is h. s is the surface tension for water-glass.
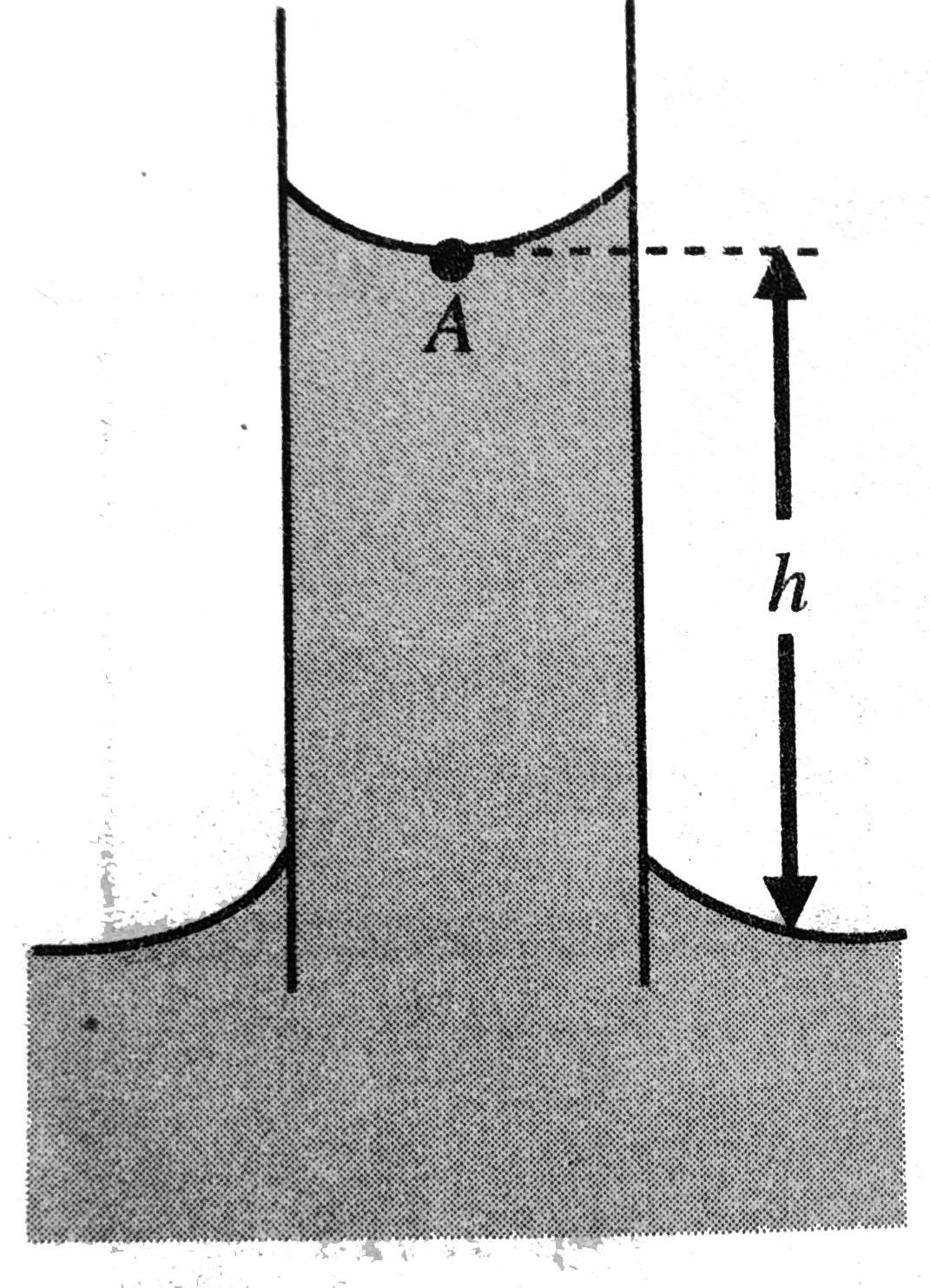
Initially, h=10cm. If the capillary tube is now incline at 45∘, the length of water rising in the tube will be
View SolutionA glass U-tube is such that the diameter of one limb is 3.0mm and that of the other is 6.0mm. The tube is inverted vertically with the open ends below the surface of water in a beaker. What is the difference between the height to which water rises in the two limbs? Surface tension of water is 0.07Nm−1. Assume that the angle of contact between water and glass is 0∘.
View SolutionThe following observations were taken for dtermining the surface tension of water by capillary tube method: diameter of capillary , D=1.25×10−2m and rise of water in capillary , h=1.45×10−2m. Taking g=9.80ms−2 and using the relation T=(rgh/2)×103Nm−1, what is the possible error in measurement of surface tension T?
(a) 2.4% (b) 15% (c) 1.6% (d) 0.15%
View SolutionThe following observations were taken for dtermining the surface tension of water by capillary tube method: diameter of capillary , D=1.25×10−2m and rise of water in capillary , h=1.45×10−2m. Taking g=9.80ms−2 and using the relation T=(rgh/2)×103Nm−1, what is the possible error in measurement of surface tension T?
(a) 2.4% (b) 15% (c) 1.6% (d) 0.15%
View SolutionWater rises in a capillary tube to a certain height such that the upward force due to surface tension is balanced by 75×10−4 newton force due to the weight of the liquid. If the surface tension of water is 6×10^-2 newton/metre the inner circumference of the capillary must be:
View Solution
