If the water pressure gauge shows the pressure at ground floor to be 270k Pa, how high would water rise in the pipes of a building?
The correct Answer is:(27.6m)
To solve the problem, we need to determine how high water would rise in the pipes of a building when the pressure gauge shows a pressure of 270 kPa. We will use the hydrostatic pressure formula, which relates the pressure at a certain depth in a fluid to the height of the fluid column above that point.
1. Understand the Given Pressure:
The pressure given by the water gauge is P=270kPa.
Convert this pressure into pascals:
P=270×103Pa=270000Pa
2. Use the Hydrostatic Pressure Formula:
The hydrostatic pressure formula is given by:
P=ρgh
where:
- P is the pressure (in pascals),
- ρ is the density of the fluid (for water, ρ≈1000kg/m3),
- g is the acceleration due to gravity (approximately 9.81m/s2),
- h is the height of the water column (in meters).
3. Rearrange the Formula to Solve for Height h:
Rearranging the formula to find h:
h=Pρg
4. Substitute the Known Values:
Substitute P=270000Pa, ρ=1000kg/m3, and g=9.81m/s2 into the equation:
h=2700001000×9.81
5. Calculate the Height h:
h=2700009810≈27.5m
6. Conclusion:
Therefore, the height to which water would rise in the pipes of the building is approximately 27.5m.
The pressure given by the water gauge is P=270kPa.
Convert this pressure into pascals:
P=270×103Pa=270000Pa
The hydrostatic pressure formula is given by:
P=ρgh
where:
- P is the pressure (in pascals),
- ρ is the density of the fluid (for water, ρ≈1000kg/m3),
- g is the acceleration due to gravity (approximately 9.81m/s2),
- h is the height of the water column (in meters).
Rearranging the formula to find h:
h=Pρg
Substitute P=270000Pa, ρ=1000kg/m3, and g=9.81m/s2 into the equation:
h=2700001000×9.81
h=2700009810≈27.5m
Therefore, the height to which water would rise in the pipes of the building is approximately 27.5m.
Topper's Solved these Questions
Similar Questions
How high would the water column have been in Q. 3?
View SolutionA water pipe with internal diameter of 1 inch carries water at ground floor of a house with velocity 3 ft/ sec and at pressrue 25 lb/inch.2. Another pipe of of internal diameter 1/2 inch is connected to it and takes water to the first floor 25 feet above ground. what is the velocity and water pressure at first floor ?
View Solution
Knowledge Check
Where would the pressure of sea water be maximum?
ABelow 100 metres from the surface.BBelow 80 metres from the surface.CBelow 105 metres from the surface.DBelow 10 metres from the surface.The reading of a pressure gauge attached to a closed horizontal pipe was 3.5×105 Pa. When the valve of the pipe was opened , the pressure was reduced to 3×105 Pa. What was the speed of water flowing out of the pipe ?
A2.5 m/sB5 m/sC7.5 m/sD10 m/sFigure shows a crude type of atomizer. is compressed, air flows swiftly through tube BC causing a reduced pressure in the particles of the vertical to Liquid rises in the tube, enters BC and is sprayed out. if the pressure in the bulb is Pa+P, where P is the gauge pressure and Pa is the atmospheric pressure, v is the speed of air in BC, find how large would v need to be to cause the liquid to rise to BC. Density of air =1.3kg/m3.
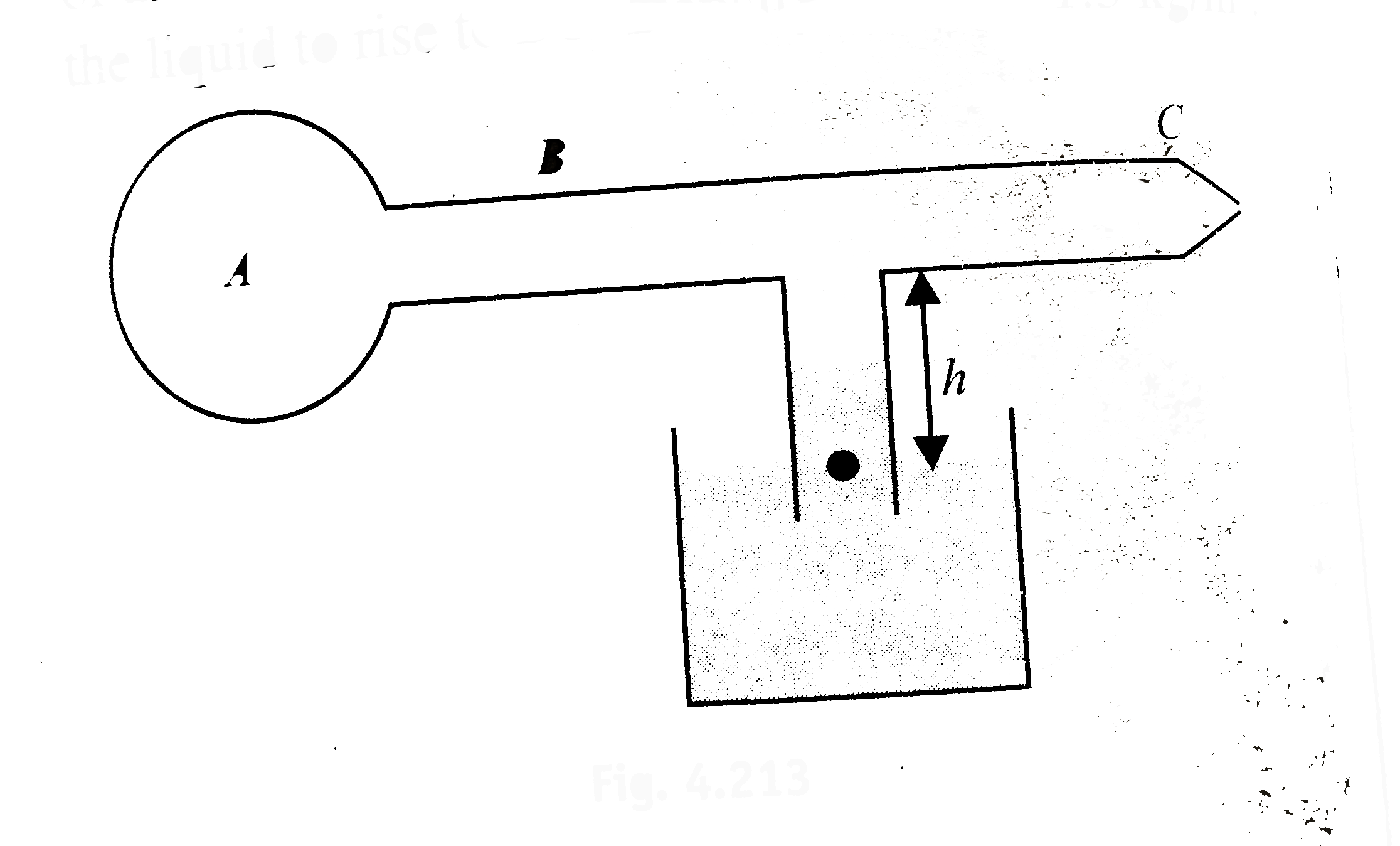 AP+ρgh√0.65BPhρg√0.650CP√hρg0.65DPhρg√0.65
AP+ρgh√0.65BPhρg√0.650CP√hρg0.65DPhρg√0.65
A cylinder containing cooking gas can withstand a pressure of 15atm. The pressure gauge of the cylinder indicates 12atm at 27∘C. Due to a sudden fire in the building, the temperature starts rising. At what temperature will the cylinder explode?
View SolutionThree flasks are filled with coloured water as shown here. How does the water pressure at the bottom of each flask compare with the water pressure at the bottom of the other two?
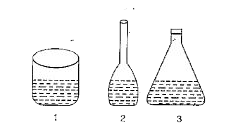
View Solution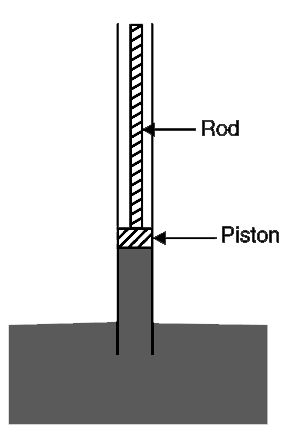
A tightly fitted piston can slide along the inner wall of a long cylindrical pipe. With the piston at the lower end of the pipe, the lower end of the pipe is dipped into a large tank, filled with water. Now the piston is pulled up with the help of the rod attached to it. water rises in the pipe along with the piston. Why? To what maximum height water can be raised in the pipe using this method? What will be the answer to your question if water is replaced with mercuty? Atmosphere pressure is Patm=1.01×105Pa.
View SolutionIf the gauge pressure, at the bottom of a water tank is 2.7 kPa, what is the height of the water [Take g = 10 m s−2]
Hint : Gauge Pressure = absolute pressure - atmospheric pressure = pgh
View SolutionWater flows in a horizontal pipe whose one end is closed with a value and the pressure gauge falls to 1×105N/m2 when the value is opend. Calculate the speed of water flowing in the pipe.
View Solution
