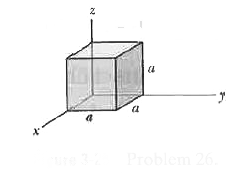
In a cube of edge length a sits with one corncr at the origin of an xyz coordinate system. A body diagonal is a line that extends from one corner to another through the center. In unit-vector , what is the body diagonal that extends from the corner at (a) corrdianates (b) corrdinates (a,0,0) (c ) coordinates (0,a,0) and (d) coordinates (a,a,0)? (e) Determine the angles that the body diagonals make with the adjacent edges . (f) Determine the length of the boedy diagonals in terms of a.
Text Solution
|
Topper's Solved these Questions
- VECTORS BOOK - RESNICK AND HALLIDAYCHAPTER - VECTORS EXERCISE - PRACTICE QUESTIONS
39 Videos
- VECTORS BOOK - RESNICK AND HALLIDAYCHAPTER - VECTORS EXERCISE - CHECKPOINT
5 Videos
- UNITS AND MEASUREMENT BOOK - RESNICK AND HALLIDAYCHAPTER - UNITS AND MEASUREMENT EXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)
11 Videos
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
5 Videos
Similar Questions
Knowledge Check
The point equidistant from the O(0,0,0),A(a,0,0),B(0,b,0) and C(0,0,c) has the coordinates
A(a,b,c)B(a/2,b/2,c/2)C(a/3,b/3,c/3)D(a/4,b/4,c/4)What are coordinates of the point equidistant from the points (a,0,0), (0,a,0),(0,0,a) and (0,0,0)?
A(a3,a3,a3)B(a2,a2,a2)C(a,a,a)D(2a,2a,2a)
Find the coordinates of a point equidistant from the origin and pints A(a,0,0), B(0,b,0)and C(0,0,c).
View SolutionFind the coordinates of the point equidistant from the four points A(0,0,0) , B (a,0,0) , C(0,b,0) and D(0,0,c) .
View SolutionFind the coordinates of a point equidistant from the four points O(0,0,0), A(p,0,0), B(0,q,0) and C(0,0,r)
View SolutionFind the coordinates of a point equidistant from th four points O(0,0,0),A(l,0,0),B(0,m,0)andC(0,0,n).
View SolutionFind the coordinates of a point equidistant from th four points O(0,0,0),A(a,0,0),B(0,b,0)andC(0,0,c).
View SolutionFind the coordinates of a point equidistant from the four points O(0,0,0),A(ℓ,0,0),B(0,m,0) and C(0,0,n).
View Solution
