In a experiment , N identical electrical bulbs, each having resistance R , are connecteed in parallel to a dc source of emf E and internalresistance r . What is the power consumed by each bulb . Also find the percentage change in power by each bulb if one bulb turns out.
The correct Answer is:E2R((Nr+R))2 , 200rNr+R
To solve the problem step by step, we will break it down into two parts: finding the power consumed by each bulb when N identical bulbs are connected in parallel, and then finding the percentage change in power when one bulb turns off.
Step 1: Calculate the Equivalent Resistance
When N identical bulbs, each with resistance R, are connected in parallel, the equivalent resistance \( R_{eq} \) can be calculated using the formula for resistors in parallel:
\(
\frac{1}{R_{eq}} = \frac{1}{R} + \frac{1}{R} + \ldots + \frac{1}{R} \quad (N \text{ times})
\)
This simplifies to:
\(
\frac{1}{R_{eq}} = \frac{N}{R} \implies R_{eq} = \frac{R}{N}
\)
Step 2: Calculate Total Resistance in the Circuit
The total resistance \( R_{total} \) in the circuit includes the internal resistance \( r \) of the source:
\(
R_{total} = R_{eq} + r = \frac{R}{N} + r
\)
Step 3: Calculate the Total Current in the Circuit
Using Ohm's law, the total current \( I \) flowing through the circuit can be calculated as:
\(
I = \frac{E}{R_{total}} = \frac{E}{\frac{R}{N} + r}
\)
Step 4: Calculate the Current through Each Bulb
Since the bulbs are in parallel, the current through each bulb \( I_{bulb} \) is given by:
\(
I_{bulb} = \frac{I}{N} = \frac{E}{N \left(\frac{R}{N} + r\right)} = \frac{E}{R + Nr}
\)
Step 5: Calculate the Power Consumed by Each Bulb
The power \( P \) consumed by each bulb can be calculated using the formula:
\(
P = I_{bulb}^2 \cdot R
\)
Substituting \( I_{bulb} \):
\(
P = \left(\frac{E}{R + Nr}\right)^2 \cdot R = \frac{E^2 R}{(R + Nr)^2}
\)
Step 6: Calculate the New Power when One Bulb Turns Off
When one bulb turns off, the number of bulbs becomes \( N-1 \). The new equivalent resistance becomes:
\(
R_{eq}' = \frac{R}{N-1}
\)
The new total resistance is:
\(
R_{total}' = R_{eq}' + r = \frac{R}{N-1} + r
\)
The new total current \( I' \) is:
\(
I' = \frac{E}{R_{total}'} = \frac{E}{\frac{R}{N-1} + r}
\)
The current through each remaining bulb is:
\(
I_{bulb}' = \frac{I'}{N-1} = \frac{E}{(N-1)\left(\frac{R}{N-1} + r\right)} = \frac{E}{R + (N-1)r}
\)
The new power \( P' \) consumed by each bulb is:
\(
P' = I_{bulb}'^2 \cdot R = \left(\frac{E}{R + (N-1)r}\right)^2 \cdot R = \frac{E^2 R}{(R + (N-1)r)^2}
\)
Step 7: Calculate the Percentage Change in Power
The percentage change in power when one bulb turns off is given by:
\(
\text{Percentage Change} = \frac{P - P'}{P} \times 100
\)
Substituting the values of \( P \) and \( P' \):
\(
\text{Percentage Change} = \frac{\frac{E^2 R}{(R + Nr)^2} - \frac{E^2 R}{(R + (N-1)r)^2}}{\frac{E^2 R}{(R + Nr)^2}} \times 100
\)
This simplifies to:
\(
\text{Percentage Change} = \left(1 - \frac{(R + Nr)^2}{(R + (N-1)r)^2}\right) \times 100
\)
After simplification, the final result for the percentage change in power is:
\(
\text{Percentage Change} = \frac{200R}{Nr + R}
\)
Final Answers
1. Power consumed by each bulb when N bulbs are connected:
\(
P = \frac{E^2 R}{(R + Nr)^2}
\)
2. Percentage change in power when one bulb turns off:
\(
\text{Percentage Change} = \frac{200R}{Nr + R}
\)
When N identical bulbs, each with resistance R, are connected in parallel, the equivalent resistance \( R_{eq} \) can be calculated using the formula for resistors in parallel:
\frac{1}{R_{eq}} = \frac{1}{R} + \frac{1}{R} + \ldots + \frac{1}{R} \quad (N \text{ times})
\)
\frac{1}{R_{eq}} = \frac{N}{R} \implies R_{eq} = \frac{R}{N}
\)
The total resistance \( R_{total} \) in the circuit includes the internal resistance \( r \) of the source:
R_{total} = R_{eq} + r = \frac{R}{N} + r
\)
Using Ohm's law, the total current \( I \) flowing through the circuit can be calculated as:
I = \frac{E}{R_{total}} = \frac{E}{\frac{R}{N} + r}
\)
Since the bulbs are in parallel, the current through each bulb \( I_{bulb} \) is given by:
I_{bulb} = \frac{I}{N} = \frac{E}{N \left(\frac{R}{N} + r\right)} = \frac{E}{R + Nr}
\)
The power \( P \) consumed by each bulb can be calculated using the formula:
P = I_{bulb}^2 \cdot R
\)
P = \left(\frac{E}{R + Nr}\right)^2 \cdot R = \frac{E^2 R}{(R + Nr)^2}
\)
When one bulb turns off, the number of bulbs becomes \( N-1 \). The new equivalent resistance becomes:
R_{eq}' = \frac{R}{N-1}
\)
R_{total}' = R_{eq}' + r = \frac{R}{N-1} + r
\)
I' = \frac{E}{R_{total}'} = \frac{E}{\frac{R}{N-1} + r}
\)
I_{bulb}' = \frac{I'}{N-1} = \frac{E}{(N-1)\left(\frac{R}{N-1} + r\right)} = \frac{E}{R + (N-1)r}
\)
P' = I_{bulb}'^2 \cdot R = \left(\frac{E}{R + (N-1)r}\right)^2 \cdot R = \frac{E^2 R}{(R + (N-1)r)^2}
\)
The percentage change in power when one bulb turns off is given by:
\text{Percentage Change} = \frac{P - P'}{P} \times 100
\)
\text{Percentage Change} = \frac{\frac{E^2 R}{(R + Nr)^2} - \frac{E^2 R}{(R + (N-1)r)^2}}{\frac{E^2 R}{(R + Nr)^2}} \times 100
\)
\text{Percentage Change} = \left(1 - \frac{(R + Nr)^2}{(R + (N-1)r)^2}\right) \times 100
\)
\text{Percentage Change} = \frac{200R}{Nr + R}
\)
1. Power consumed by each bulb when N bulbs are connected:
\(
P = \frac{E^2 R}{(R + Nr)^2}
\)
\(
\text{Percentage Change} = \frac{200R}{Nr + R}
\)
Topper's Solved these Questions
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Single Correct
60 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Multiple Correct
5 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Exercise 7.1
22 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Integer Type
4 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
Similar Questions
If electric bulbs having resistances in the ratio 2 : 3 are connected in parallel to a voltage sources of 220 V. The ratio of the power dissipated in them is
View SolutionTwo identical cells each of emf ε, having negligible internal reistance r, are connercted in parallel with each other across an external resistance R. What is the current through this resistance.
View SolutionTwo bulbs when connected in parallel to a source take 60 W each, the power consumed, when they are connected in series with the same source is
View SolutionTwo bulbs when connected in parallel to a source take 100 W each. The total power consumed when they are connected in series with the same source is
View SolutionThree bulbs,each having a resistance of 180(Ω)are connected in parallel to an ideal battery of emf60V. Find the current delivered by the battery when (a)all the bulbs are switched on,(b)two of the bulbs are switched on and (c )only one bulb is switched on.
View SolutionTwo identical bulbs are conneted in parallel across an ideal source of emf E . The ammeter A voltmeter V are ideal . If bulb B2 gets fused , then
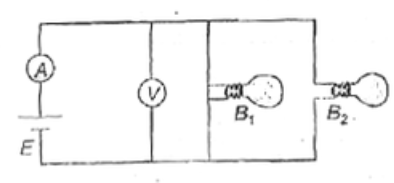 .
.
View SolutionThree identical bulbs are connected in parallel across an ideal sources of emf E .The ammeter A and voltmeter V are ideal . If bulb B3 gets fused, then
_PHY_E05_094_Q01.png)
View SolutionThe ratio of distance of two satellites from the centre of earth is 1 : 4. The ratio of their time periods of rotation will be :
View SolutionTwo electric bulbs whose resistances are in the ratio of 1:2 are connected inparallel to a constant voltage source. The powers dissipated in them have the ratio
View SolutionTwo cells , each of emfE and internal resistance r, are connected in parallel across a resistor R. The power delivered to the resistor is maximum if R is equal to
View Solution
