In a reference frame a photon of frequency falls normally on a mirror approaching it with relativistic velocity . Find the momentum inparted to the mirror during the reflection of the photon
(a) in the reference frame fixed to the mirroe,
(b) in the frame .
(a) In the reference frame fixed to the mirror, the frequency of the photons is, by the Doppler shift formula
In this frame momentum imparted to the mirror is
,
(b) In the frame, the incident particle carries a momentum of and returns with momentum
The momentum inparted to the mirror, then, has the magnitude
Hence .
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources
22 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
A plane electromagnetic wave of frequency ω0 falls normally on the surface of a mirror approching with a relativistic velocity V. Making use of the Doppler formula, find the frequency of the reflected wave. Simplify the obtained expression for the case V<<c.
View SolutionA moving source emits photons. Find the expressions for the energy and the momentum of a photon in the labo-ratory reference frame.
View Solution
Knowledge Check
A plane electromagnetic wave of frequency w0 falls normally on the surface of a mirror approaching with a relativisitic velocity v. Then frequency of the reflected wave will be (given β=vc):
A(1−β1+β)w0B1+β(1−β)w0C(1+β)w0(1−β)D(1−β)(1+β)w0
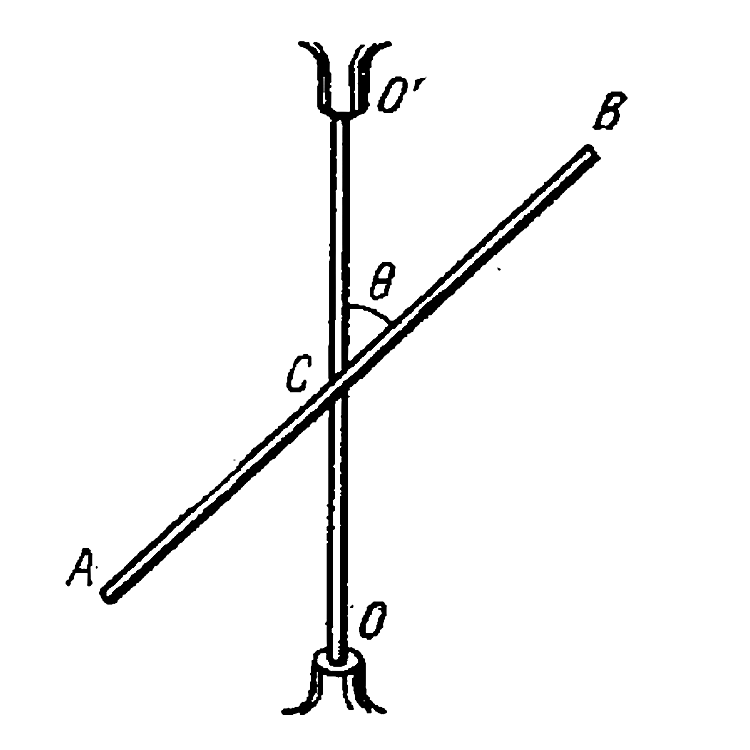
A midpoint of a thin uniform rod AB of mass m and length l is rigidly fixed to a rotation axle OO′ as shown in figure. The rod is set into rotation with a constant angular velocity ω. Find the resultant moment of the centrifugal forces of inertia relative to the point C in the reference frame fixed to the axle OO′ and to the end.
View SolutionA closed system consists of two particles of masses m1 and m2 which move at right angles to each other with velocities v1 and v2. Find:
(a) the momentum of each particle and
(b) the total kinetic energy of the two particles in the reference frame fixed to their centre of inertia.
View SolutionA satellite revolves from east to west in a circular equatorial orbit of radius R=1.00⋅104km around the Earth. Find the velocity and the acceleration of the satellite in the reference frame fixed to the Earth.
View SolutionA smooth horizontal disc rotates with a constant angular velocity ω about a stationary vertical axis passing through its centre, the point O. At a moment t=0 a disc is set in motion from that:
point with velocity v0. Find the angular momentum M(t) of the disc relative to the point O in the reference frame fixed to the disc. Make sure that this angular momentum is caused by the Coriolis force.
View SolutionTwo unstable particles move in the reference frame K along a straight line in the same direction with a velocity v=0.990c. The distance between them in this reference frame is equal to l=120m. At a certain moment both particles decay simultaneously in the reference frame fixed to them. What time interval between the moments of decay of the two particles will be observed in the frame K? Which particle decays later in the frame K?
View SolutionTwo rods having the same proper length l0 move lengthwise toward each other parallel to a common axis with the same velocity v relative to the laboratory frame of reference. What is the length of each rod in the reference frame fixed to the other rod?
View SolutionA rod moves along a ruler with a constant velocity. When the positions of both ends of the rod are marked simultaneously in the reference frame fixed to the rule, the difference of readings on the rule is equal to Δx1=4.0m. But when the positions of the rod's ends are marked simultaneously in the reference frame fixed to the rod, the difference of readings on the same ruler is equal to Δx2=9.0m. Find the proper length of the rod and its velocity relative to the ruler.
View Solution
