In a resonance tube the first resonance with a tuning fork occurs at 16 cm and second at 49 cm . If the velocity of sound is 330 m/s , the frequency of tuning fork is
The correct Answer is:500
To solve the problem, we need to find the frequency of the tuning fork using the information given about the resonance tube.
1. Identify the lengths of the first and second resonances:
- The first resonance occurs at L1=16cm.
- The second resonance occurs at L2=49cm.
2. Calculate the difference in lengths:
- The difference between the two resonance lengths is:
ΔL=L2−L1=49cm−16cm=33cm
3. Relate the difference in lengths to the wavelength:
- The difference in lengths corresponds to half the wavelength (λ2), since in a resonance tube, the distance between two consecutive resonances corresponds to half a wavelength.
- Therefore, we have:
λ2=33cm
- To find the wavelength (λ):
λ=2×33cm=66cm
4. Convert the wavelength to meters:
- Since the velocity of sound is given in meters per second, we convert λ to meters:
λ=66cm=0.66m
5. Use the wave equation to find the frequency:
- The wave equation relates velocity (v), frequency (f), and wavelength (λ):
v=f⋅λ
- Rearranging this equation to solve for frequency gives:
f=vλ
6. Substitute the values:
- Given that the velocity of sound v=330m/s:
f=330m/s0.66m=500Hz
7. Final answer:
- The frequency of the tuning fork is 500Hz.
- The first resonance occurs at L1=16cm.
- The second resonance occurs at L2=49cm.
- The difference between the two resonance lengths is:
ΔL=L2−L1=49cm−16cm=33cm
- The difference in lengths corresponds to half the wavelength (λ2), since in a resonance tube, the distance between two consecutive resonances corresponds to half a wavelength.
- Therefore, we have:
λ2=33cm
- To find the wavelength (λ):
λ=2×33cm=66cm
- Since the velocity of sound is given in meters per second, we convert λ to meters:
λ=66cm=0.66m
- The wave equation relates velocity (v), frequency (f), and wavelength (λ):
v=f⋅λ
- Rearranging this equation to solve for frequency gives:
f=vλ
- Given that the velocity of sound v=330m/s:
f=330m/s0.66m=500Hz
- The frequency of the tuning fork is 500Hz.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective Type2videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type5videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple Correct Answers Type5videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 241videos
THERMODYNAMICS
Similar Questions
In a resonance tube experiment when the tube is filled with water up to a height of 17.0 cm from bottom, it resonates with a givben tunign fork. When the water level is raised the next resonance with the same tuning fork occurs at a height of 24.5 cm. If the velocity of sound in air is 330m/s,the tunign fork frequency is :
View SolutionIf a resonance tube is sounded with a tuning fork of frequency 256 Hz, resonance occurs at 35 cm and 105 cm. The velocity of sound is about
View Solution
Knowledge Check
- Question 1 - Select One
A long glass tube is held vertically in water . A tuning fork is struck and held over the tube . Strong resonances are observed at two successive lengths 0.50mand0.84m above the surface of water . If the velocity of sound is 340m/s, then the frequency of the tuning fork is
A128 HzB256 HzC384 HzD500 Hz
In resonance tube tube successive positions of resonance are obtained at 15 cm and 48 cm . If the frequency of the fork is 500 cps , the velocity of sound is
View SolutionA vibrating string of certain length l under a tension T resonates with a mode corresponding to the first overtone (third harmonic) of an air column of length 75cm inside a tube closed at one end. The string also generates 4 beats per second when excited along with a tuning fork of frequency n. Now when the tension of the string is slightly increased the number of beats reduces 2 per second. Assuming the velocity of sound in air to be 340m/s, the frequency n of the tuning fork in Hz is
View SolutionA tuning fork makes 256 vibrations per second in air. When the speed of sound is 330m/s, the wavelength of the note emitted is :
View SolutionThere is a source of sound (a tuning fork) moving towards a reflecting wall will a speed of 30ms−1. The velocity of the sound in air is 330ms−1and the frequency of the tuning fork is 600 Hz. The observer is between the source and the wall and is moving with some velocity V1 towards the wall. The beat frequency heard by the observer is 4 Hz. If the tuning fork is waxed, the frequency beats heard by the observer becomes 3 Hz. If the new frequency of tuning fork is k then k/50 is
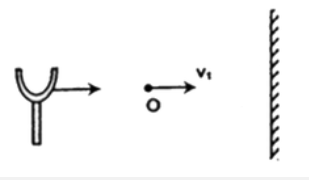 View Solution
View SolutionIn determination of velocity of sound in air using resonance column tube, radius of cylindrical tube is 1 cm and frequency of tuning fork is 256 Hz. If velocity of sound is 340 m/s then length of air column in the tube when first resonance occurs, is
View SolutionWhen the wire of a sonometer is 73 cm long, it is in resonance with a tuning fork. On shortening the wire by 0.5 cm, it makes 3 beats per second with the same fork. Calculate the frequency of the tuning fork?
View SolutionIn a resonance column the first resonant Iength is 0.16 in using a tuning fork of frequency 512 Hz. If the diameter of the tube is 0.025 m, calculate the velocity of sound ?
View Solution

