In a velocity selector , the intensity of the electric field is 3×104 V/m and the strength of the magnetic field is 0.015Wb/m2 . The speed of the charged particles which pass undeflected through the velocity selector is
2×106 m/s
2×10−6 m/s
5×106 m/s
5×10−6 m/s
The correct Answer is:A
Step by step video, text & image solution for In a velocity selector , the intensity of the electric field is 3 xx 10^(4) V/m and the strength of the magnetic field is 0.015 Wb//m^2 . The speed of the charged particles which pass undeflected through the velocity selector is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
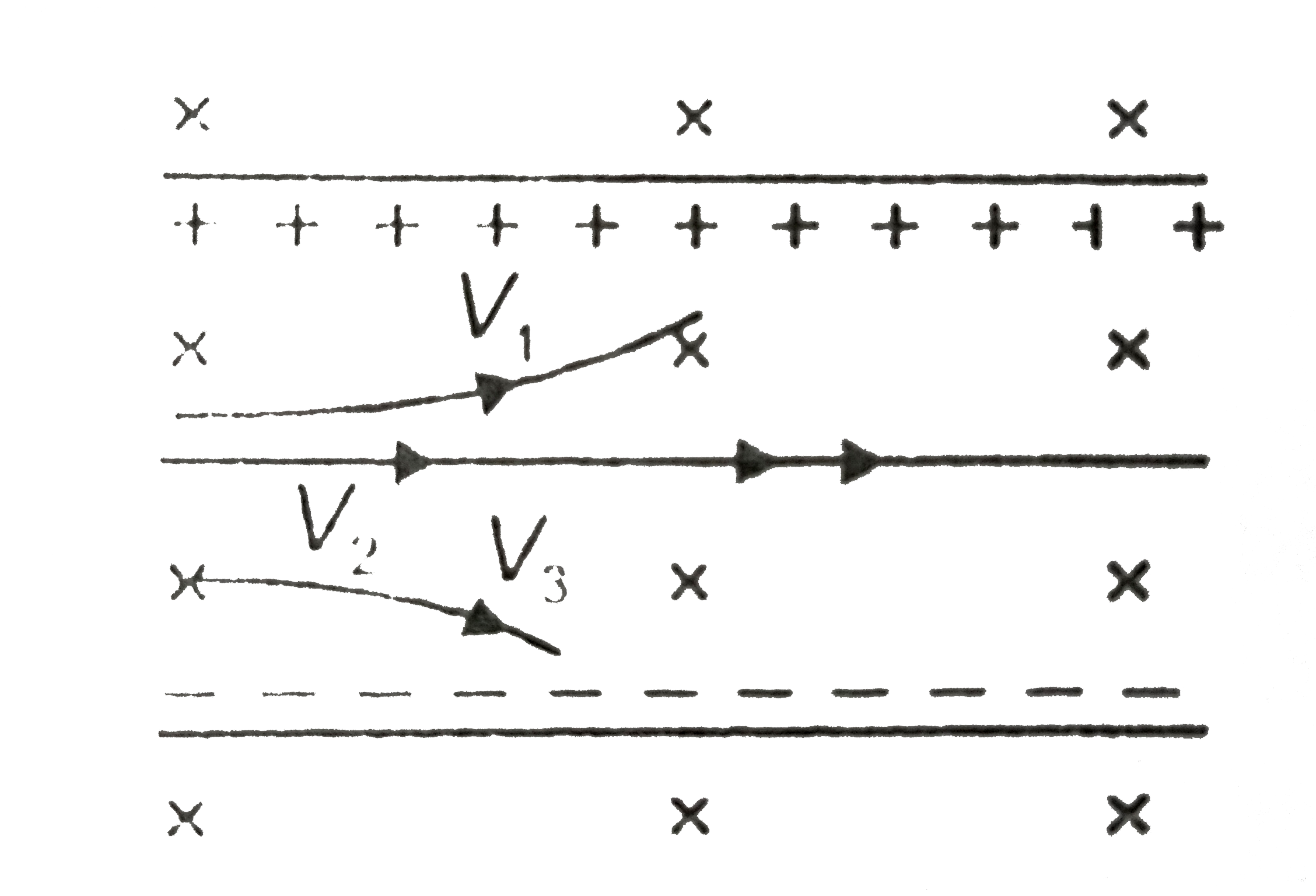
In a region of crossed fields as shown, the strength of electric field is E and that of magnetic field is B. Three positively charged particles with speeds V1,V2andV3 are projected and their paths are shown. From this it implies that
View SolutionWrite the expression for the force →F, acting on a charged particle of charge q moving with a velocity →v in the pressure of both electric field →E and magnetic field →B. Obtain the condition under which the particle moves undeflected through the fields.
View Solution
Knowledge Check
If the velocity of the charged particle is doubled and strength of magnetic field is halved, then radius becomes
A8 timesB4timesC2timesD16timesAn electron enters a region where electrostatic field is 20N/C and magnetic field is 5T. If electron passes undeflected through the region, then velocity of electron will be
A0.25ms−1B2ms−1C4ms−1D8ms−1A charged particle can be deflected by electric and magnetic filed both The electric force→Fe=q→E and magnetic force −→Fm=→qv×→Bq = charge in coulomb, oversetrarrV is velocity in m//s →E is electric filed strengh in N/C and B is magnetic field strength in tesla (T) If a moving charged particle enters simultaneous electric and magnetic field, the net force on the charged particle is
→F=q(→E+→v×→B)
If →F=0 the charged particle will move undeflected in simultaneous fields
A charged particle moves in simultaneous electric field →E and magnetic field →B If the charged particle passes undeflected , then the value of electric field vectore →E must be .A→E=→v×→BB→E=−→v×→BC→E=→B→vD→E=→v→B
The amplitude of the electric field of a plane electromagnetic wave in air is 6.0×10−4Vm−1. The amplitude of the magnetic field will be
View SolutionA proton is moving with a velocity of 5×105m/s along the Y-direction. It is acted upon by an electric field of intensity 105 V/m along the X-direction and a magnetic field of 1Wb/m2 along the Z-direction. Then the Lorentz force on the particle is :
View SolutionBainbridge's mass spectrometer separates ion having the same velocity. The ions, after enteering through slits, S1 and S2, pass through a velocity selector composed of an electric field pproduced by the charged plates P and P' abd a magnetic field →B perpendicular to electric field and the ion path.Thew ions that then passs undeviated through the crossed →E1 and →B fields enter into a region where a second magnetic field →B exists, where they are made to follow circular path. A photographic plate ( or a modern detector) registors their arrival. If r is the radius of the circular orbit , then specific charge qm of ion is

View SolutionAn electric field of 1500 V/m and a magnetic field of 0.40 Wb/m2 act on a moving electron. The minimum uniform speed along a straight line, the electron could have is
View SolutionIn an experiment to measure the charge on an electrons , the electrons travelling at a speed of 1.825×107 m/s are subjected to a deflecting electric field 3.2×104 V/m . The intensity of magnetic field applied so that the beam remains undeflected is
View Solution
