Question

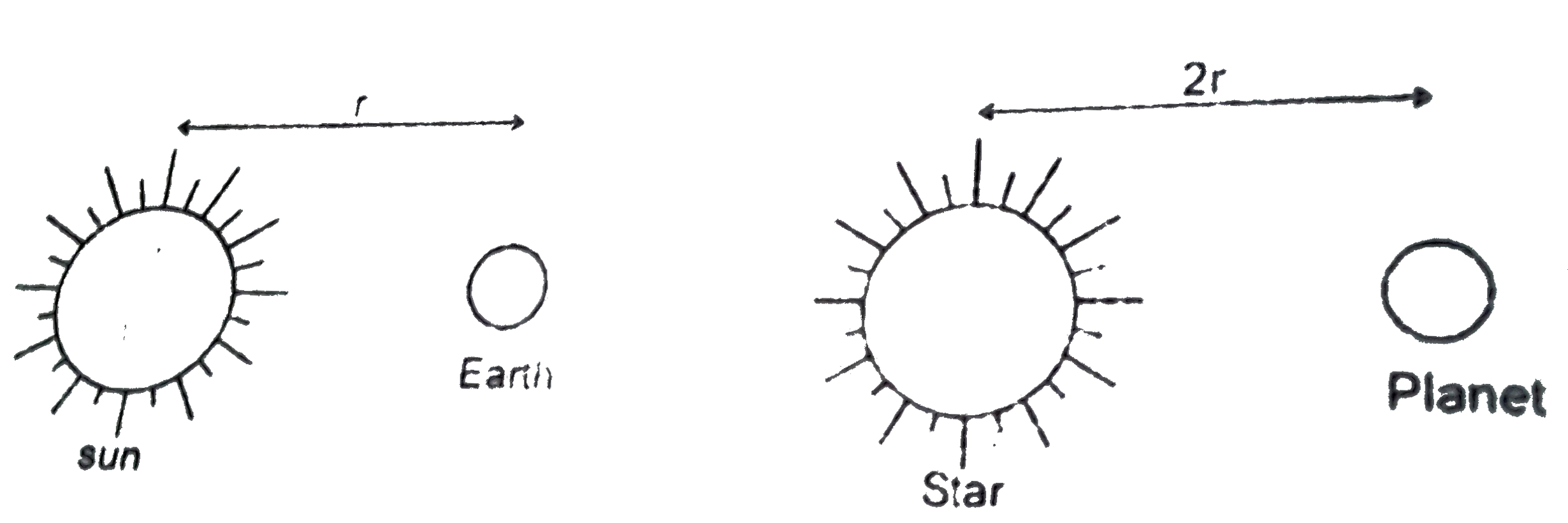
In an imaginary planetary system, the central star has the same mass as our sun, but is much brighter so that only a planet twice the distance between the earth and the sun can support life. Assuming biological evolution ( including aging process etc.) on that is similar to ours, what would be the average life span of a 'human' on that planet in terms of its natural year? The average life span of a human on the earth may be taken to be 70 years.

Explanation

To solve the problem, we need to determine the average lifespan of a human on an imaginary planet that is twice the distance from its star compared to the Earth-Sun distance. We will use Kepler's Third Law of planetary motion to find the relationship between the orbital period of the planet and its distance from the star.

1. Identify the parameters:
- Let the distance between the Earth and the Sun be \( a \).
- The distance from the central star to the imaginary planet is \( a' = 2a \).
- The average lifespan of a human on Earth is \( 70 \) years.

2. Apply Kepler's Third Law:
- According to Kepler's Third Law, the square of the orbital period \( T \) of a planet is directly proportional to the cube of the semi-major axis \( R \) of its orbit:
\( T^2 \propto R^3 \)
- For Earth, we can write:
\( T_1^2 \propto a^3 \)
- For the imaginary planet, we write:
\( T_2^2 \propto (2a)^3 = 8a^3 \)

3. Set up the ratio of the periods:
- From the above equations, we can set up the ratio:
\( \frac{T_2^2}{T_1^2} = \frac{8a^3}{a^3} = 8 \)
- Taking the square root gives:
\( \frac{T_2}{T_1} = \sqrt{8} = 2\sqrt{2} \)

4. Express \( T_2 \) in terms of \( T_1 \):
- Therefore, we can express \( T_2 \) as:
\( T_2 = 2\sqrt{2} T_1 \)

5. Calculate the lifespan on the imaginary planet:
- Since \( T_1 \) (the period for Earth) corresponds to 1 year, the period for the imaginary planet becomes:
\( T_2 = 2\sqrt{2} \text{ years} \)
- To find the average lifespan of a human on the imaginary planet, we can set up the following relationship:
\( \text{Lifespan on imaginary planet} = \frac{70 \text{ years}}{T_2} = \frac{70}{2\sqrt{2}} \approx 24.74 \text{ years} \)

Final Answer:
The average lifespan of a human on that imaginary planet would be approximately 24.74 years.