In Fig. a particle is moving in a circle of radius r centred at O with constant speed v. what is the change in velocity in moving from A to B ? Given ∠AOB=40∘.
To find the change in velocity of a particle moving from point A to point B along a circular path with a constant speed v and an angle ∠AOB=40∘, we can follow these steps:
Step 1: Identify the Initial and Final Velocities
- The initial velocity →Vi at point A can be represented as:
→Vi=v^i
(assuming the velocity is directed along the positive x-axis).
- The final velocity →Vf at point B can be determined by the angle ∠AOB. The angle between the radius to point A and the radius to point B is 40∘.
Step 2: Calculate the Components of the Final Velocity
- The final velocity →Vf can be broken down into its components using trigonometry:
- The x-component of the final velocity is:
Vfx=vcos(40∘)
- The y-component of the final velocity is:
Vfy=vsin(40∘)
- Thus, the final velocity vector can be expressed as:
→Vf=Vfx^i+Vfy^j=vcos(40∘)^i+vsin(40∘)^j
Step 3: Calculate the Change in Velocity
- The change in velocity Δ→V is given by the difference between the final and initial velocities:
Δ→V=→Vf−→Vi
- Substituting the expressions for →Vf and →Vi:
Δ→V=(vcos(40∘)^i+vsin(40∘)^j)−(v^i)
- This simplifies to:
Δ→V=(vcos(40∘)−v)^i+vsin(40∘)^j
Δ→V=v(cos(40∘)−1)^i+vsin(40∘)^j
Final Result
The change in velocity as the particle moves from A to B is:
Δ→V=v(cos(40∘)−1)^i+vsin(40∘)^j
- The initial velocity →Vi at point A can be represented as:
→Vi=v^i
(assuming the velocity is directed along the positive x-axis).
- The final velocity →Vf can be broken down into its components using trigonometry:
- The x-component of the final velocity is:
Vfx=vcos(40∘)
- The y-component of the final velocity is:
Vfy=vsin(40∘)
- Thus, the final velocity vector can be expressed as:
→Vf=Vfx^i+Vfy^j=vcos(40∘)^i+vsin(40∘)^j
- The change in velocity Δ→V is given by the difference between the final and initial velocities:
Δ→V=→Vf−→Vi
- Substituting the expressions for →Vf and →Vi:
Δ→V=(vcos(40∘)^i+vsin(40∘)^j)−(v^i)
Δ→V=(vcos(40∘)−v)^i+vsin(40∘)^j
Δ→V=v(cos(40∘)−1)^i+vsin(40∘)^j
The change in velocity as the particle moves from A to B is:
Δ→V=v(cos(40∘)−1)^i+vsin(40∘)^j
Topper's Solved these Questions
- VECTORSBOOK - SL ARORACHAPTER - VECTORSEXERCISE - Problems For Self Practice
56 Videos
- VECTORSBOOK - SL ARORACHAPTER - VECTORSEXERCISE - TYPE F
2 Videos
- Units and MeasurementsBOOK - SL ARORACHAPTER - Units and MeasurementsEXERCISE - Exercise
499 Videos
- WAVE MOTIONBOOK - SL ARORACHAPTER - WAVE MOTIONEXERCISE - All Questions
271 Videos
Similar Questions
A particle is moving in a circle of radius having centre at 0. with a constant speed V. The magnitude at change in velocity in moving from A to B is 60°
View SolutionA particle is moving an a cirle of radius 'r' and centre O with constant speed . What is the change in velocity in moving from A to B ?
(If any angle between two velocity vector is θ.)
View Solution
Knowledge Check
A particle is moving in a circle of radius r centred at O with constant speed v. What is the change in velocity in moving from A to B (∠AOB=40∘) ?
A2vsin20∘B4vsin40∘C2vsin40∘Dvsin20∘A particle is moving in a circle of radius r having centre at O with a constant speed v . The magnitude of change in velocity in moving from A to B is
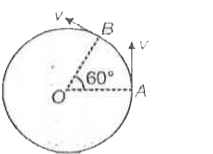 A2 vBZeroC√3vDv
A2 vBZeroC√3vDvA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
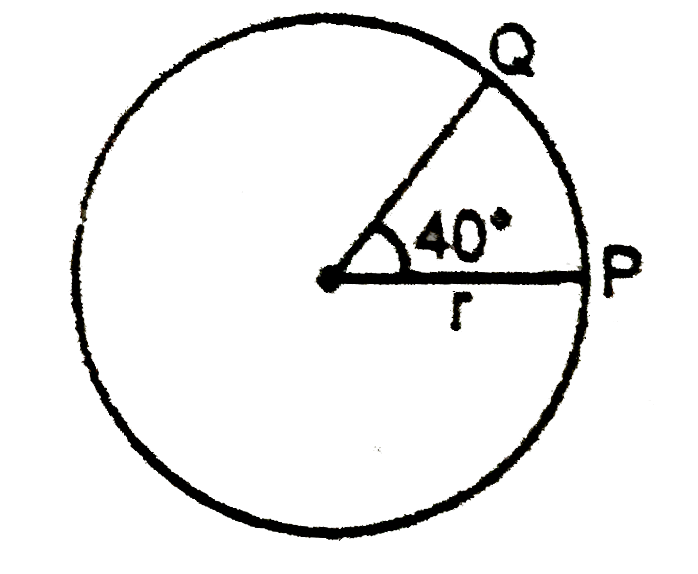 A2vcos40∘B2vsin40∘C2vcos20∘D2vsin20∘
A2vcos40∘B2vsin40∘C2vcos20∘D2vsin20∘
A particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
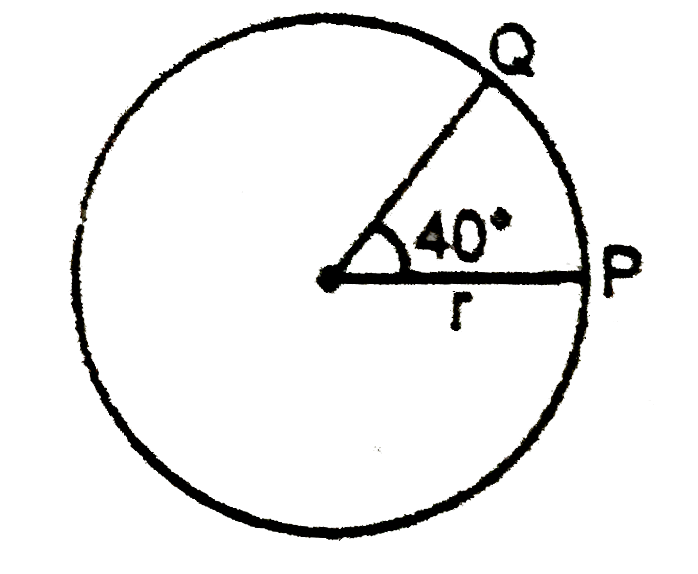
View SolutionIf the body is moving in a circle of radius r with a constant speed v , its angular velocity is
View SolutionA particle is moving on a circular path with a constant speed 'v'. Its change of velocity as it moves from A to B is:

View SolutionA particle is moving in a circle with a constant speed. Its moving is
View SolutionA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B

View Solution
