In previous question, if the second pendulum bob is thrown at velocity $v_{2}$ at an angle $β$ from mean position, but on other side of mean position , find the phase diffrence in the two SHMs now as show in the figure.

Video Solution

Text Solution

Verified by Experts

In this case , amplitudes of the two SHMs will still remain the same and are given by Eqs (i) and (ii) but when we represent the two SHM s on their corresponding circular motion , the position of the partical of the principal in circular motion , the position of the partical in circular motion in second pendulum is now difference as shown in figure .

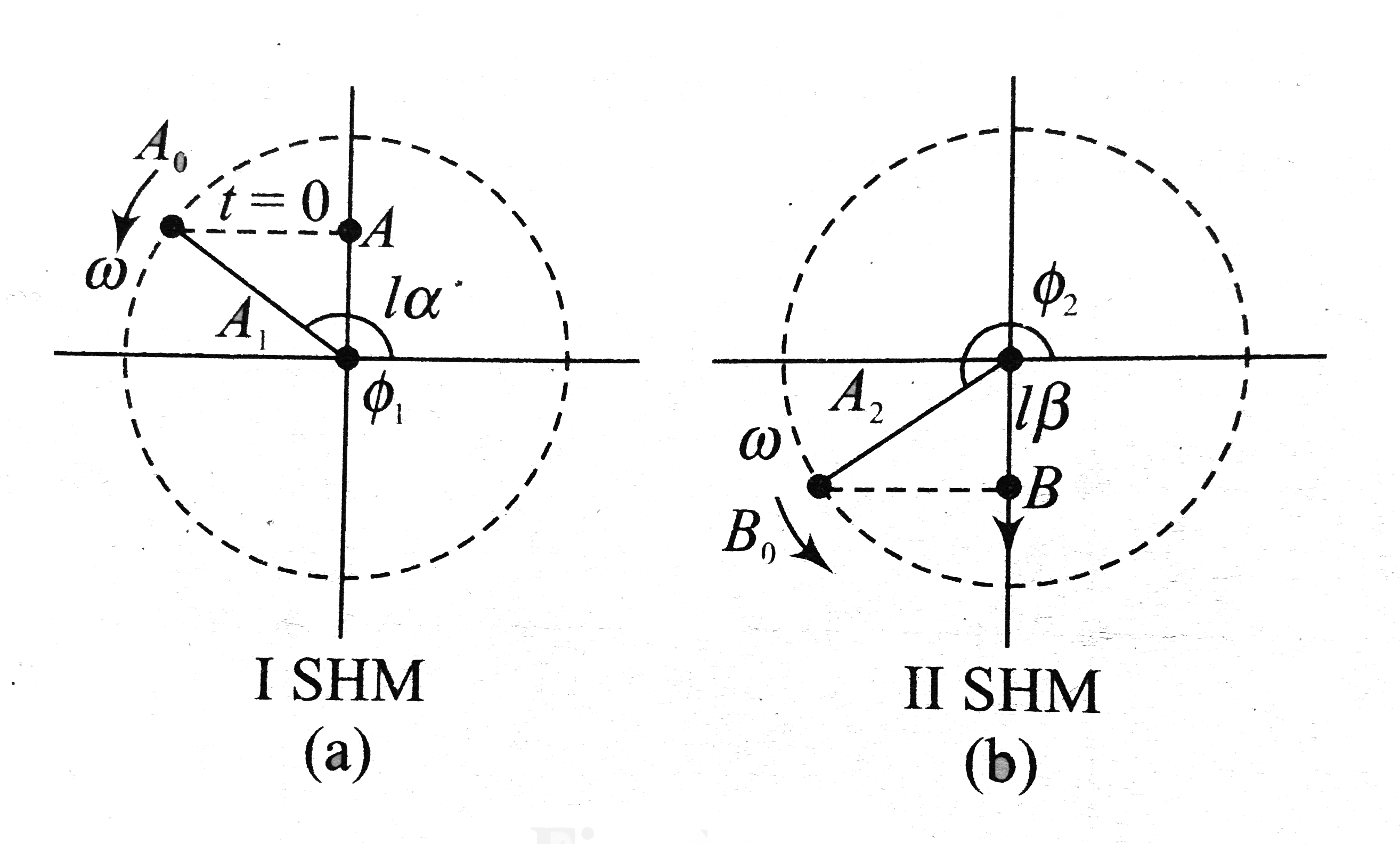
As shown in figure (a) and (b) the initial phase of the two pendulum bobs are
$ϕ_{1} = π - {sin}^{- 1} (\frac{l α}{A_{1}}) and ϕ_{2} = π + {sin}^{- 1} (\frac{l β}{A_{2}})$
As $ω$ for both SHm are same, their phase difference remain constant so it is given as
$Δ ϕ_{2} - π_{1} = {sin}^{- 1} (\frac{l β}{A_{2}}) + {sin}^{- 1} (\frac{l α}{A_{1}})$

Updated on:21/07/2023

Two simple pendulums of equal length cross each other at mean position. Then their phase difference is
A $π$ radian
B $\frac{3 π}{2}$ radian
C $\frac{π}{2}$ radian
D0 radian
The velocity of a particle in simple harmonic motion at displacement y from mean position is
A $ω \sqrt{(a^{2} + y^{2})}$
B $ω \sqrt{(a^{2} - y^{2})}$
C $w y$
D $ω^{2} \sqrt{(a^{2} + y^{2})}$
Two particle are in $S H M$ on same striaght line with amplitude $A$ and $2 A$ and with same angular frequency $ω$ . It is observed that when first particle is at as distance $\frac{A}{\sqrt{2}}$ from origin and going toward mean position, other particle is at extreme postion on other side of mean positon. find phase difference betwenen he two paricles.
A $45^{\circ}$
B $90^{\circ}$
C $135^{\circ}$
D $180^{\circ}$