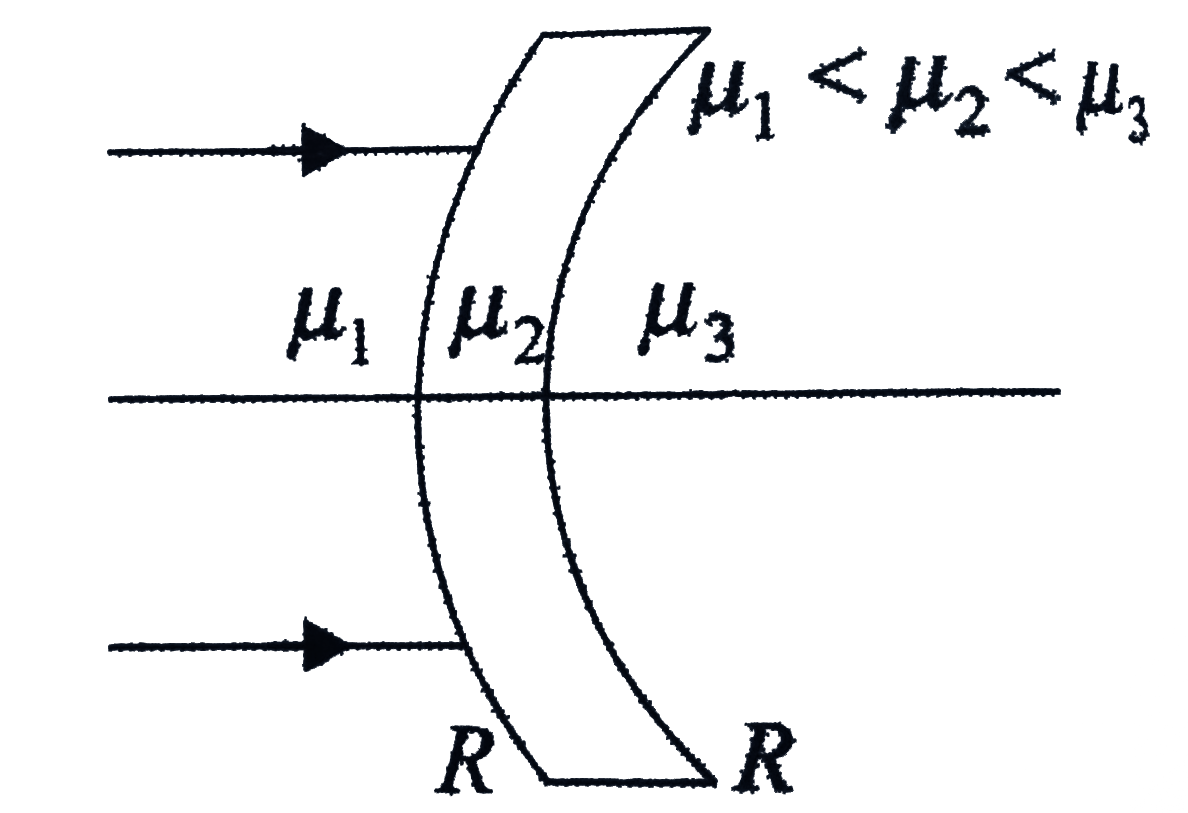
In the figure, light is incident on a thin lens as shown. The radius of curvature for both the surfaces is R. Determine the focal length of this system. 
For refraction at first surface,
μ2v1−μ1−∞=μ2−μ1+R………(i)
For refraction at second surface,
μ3v2−μ2v1=μ3−μ2+R …………(ii)
Adding Eqs. (i) and (ii), we get μ3v2−μ2−μ1Rorv2=μ3Rμ3−μ1

Therefore, focal length of the given lens system is
f=μ3Rμ3−μ1
Topper's Solved these Questions
- REFRACTION OF LIGHTBook:DC PANDEYChapter:REFRACTION OF LIGHTExercise:Solved Example
Explore 15 Videos
- REFRACTION OF LIGHTBook:DC PANDEYChapter:REFRACTION OF LIGHTExercise:Exercise 31.1
Explore 3 Videos
- REFRACTION OF LIGHTBook:DC PANDEYChapter:REFRACTION OF LIGHTExercise:Example Type 10
Explore 1 Video
- REFLECTION OF LIGHTBook:DC PANDEYChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
- SEMICONDUCTORSBook:DC PANDEYChapter:SEMICONDUCTORSExercise:Subjective
Explore 12 Videos
Similar Questions
Knowledge Check
Radius of curvature of a equi convex lens is R. Find focal length (n=32)
AR2B−R2CRD-RRadius of curvature of a equi convex lens is R. Find focal length (n=32)
AR2B−R2CRD-RA plano-convex lens is made of refractive index 1.6. The radius of curvature of the curved surface is 60 cm. The focal length of the lens is
A200 cmB100 cmC50 cmD400 cm
Find the focal length of the lens shown in the figure. The radii of curvature of both the surfaces are equal to R
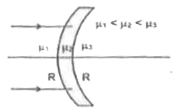
View SolutionWhat is the refractive index of material of a plano-convex lens , if the radius of curvature of the convex surface is 10 cm and focal length of the lens is 30 cm ?
View SolutionA planoconvex lens is made of refractive index 1.6. The radius of curvature of curved surface is 60 cm. The focal length of the lens is
View SolutionA plano-convex lens is made of glass of refractive index 1.5. The radius of curvature of its convex surface is R. Its focal length is
View SolutionA plano-convex lens is made of glass of refractive index 1.5. the radius of curvature of its convex surface is R. its focal length is
View Solution