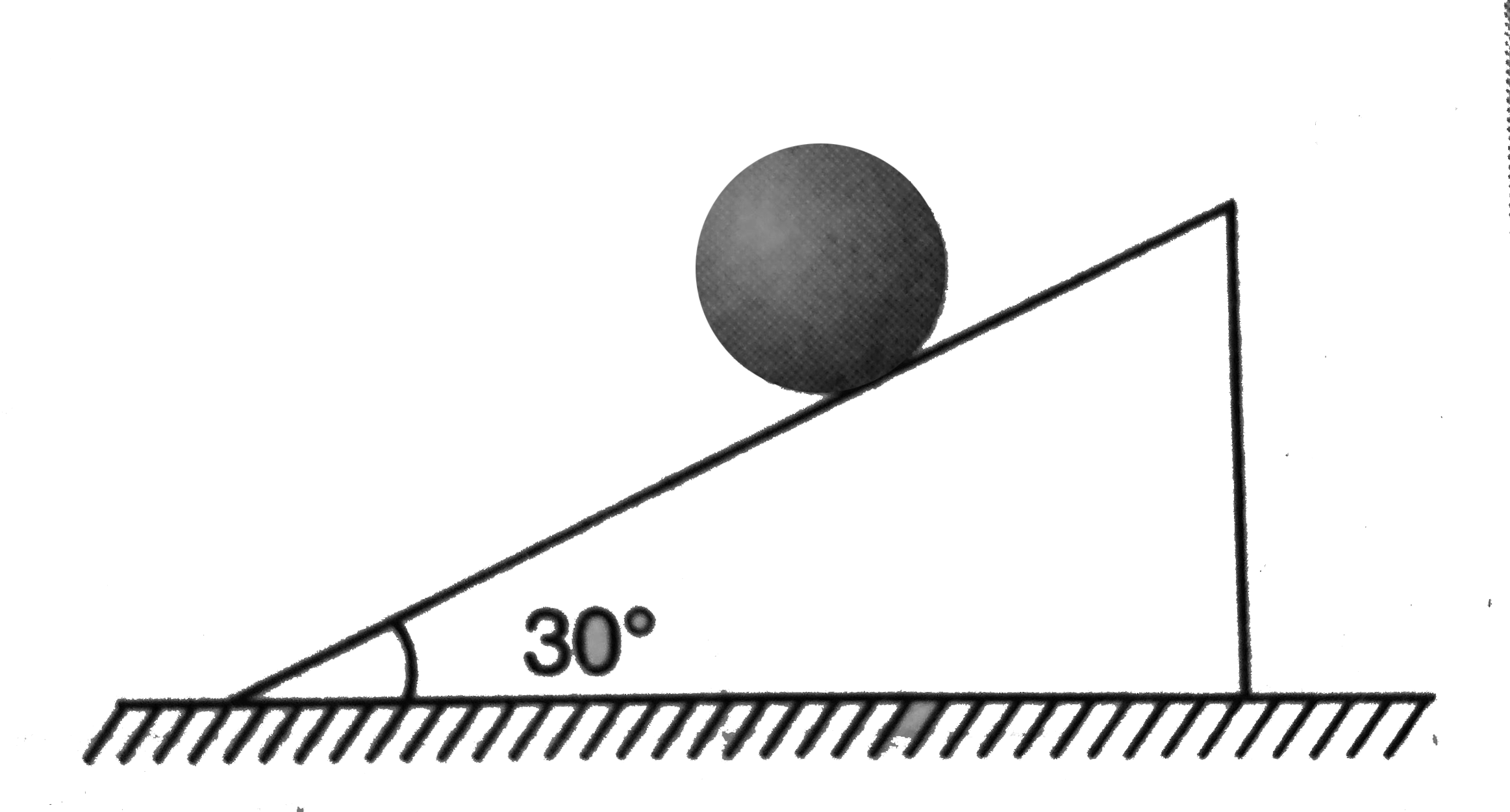
In the figure shown a solid sphere of mass 4kg and radius 0.25 m is placed on a rough surface. (g=10ms2)
(a). Minimum coefficient of friction for pure rolling to take place,
(b). If μ>μmin find linear acceleration of sphere.
(c). if μ=μmin2, find the linear acceleration of cylinder.
Here μmin is the value obtained part (a).
(a). μmin=tanθ1+(mR2/I)
=tan30∘1+5/2=27√3
(b). a=gsinθ1+I/mR2
=(10)sin30∘1+2/5
=257m/s2
(c) a=gsinθ−μgcosθ
=(10)sin30∘−(17√3)(10)(cos30∘)
=5−57=307m/s2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercise 12.11
2 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Assertion And Reason
11 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercise 12.9
2 Videos
- ROTATIONBOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
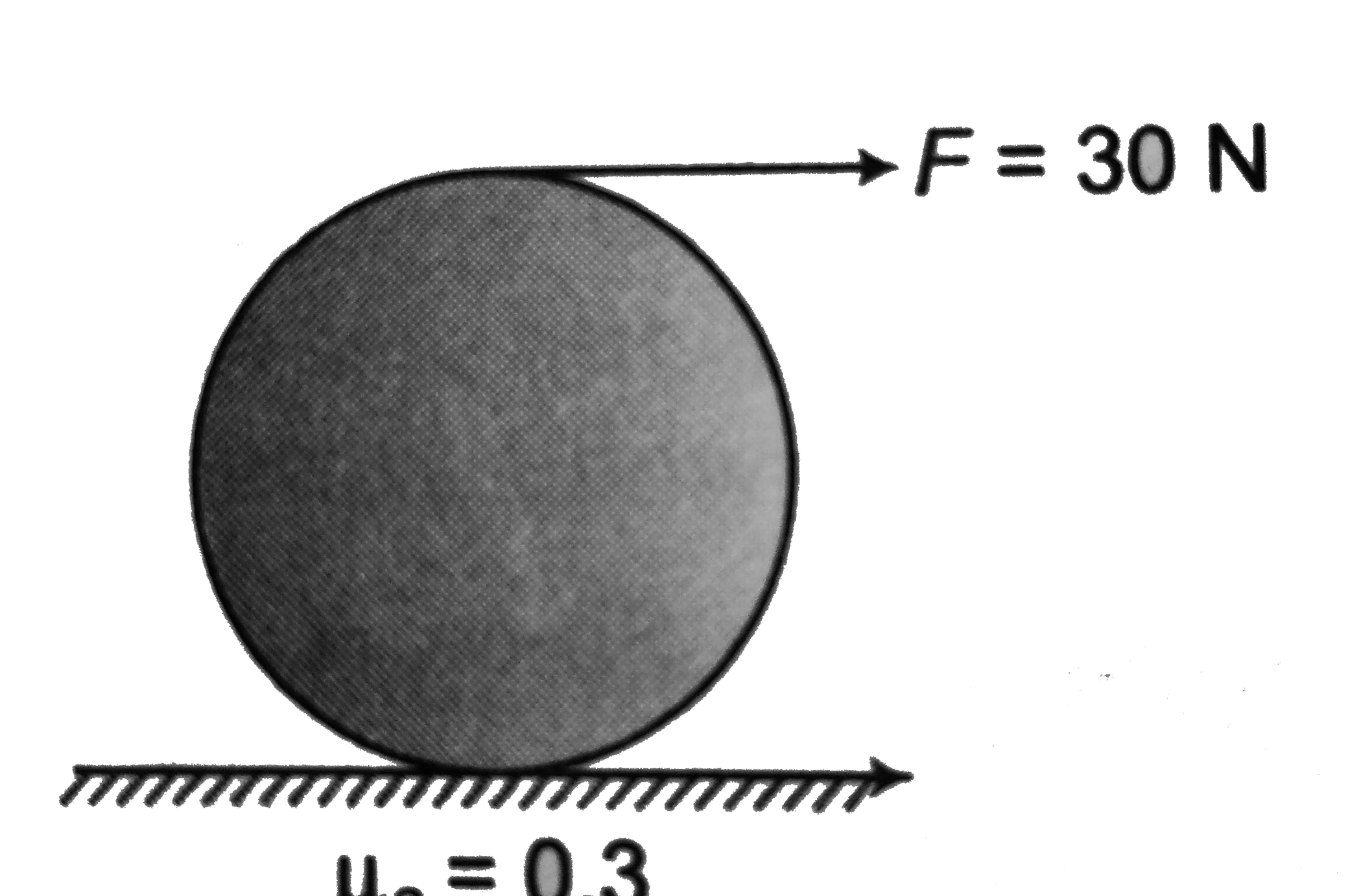
A solid sphere of mass 5 kg and radius 1m is kept over a rough surface as shown in figure. A force F=30N is acting at the topmost point.
(a). Check whether the pure rolling will take place or not
(b). Find direction and magnitude of friction actually acting on the sphere.
(c). Find linear acceleration a and angular acceleration α take g=10m/s2
View SolutionA body of mass 25 kg, is placed on a horizontal surface whose coefficient of friction 0.25. Find the frictional force action on it. Take (g=10ms−2)
View Solution
Knowledge Check
A sphere of mass M and radius R is moving on a rough fixed surface, having coefficient of friction μ. as shown in Fig. It will attain a minimum linear velocity after time v0>ω0R)
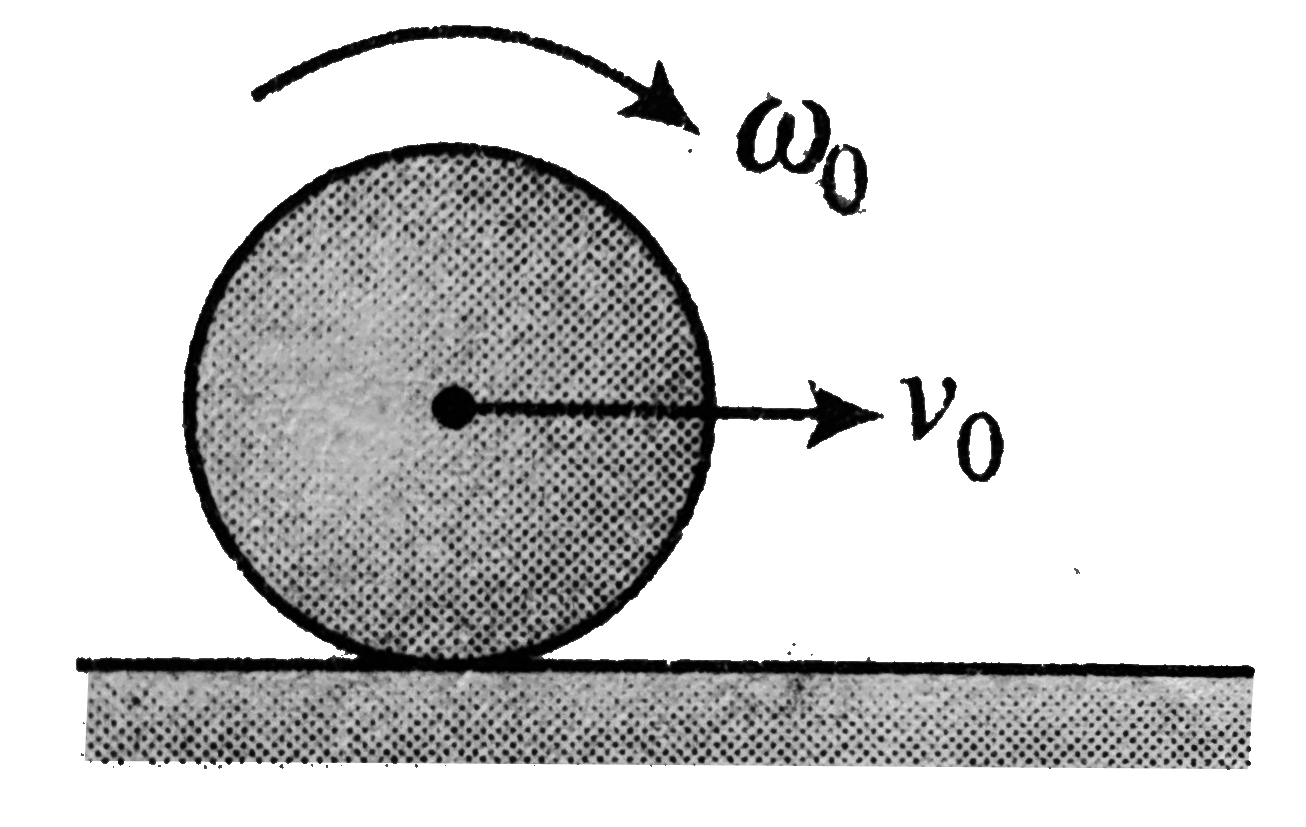 Av0/μgBω0R/μgC2(v0−ω0R)/5μgD2(v0−ω0R)/7μg
Av0/μgBω0R/μgC2(v0−ω0R)/5μgD2(v0−ω0R)/7μgA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
A0.5m/s2B5m/s2C10m/s2D15m/s2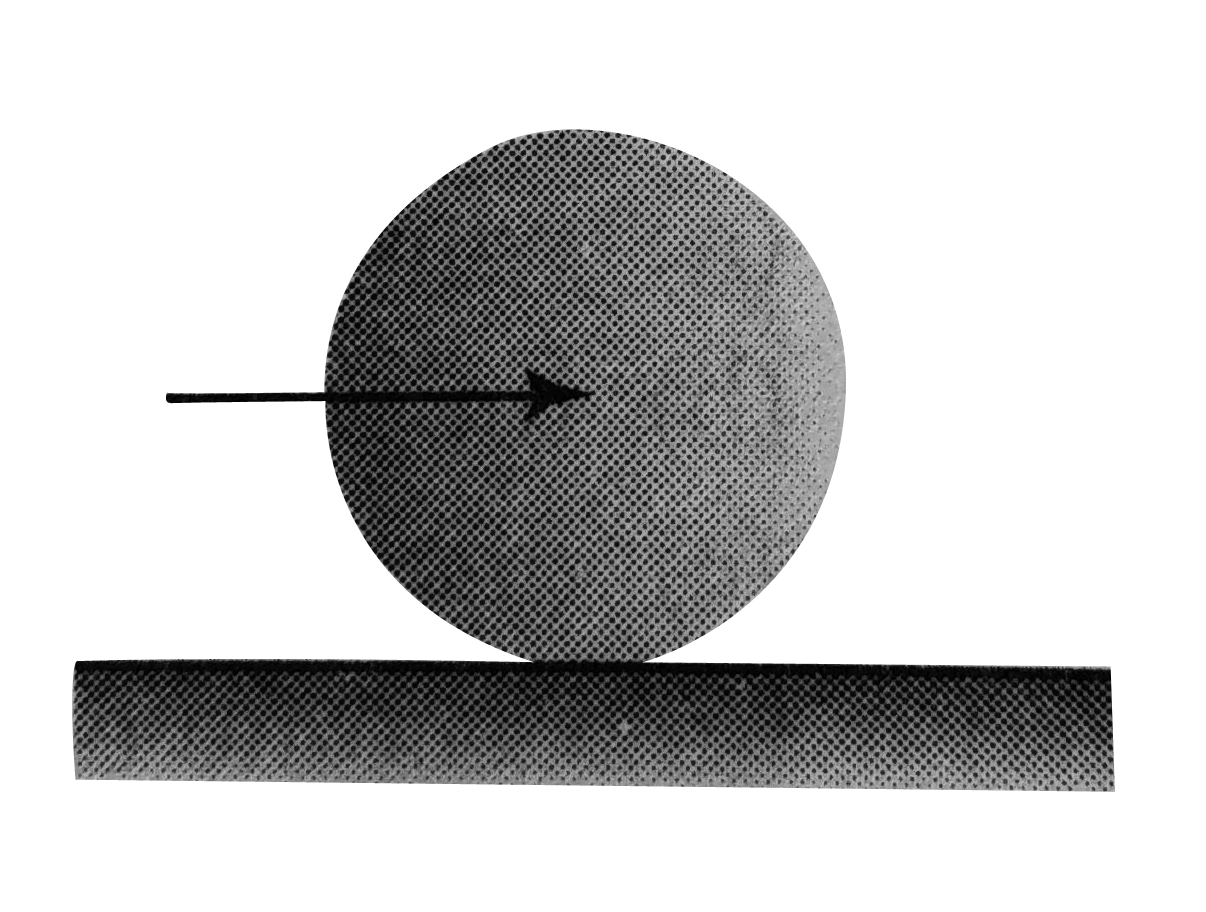
A solid sphere of mass 10 kg is placed on a rough surface having coefficient of friction μ=0.1A constant force F=7N is applied along a line passing through the centre of the sphere as shown in the figure. The value of frictional force on the sphere isA1 NB2 NC3 ND7 N
A ball of mass M and radius R is released on a rough inclined plane of inclination θ. Friction is not sufficient to prevent slipping. The coefficient friction between the ball and the plane is μ. Find
(a). The linear acceleration of the ball down the plane.
(b). the angular acceleration of the ball about its centre of mass.
View SolutionA block of mass 6 kg is kept on rough surface as shown in figure. Find acceleration and friction force acting on the block. (Take g=10m/s2)
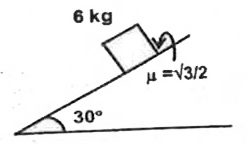
View SolutionA plank of mass 3m is placed on a rough inclined plane and a man of mass m, the coefficient of friction between the board and inclined plane is μ=0.5, the minimum acceleration of man so that plank does not slide is :
View SolutionA solid sphere of mass 10kg is placed on a rough surface having coefficient of friction μ=0.1. A constant force F=7N is applied along a line passing through the centre of the sphere as shown in the figure. The value of frictional force on the sphere is.
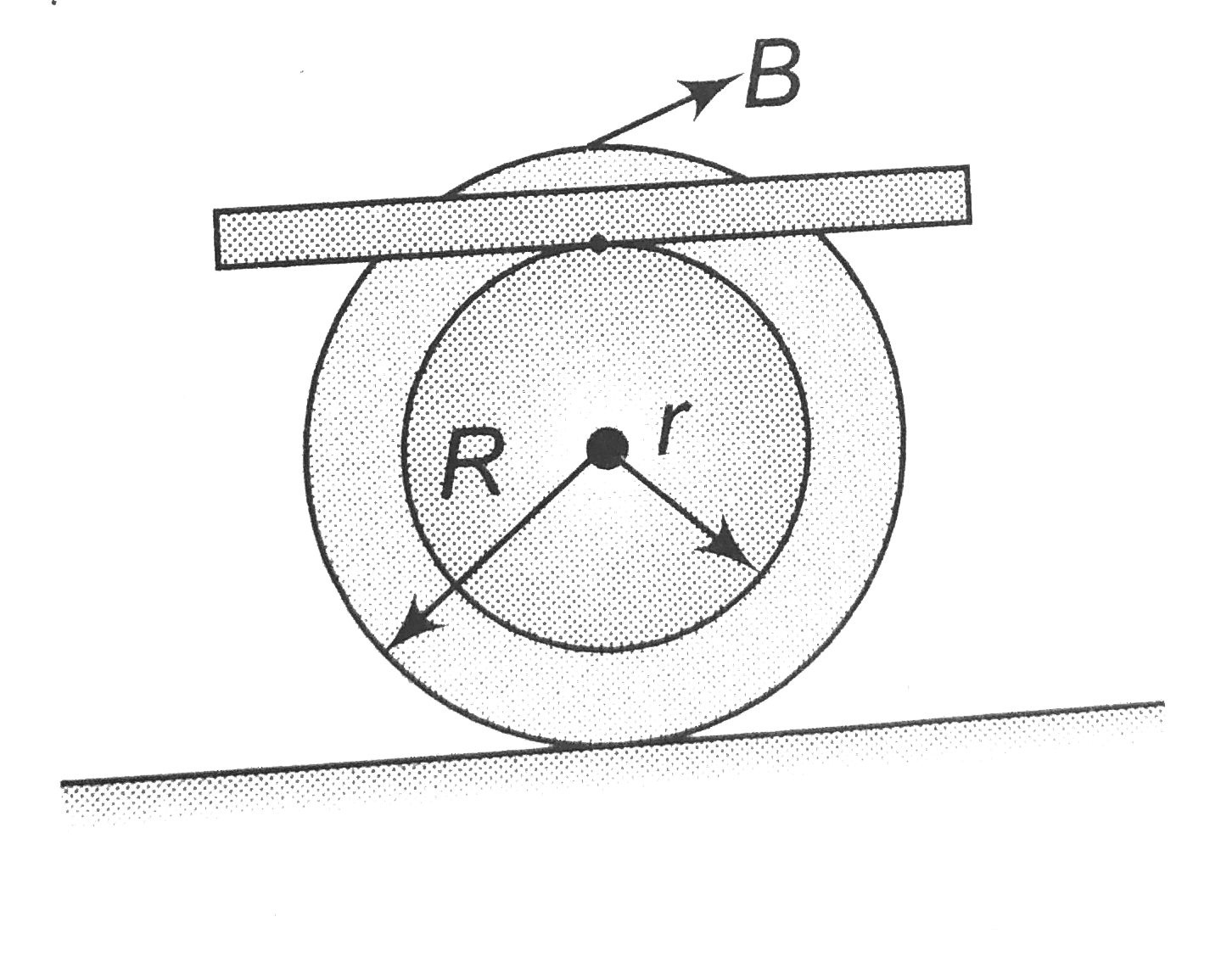 .
.
View SolutionA uniform cylinder of mass M and radius R is placed on a rough horizontal board, which in turn is placed on a smooth surface. The coefficient of friction between the board and the cylinder is μ. If the board starts accelerating with constant acceleration a, as shown in the figure, then

View Solution
