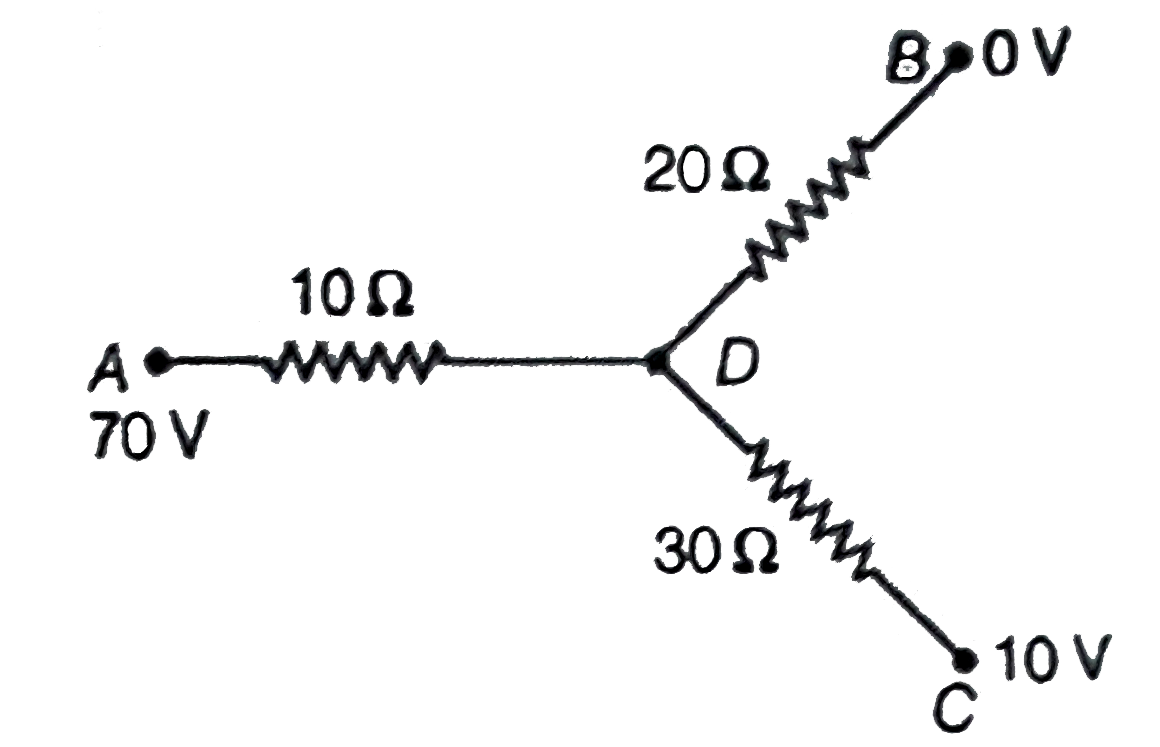
In the network shown in fig. , points A, B, and C are at potentials of 70 V, 0, and 10V, respectively.
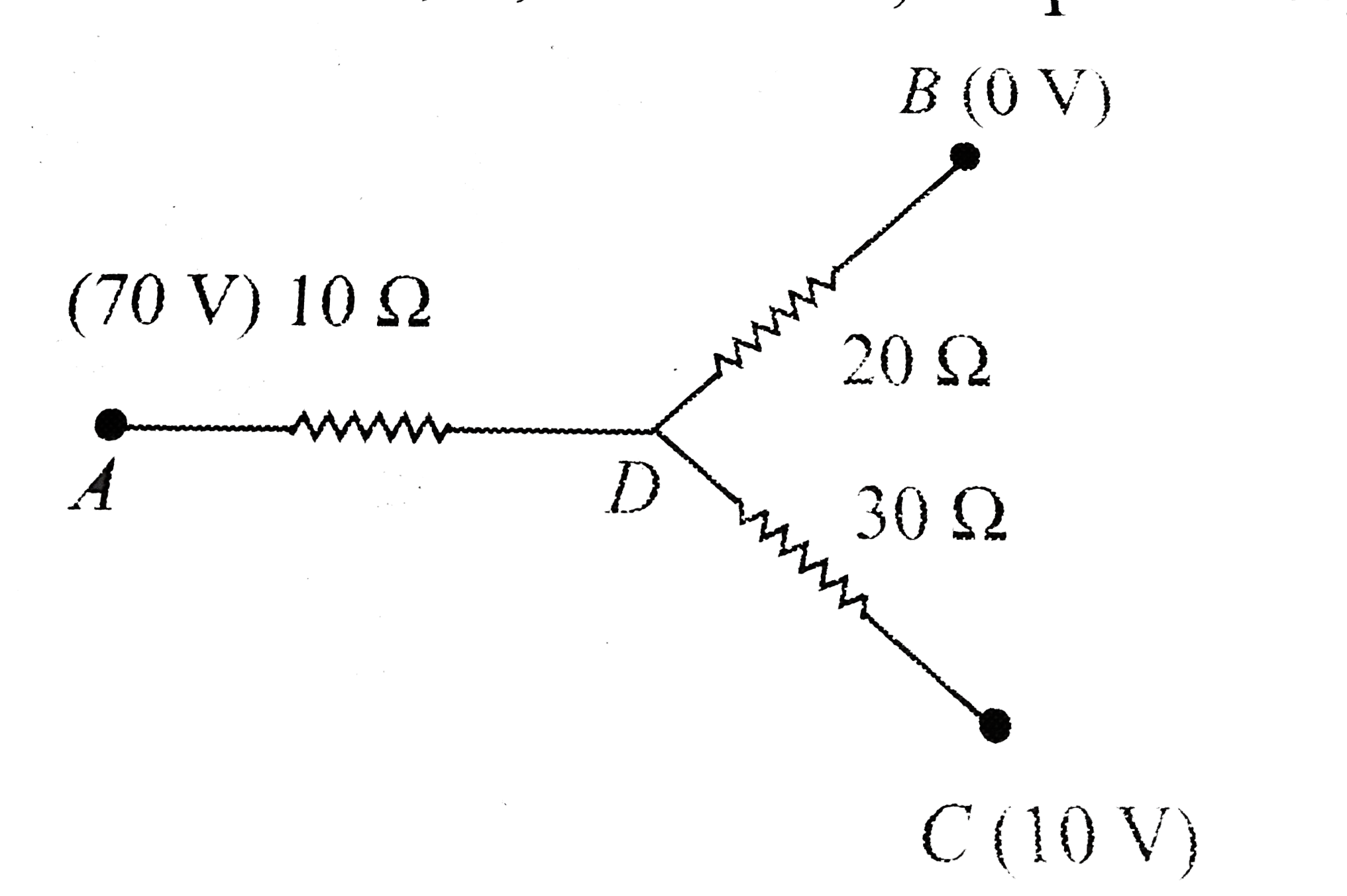
The point D will be at a potential of 60V
The point D will be at a potential of 20V
Current in the paths AD,DB and DC are in the ratio 1:2:3
Current in the paths AD,DB and DC are in the ratio 3:2:1
The correct Answer is:D
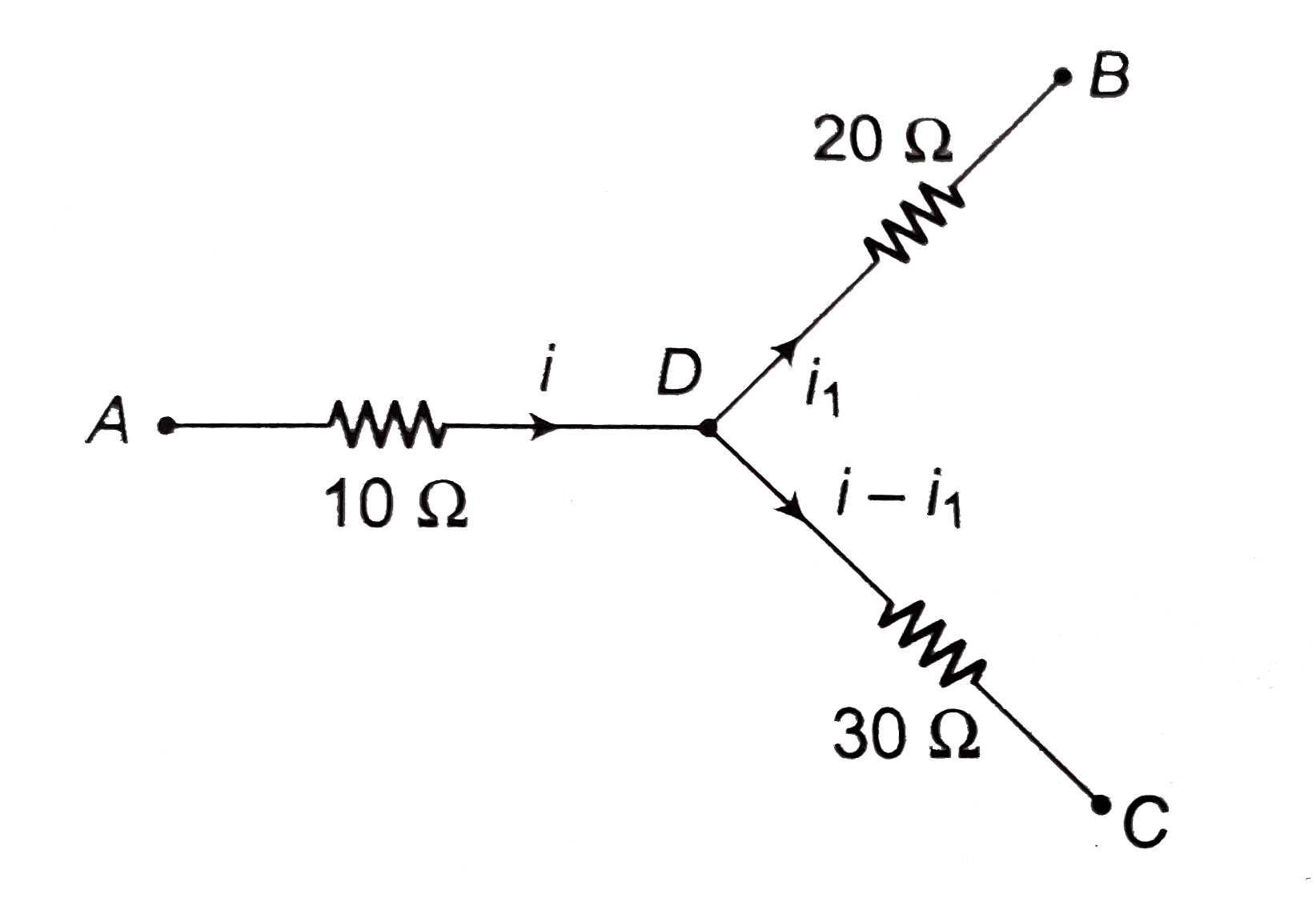
VA−VD=i×10⇒70−VD=10i …(i)
VD−VB=i1×20⇒VD−0=20i1 …(ii)
VD−VC=(i−i1)×30⇒VD−10=30(i−i1)…(iii)
From (i) and (ii)
70−20i1=10i⇒i+2i1=7....(a)
From (ii) and (iii)
20i1−10=30(i−i1)⇒3i−5i1=−1 ...(b)
Solving (a) and (b)
i1=2A,i=3A,i−i1=1A
VD=20i1=40V
i:i1:(i−i1)=3:2:1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
For the network shown in figure, points A, B and C are at potentials of 70 V, zero and 10 V respectively
 Apoint D is at a potential of 40 VBthe currents in the sections AD, DB and DC are in the ratio 4:3:2Cthe current in the sections AD, DB and DC are in the ratio 1:2:3Dthe network draws a total power of 100 W
Apoint D is at a potential of 40 VBthe currents in the sections AD, DB and DC are in the ratio 4:3:2Cthe current in the sections AD, DB and DC are in the ratio 1:2:3Dthe network draws a total power of 100 W - Question 2 - Select One
In the network shown in fig. , the potential differene across A and B is
 A6VBzeroC2VD4V
A6VBzeroC2VD4V - Question 3 - Select One
A hollow conducting sphere is placed in an electric field produced by a point charge placed at P as shown in figure.
Let VA,VB,VC be the potentials at points A,BandC respectively. Then
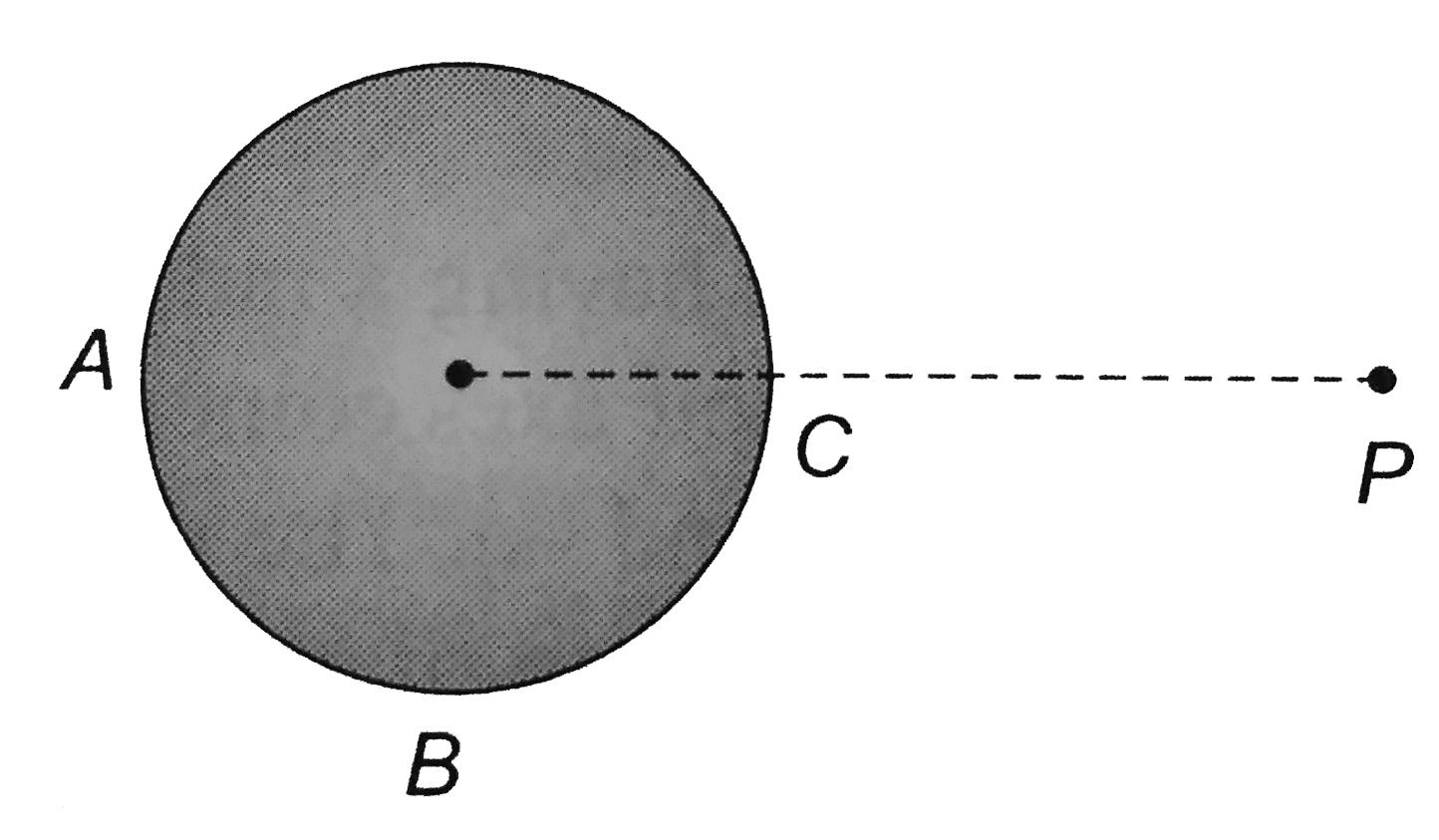 AVC>VBBVB>VCCVA>VBDVA=VC
AVC>VBBVB>VCCVA>VBDVA=VC
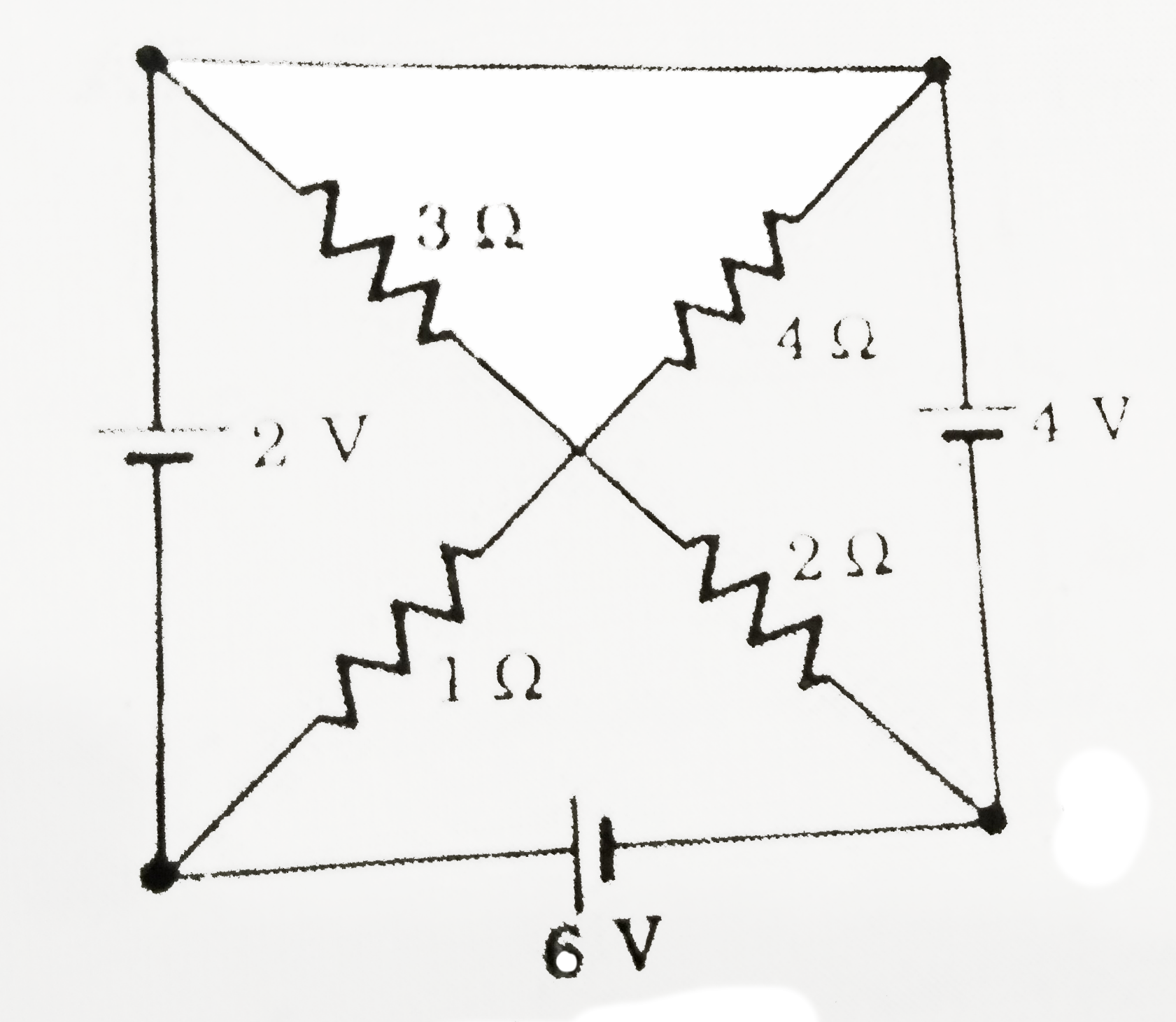
In the network shown in figure. (i) calculate the current of the 6V battery and (ii) determine the potential difference between the points A and B.View SolutionThree uncharged capacitors of capacities C1,C2 and C3 are connected as shown in the figure to one another and the potentials V1,V2 and V3 respectively. Then the potential at O will be
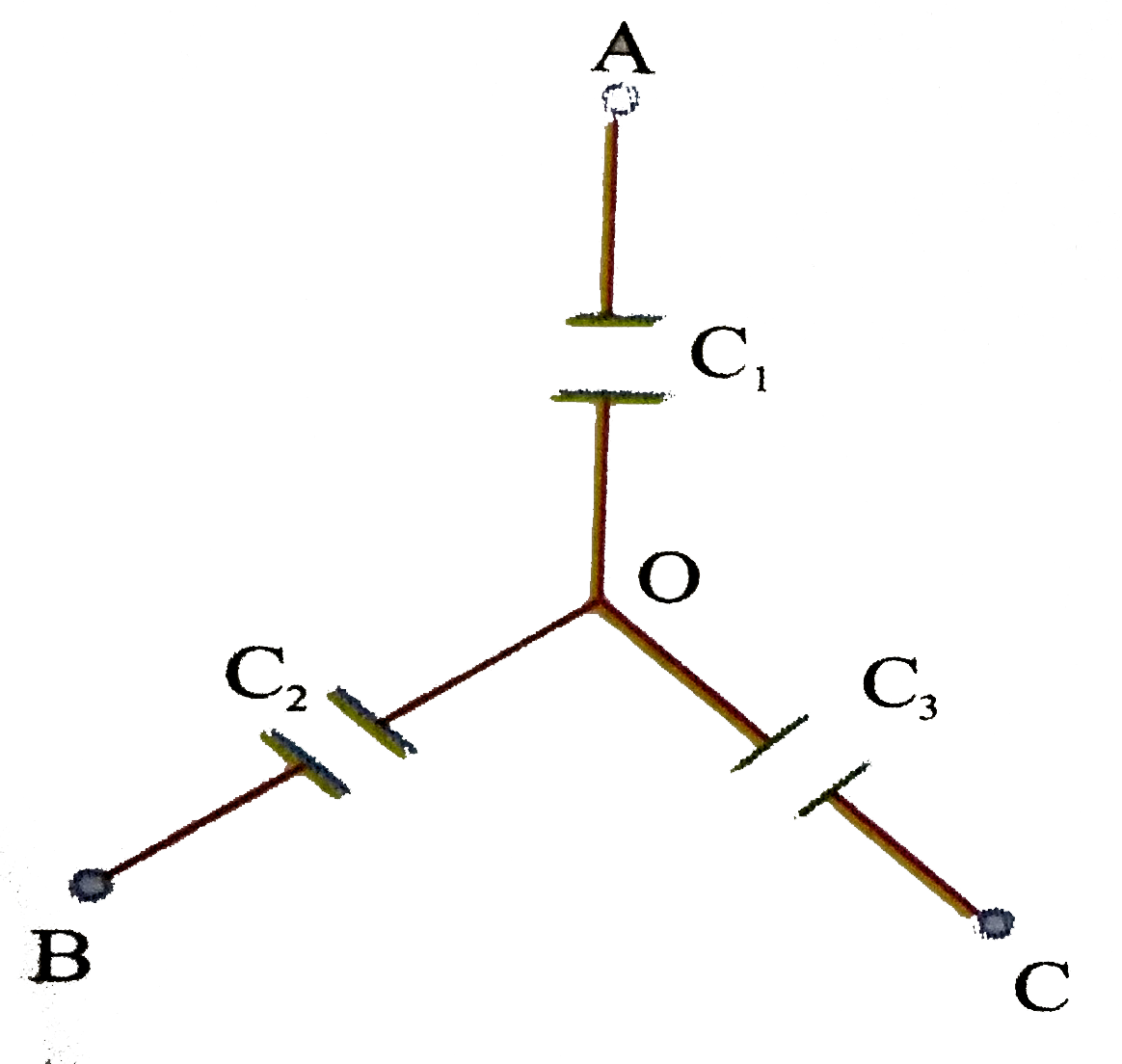 .View Solution
.View SolutionThree resistance are joined together to form a letter Y, as shown in figure. If the potentials of the terminals A,B and C are 6V,3V and 2V respectively, then the potential of the point O will be
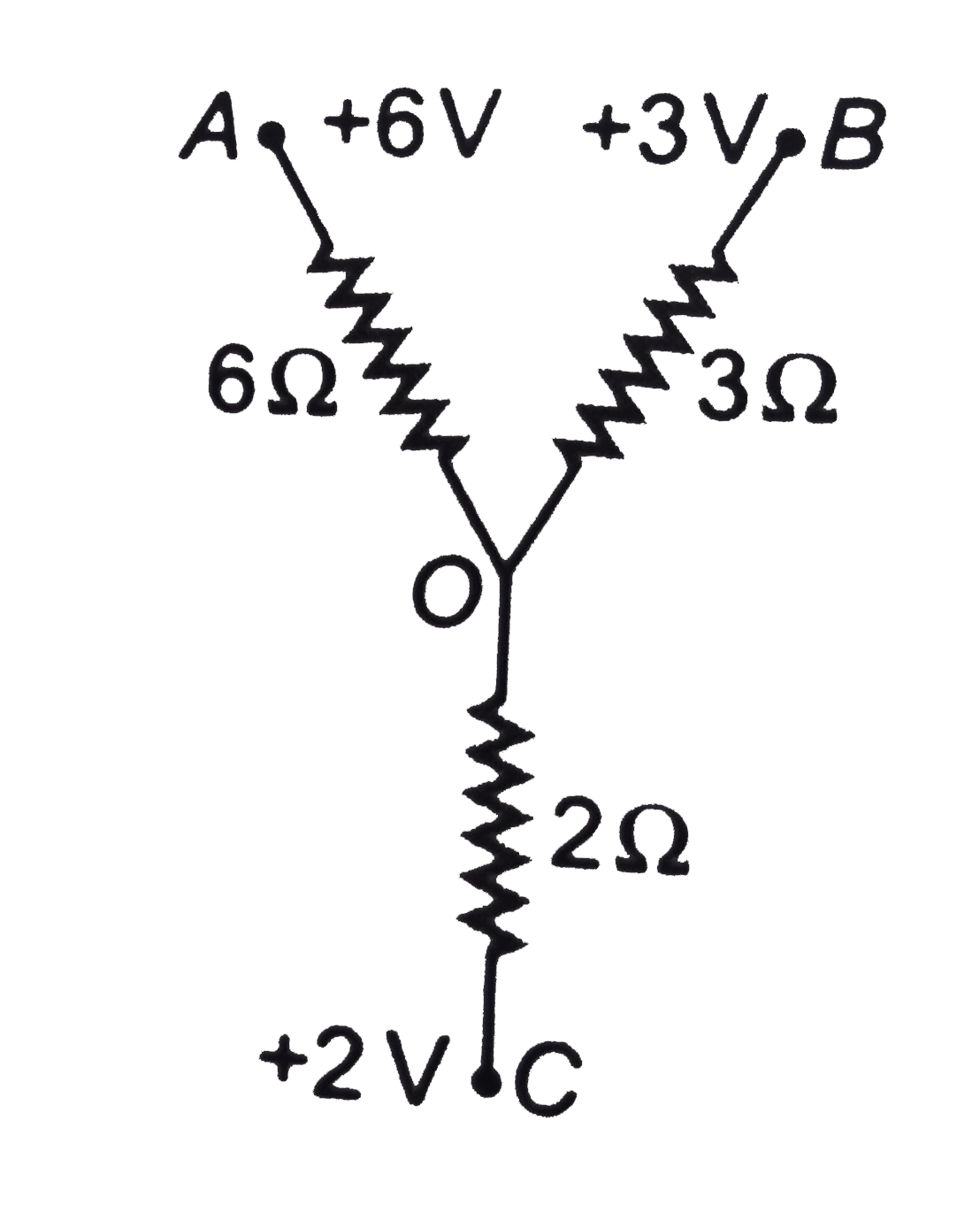 View Solution
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionFour identical capacitors are connected in series with a 10V battery as shown in the figure. The point N is earthed. The potentials of points A and B are
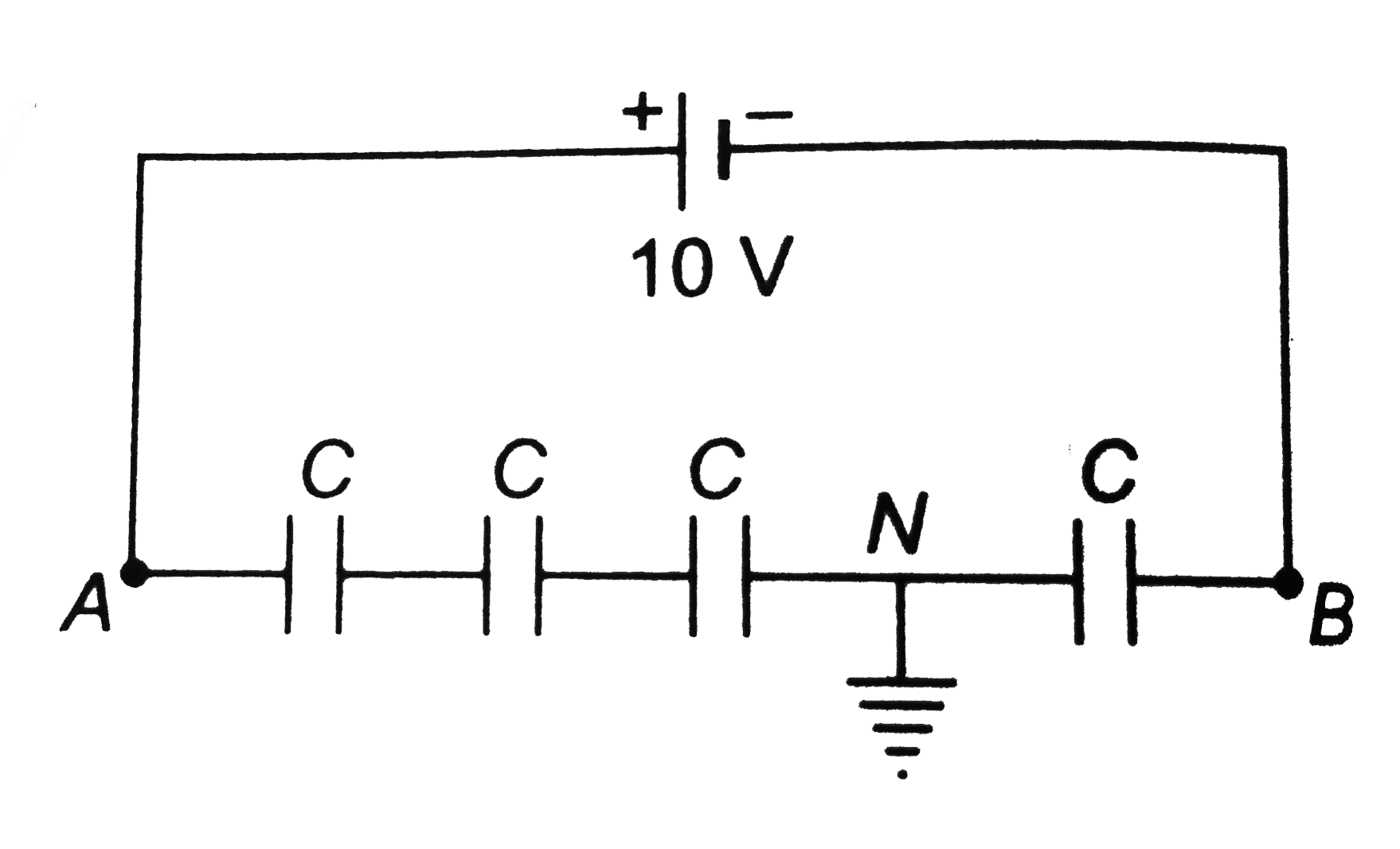 View Solution
View Solution


 .
.