In the previous problem if t is the time period of rotation
(i) t=2π√Lg
(ii) t=2π√Lcosθg
(iii) T=4π2mLt2
(iv) The ball is in equilibrium
A
(i),(ii)
B
(ii),(iii)
C
(i),(iv)
D
(ii),(iv)
Video Solution
Text Solution
The correct Answer is:B
Tcosθ=mg⇒T=mg/cosθ
Tsinθ=mω2r=mω2(Lsinθ)
T=mω2L
mgcosθ=mω2Limpliesω=√gLcosθ
Time period t=2πω=2π√Lcosθg
T=mω2L=m(4π2t2)L=4π2mLt2 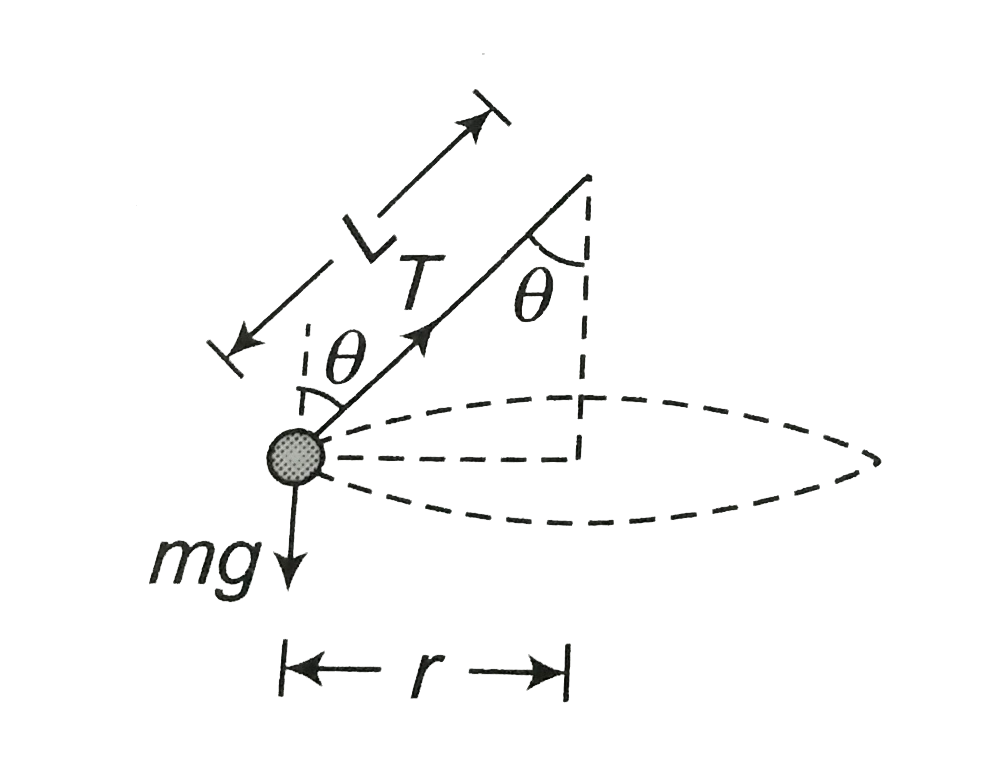
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The number of variables in the formula T=2π√lg is _____
A4B5C3D2 - Question 2 - Select One
The equation T=2π√l/g is valid only when
Athe length of the pendulum is less than the radius the earthBthe length of the pendulum is greater than or equal to radius by the earthCthe length of pendulum is independent of the radius of the earthDlength of the pendulum is equal to radius and the earth. - Question 3 - Select One
A simple pendulum of length l has a bob of mass m , with a charge q on it. A vertical sheet of charge , with the vertical . Its time period of oscillation is T in this position
(i) tanθ=σq2ε0mg
(ii) tanθ=σqε0mg
(iii) T<2π√lg
(iv) T>2π(lg)A(i),(ii)B(i),(iii)C(ii),(iii)D(ii),(iv)
The acceleration due to gravity on the surface of earth is 9.8ms−2.Time period of a simple pendulum on earth and moon are 3.5 second and 8.4 second respectively. Find the acceleration due to gravity on the moon .
Hint :Te=2π√LgeTm=2π√Lgm
T2eT2m=gmge
gm=T2eT2mgeView SolutionThe time period of an oscillating body is given by T=2π√madg. What is the force equation for this body?
View SolutionShow that the expression of the time period T of a simple pendulum of length l given by T=2π√lg is dimensionally currect
View SolutionFind the rms value of current i=Imsinωt from (i)t=0tot=πω (ii) t=π2ωtot=3π2ω
View SolutionThe number of variables in the formula T=2π√lg is _____
View Solution

