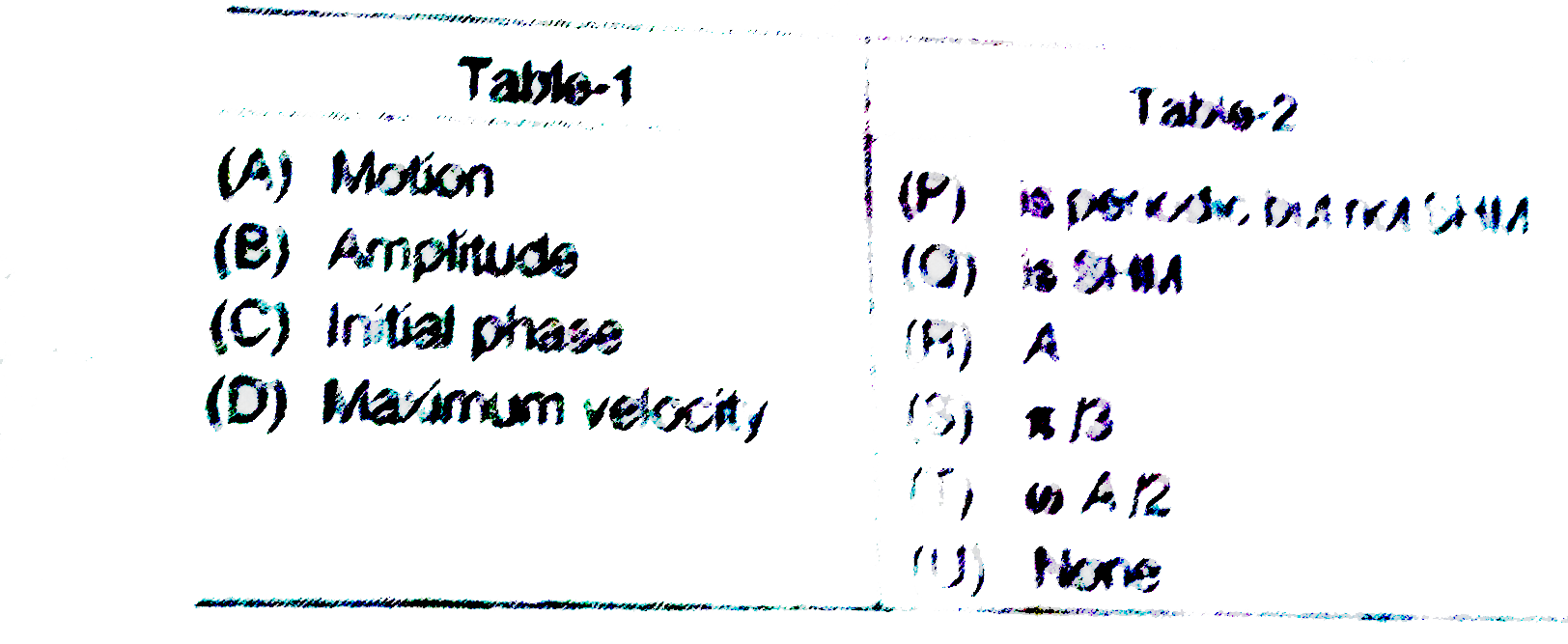In the relation dydt=2ωsin(ωt+ϕ0), the dimensional formula for ωt+ϕ0 is
MLT
MLT0
ML0T0
M0L0T0
The correct Answer is:D
To find the dimensional formula for the expression ωt+ϕ0 in the relation
dydt=2ωsin(ωt+ϕ0),
we need to analyze the components of this expression step by step.
Step 1: Understand the components of the expression
The expression ωt+ϕ0 consists of two parts: ωt and ϕ0.
Step 2: Analyze ωt
- Here, ω represents angular frequency, which is defined as the rate of change of the angle with respect to time. The dimensional formula for angular frequency ω is given by:
\omega = \frac{2\pi}{T} \quad \text{(where T is the time period)}
Thus, the dimensional formula for ω is:
(ω)=(T−1)
- The variable t represents time, and its dimensional formula is:
(t)=(T)
- Now, when we multiply ω and t:
(ωt)=(T−1)⋅(T)=(T0)=(1)(dimensionless)
Step 3: Analyze ϕ0
- The term ϕ0 represents a phase constant, which is also a measure of angle. Angles are typically measured in radians or degrees, both of which are dimensionless quantities.
Thus, the dimensional formula for ϕ0 is:
(ϕ0)=(1)(dimensionless)
Step 4: Combine the components
Now we can combine the two parts of the expression:
ωt+ϕ0
Since both ωt and ϕ0 are dimensionless, their sum is also dimensionless:
(ωt+ϕ0)=(1)+(1)=(1)(dimensionless)
Conclusion
The dimensional formula for ωt+ϕ0 is:
(M0L0T0)or simply (1)
Final Answer
The dimensional formula for ωt+ϕ0 is M0L0T0 (dimensionless).
---
\omega = \frac{2\pi}{T} \quad \text{(where T is the time period)}
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Multiple Correct
Explore 2 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Linked Comprehension
Explore 3 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Subjective
Explore 31 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
Knowledge Check
Equations of two progressive wave are given by y1=asin(ωt+ϕ1) and y2=asin(ωt+ϕ2). IF amplitude and time period of resultant wave is same as that of both the waves, then (ϕ1−ϕ2) is
Aπ3B2π3Cπ6Dπ4
The displacement of a progressive wave is represented by y=Asin(ωt−kx), where x is distance and t is time. Write the dimensional formula of (i) ω and (ii) k.
View SolutionFind the displacement equation of the simple harmonic motion obtained by combining the motion.
x1=2sinωt, x2=4sin(ωt+π6) and x3=6sin(ωt+π3)
View SolutionTwo particle AandB execute simple harmonic motion according to the equation y1=3sinωt and y2=4sin[ωt+(π/2)]+3sinωt. Find the phase difference between them.
View SolutionPlot the corresponding reference circle for given SHM, indicate the initial (t=0) position of the paritcle, the radius of the circle , and the angular speed of the rotating paritcle. Consider anticlockwise direction for rotation. x=−3sin(2t+π4) (Express in the form , x= Acos ( omega t + phi)).
View SolutionIn the expression y=asin(ωt+θ), y is the displacement and t is the time . Write the dimension ofa,ωand θ .
View Solutionif y=Asin(ωt−kx), then the value of dydx is
View SolutionFour sound sources produce the following four waves
(i) y1=asin(ωt+ϕ1)
(ii) y2=asin2ωt
(iii) y3=a'sin(ωt+ϕ2)
(iv) y4=a'sin(3ωt+ϕ)
Superposition of which two waves gives rise to interference?
View Solution