Is the force acting between two point charges kept at some distance in air, attractive or repulsive when:
To determine whether the force acting between two point charges q1 and q2 is attractive or repulsive based on their product, we can analyze the two given conditions step by step.
1. Understanding the Charges:
- We have two point charges, q1 and q2.
- The nature of the force between them depends on whether the charges are like (both positive or both negative) or unlike (one positive and one negative).
2. Condition (i): q1q2>0:
- If the product q1q2 is greater than zero, it indicates that both charges have the same sign.
- This can happen in two scenarios:
- Both q1 and q2 are positive (e.g., q1>0 and q2>0).
- Both q1 and q2 are negative (e.g., q1<0 and q2<0).
- In either case, the force between them will be repulsive because like charges repel each other.
3. Condition (ii): q1q2<0:
- If the product q1q2 is less than zero, it indicates that the charges have opposite signs.
- This can occur in two scenarios:
- q1 is positive and q2 is negative (e.g., q1>0 and q2<0).
- q1 is negative and q2 is positive (e.g., q1<0 and q2>0).
- In this case, the force between them will be attractive because unlike charges attract each other.
Conclusion:
- For condition (i) q1q2>0: The force is repulsive.
- For condition (ii) q1q2<0: The force is attractive.
- We have two point charges, q1 and q2.
- The nature of the force between them depends on whether the charges are like (both positive or both negative) or unlike (one positive and one negative).
- If the product q1q2 is greater than zero, it indicates that both charges have the same sign.
- This can happen in two scenarios:
- Both q1 and q2 are positive (e.g., q1>0 and q2>0).
- Both q1 and q2 are negative (e.g., q1<0 and q2<0).
- In either case, the force between them will be repulsive because like charges repel each other.
- If the product q1q2 is less than zero, it indicates that the charges have opposite signs.
- This can occur in two scenarios:
- q1 is positive and q2 is negative (e.g., q1>0 and q2<0).
- q1 is negative and q2 is positive (e.g., q1<0 and q2>0).
- In this case, the force between them will be attractive because unlike charges attract each other.
- For condition (i) q1q2>0: The force is repulsive.
- For condition (ii) q1q2<0: The force is attractive.
Similar Questions
Electric force between two point charges q1 and q2 at rest is F. Now is a charge −q1 is placed next to q1. What will be the (a) force on q2 due to q1(b) total force on q2 ?
View SolutionWrite the vector form of force acting between two charges q1andq2 having →r1and→r2 as their position vectors respectively.
View Solution
Knowledge Check
Magnitude of force between two point charges q1 and q2 which are separated by a distance r is given by
AF=k|q1q2|rBF=k|q1q2|rr2CF=k(|q1q2|r)2DF=k|q1q2|r2The electrostatic force between two point charges q1 and q2 at separation r is given by F=k.q1q2r2. The constant k
ADepends upon system of units onlyBDepends upon medium between the chargesCDepends on both 1 and 2DIs independent of both 1 and 2Force acting on two charges is given by F=kq1q2r2 Here k depends in :
AStrength chargeBMediumCDistance between the chargesDBoth (a) and (b)
Find out potential energy of the two point charge system having charges q1 and q2 separated by distance r.
View SolutionTwo point charges q1, and q2 are kept at a distance of r12 in air. Deduce the expression for the electrostatic potential energy of this system.
View SolutionWhen two charges q1andq2 are kept at some distance apart, force acting between these charges is F. If a third change q3 is placed quite close to q3 is placed quite close to q2 what will happen to the force between q1andq2 ?
View SolutionTwo points charges q1andq2 are placed close to each other. What is the nature of force between the charges when q1<0,q2>0,q1<0,q2<0
View SolutionA few electric field lines for a system of two charges Q1 and Q2 fixed at two different points on the x-axis are shown in the figure. These lines suggest that
(i) |Q1|>|Q2|
(ii) |Q1|<|Q2|
(iii) At a finite distance to the left of Q1 the electric field is zero
(iv) At a finite distance to the right of Q2 the electric field is zero
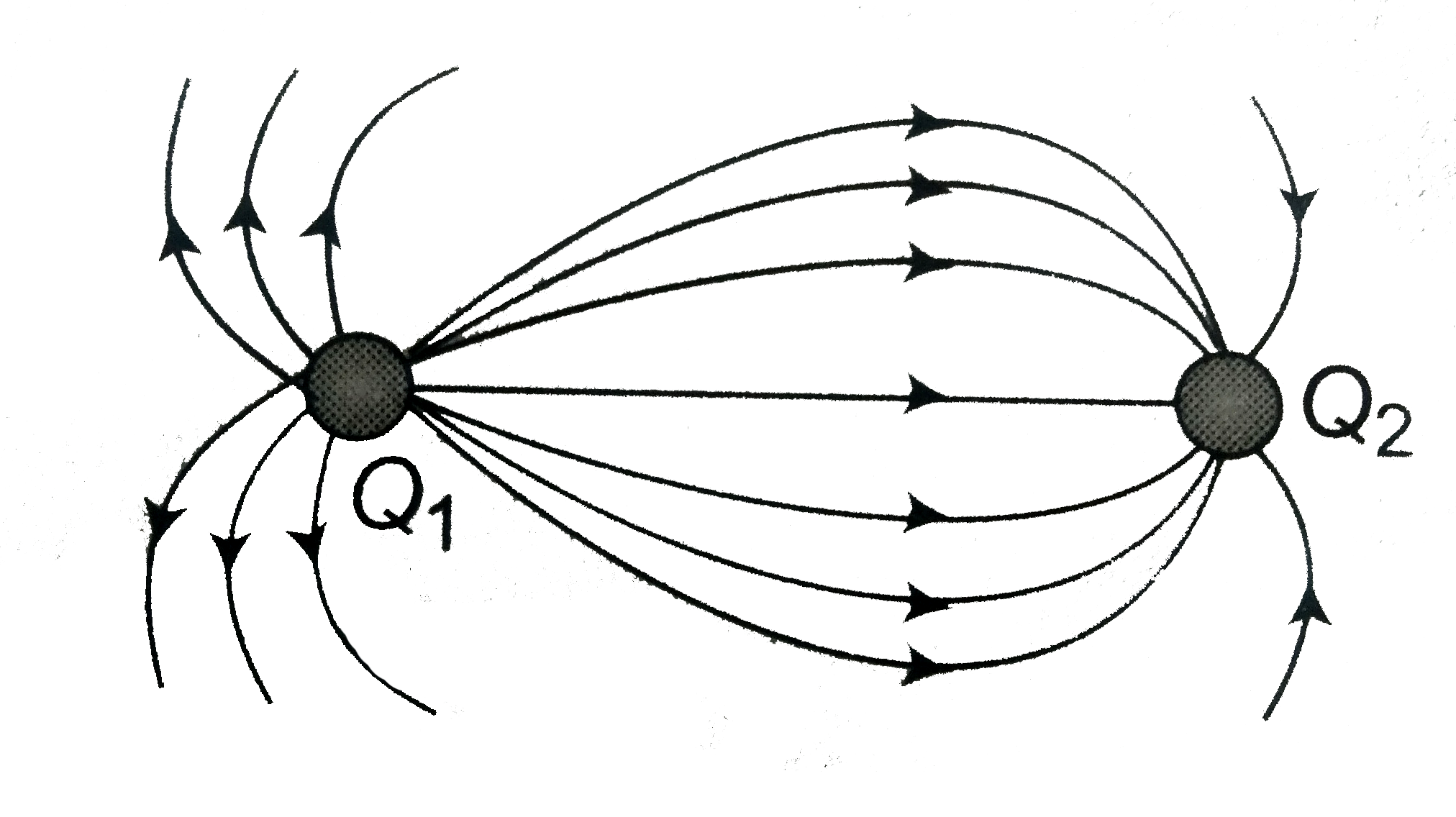
View Solution
