L is a lens such that a parallel beam of light I ncident on it, after refraction, converges to a point at a distance x from it and M is a mirror such that a parallel lbeam of light incident on it, after reflection, converges to a point at a distance y from it. Now the lens L and mirror M are placed at a distan $2 (x + y)$ from each other. An object of size 2 cm is kept infront of lens at a distance 2x from it. Find the nature, position , and size of the image that willl be seen by an observer looking toward the mirror through the lens.
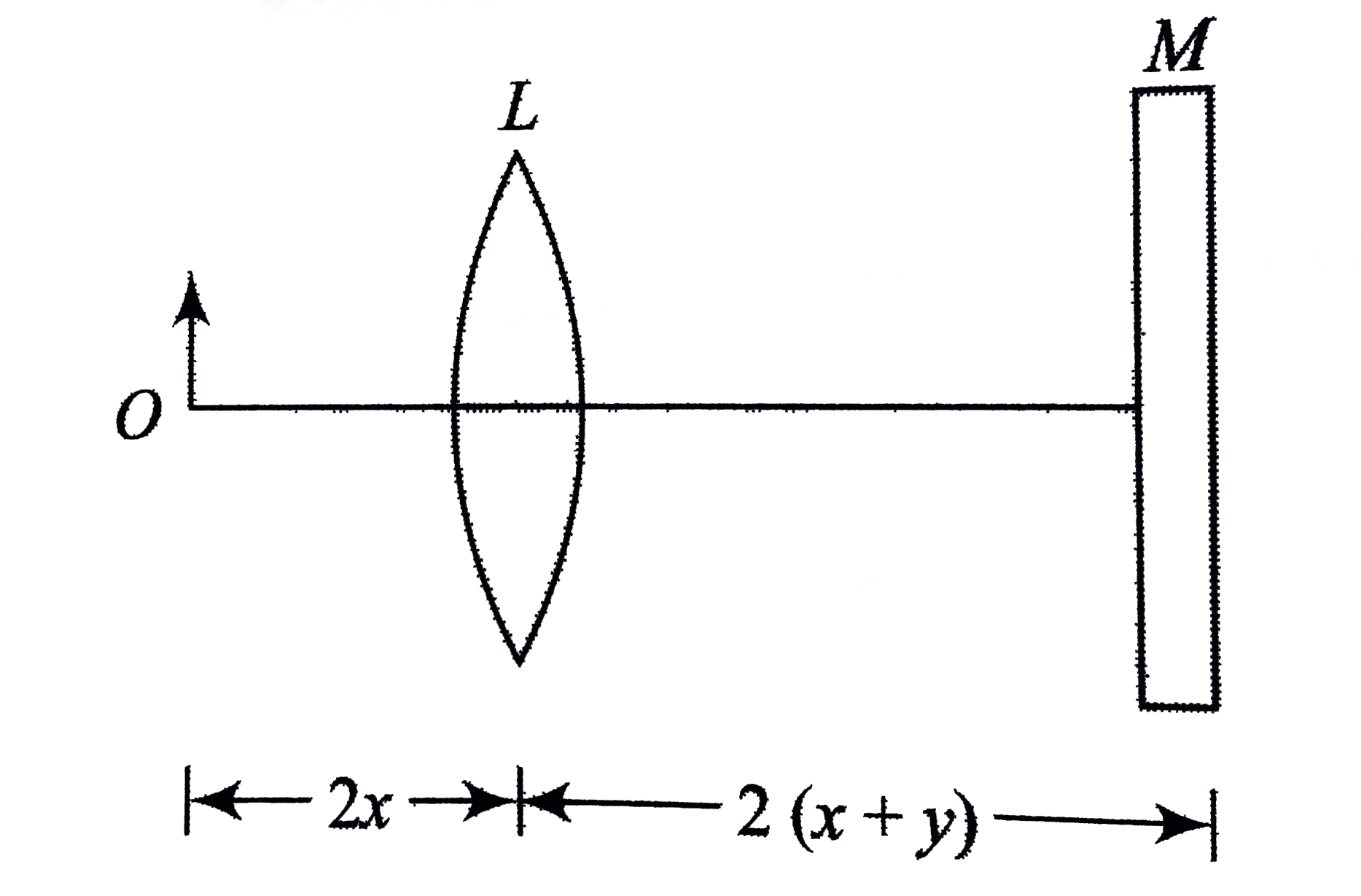
$L is a lens such that a parallel beam of light I ncident on it, after refraction, converges to a point at a distance x from it and M is a mirror such that a parallel lbeam of light incident on it, after reflection, converges to a point at a distance y from it. Now the lens L and mirror M are placed at a distan 2(x+y) from each other. An object of size 2 cm is kept infront of lens at a distance 2x from it. Find the nature, position , and size of the image that willl be seen by an observer looking toward the mirror through the lens.$

Video Solution

Text Solution

Verified by Experts

It is given that a parallel beam incident on the lens, after refraction, converges to a point at a distance x from the lens. This implies that the focal length of the lens is x.
It is also given that a parallel beam incident on the mirror, after reflectino, converges to a point at a distance y from it. This implies that the mirror is a concave mirror of focal length y.
Obviously, object O is kept at 2F of lens L. Hence, its image $I_{1}$ forms on the other side oat 2F , i.e., at distance 2x to the right of the lens. The image will be real, inverted, and magnification of this image $m_{1} = \frac{v}{u} = \frac{2 x}{- 2 x} = - 1$ .
Image $I_{1}$ of O formed by lens L acts as object for the mirror. Its distance from the pole of mirror will $2 (x + y) - 2 x = 2 y$ . This implies that it will be at the center of curvature of the concave mirror (y being the focal length of the mirror). Therefore, the concave mirror forms a real, inverted (relative to $I_{1}$ ) image $I_{2}$ at the same position.
Magnification of $I_{2}$ (relative to $I_{1}$ ), $m_{2} = - 1$ . Finally, $I_{2}$ acts as object for the lens. Its distance from thelens is 2x. Hence, it is at 2F of the lens (x being the focal length of the lens). Therefore, the lens will form a real and inverted image of $I_{2}$ at 2F on the other side, i.e., to its left. Obviously, $I_{3}$ will be at the same position as of the object.
Magnification of $I_{3}$ (relative oto $I_{2}) = - 1$
Total magnification $= m_{1} \times m_{2} \times m_{3} = - 1 \times - 1 \times - 1 = - 1$
Thus, the final image is real and inverted and of the same size (2cm).
Complete reay diagram is shown in Figure.

Updated on:21/07/2023