Light of wavelength 2000 Å can just eject electrons from a metal surface. Calculate the work function of the metal. Solution in Kannada
Given, λ=2000 Å =2×107 m, w = ?
We know that, w=hv0=hcλ
w=6.625×10−34×3×1082×10−7=9.937×10−19 J=9.937×10−191.6×10−19=6.21 eV.
Topper's Solved these Questions
- BOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - FIVE MARK QUESTIONS WITH ANSWERS5videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - SUBHASH PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NUMERICAL PROBLEMS AND ANSWERS29videos
CURRENT ELECTRICITY
- BOOK - SUBHASH PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - NUMERICALS WITH SOLUTIONS21videos
ELECTRIC CHARGES AND FIELDS
Similar Questions
Write any three experimental observations of photoelectric effect
03:01View SolutionIn the given circuit, calculate the effective resistance between A and B.
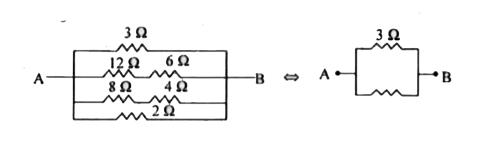 04:16View Solution
04:16View SolutionTwo point charges −2nC and +8nC are separated in air by a distance of 0.10m.Find the position of the neutral point along the line joining the 2 point charges.
04:32View SolutionThe work function of metal is 1eV. Light of wavelength 3000Å is incident on this metal surface . The velocity of emitted photo - electrons will be
01:04View SolutionThe work function of a metal is 3.45 eV. Calculate what should be the maximum wavelength of a photon that can eject photoelectrons from the metal ?
01:45View SolutionThe work function for a metal surface is 2.0 eV. Determine the longest wavelength that will eject photoelectrons from the metal surface.
02:02View SolutionWork function of a metal surface is phi=1.5eV . If a light of wavelength 5000Å falls on it then the maximum K.E. of ejected electron will be-
View SolutionLight of wavelength 2000 Å falls on a metallic surface whose work function is 4.21 eV. Calculate the stopping potential
04:13View SolutionThe work function of a metal surface is 4.2 eV. The maximum wavelength which can eject electrons from this metal surface is
01:59View SolutionElectrons are ejected from a metallic surface when light with a wavelength of 6250 Å is used. If light of wavelength 5250 Å is used instead,
01:51View SolutionLight of wavelength 3000 Å falls on a metal surface having work function 2.3 eV. Calculate the maximum velocity of ejected electrons.
(Planck's constant , h=6.63×10−34 J.s. , velocity of light c=3×108m/s , mass of an electron = 9.1×10−31 kg )04:54View SolutionShow that the two waves interfere constructively when the path difference between them is an integral multiple of wavelength Light of wavelenght 2000 A can just eject electron from a metal surface calculate the work function of the metal
01:38View SolutionThe threshold wavelength for a certain metal is 2000 Å. The work function of the is
02:06View SolutionAll electrons ejected from a metal surface by the incident light of wavelength 200 nm can be stopped before travelling 1 m in the direction of uniform electric field of 4NC^(-1) . The work function of the metal surface is
01:47View Solutionएक धातु सतह के लिये फोटो इलेक्ट्रॉनिक तरंगदैर्ध्य 6600Å है। इस धातु का कार्य फलन है
03:18View Solution

